Integers and their Opposites
Basics on the topic Integers and their Opposites
Integers and Their Opposites
Understanding integers and their opposites is like having a superpower in math! Imagine being able to represent any whole number, positive or negative, and knowing its exact opposite. From calculating temperatures to understanding bank account balances, knowing about integers and their opposites helps us make sense of the world around us. Once you understand how integers and their opposites work, you can easily learn subtracting integers and adding integers
Integers are whole numbers that can be positive, negative, or zero. They are used to represent both quantities and positions on the number line.
Understanding Integers and Their Opposites – Explanation
An integer is a number without any fractional or decimal part. It can be positive, negative, or zero. Integers are often used to represent situations involving counting, direction, temperature, and more.
What is the opposite of an integer?
The opposite of an integer is the number that, when added to it, gives zero. For example, the opposite of 5 is -5, and the opposite of -3 is 3.
| Integer | Opposite |
|---|---|
| 0 | 0 |
| 5 | -5 |
| -3 | 3 |
| 10 | -10 |
| -7 | 7 |
| 2 | -2 |
Integers and Their Opposites – Examples
Let’s practice understanding integers and their opposites:
What is the opposite of -18?
- The opposite of -18 is 18.
Integers and Their Opposites – Summary
Key Learnings from this Text:
- Integers are whole numbers that include positive, negative, and zero values.
- The opposite of an integer is the number that, when added to it, results in zero.
- Understanding integers and their opposites is essential for various mathematical operations and real-life applications.
Integers and Their Opposites – Frequently Asked Questions
Transcript Integers and their Opposites
"Hey Kai, what's under that cover?" "That button is very tempting!" "Go on Kai, you should totally push it." "Oh, that goes without saying, June!" Welcome to the Integer Universe! To make it back to your universe, you will need to win the game by using integers and their opposites. Buckle up and pay close attention to the tutorial! An integer is any whole number. The opposite of an integer is a number that is the same distance from a set point on a number line. This is called equidistant. Take a look at this number line. Going left from zero are the negative integers, and going right from zero are the positive integers. Zero is a special case here, since it is neither positive or negative, which makes zero it's own opposite! To find the opposite of an integer, you always move in the opposite direction from zero the same number of values. If we move two places to the right from zero we end up on two. To find the opposite, we must move two places to the left from zero to end up on negative two. In this case, the opposite of two is negative two! Now, you probably noticed we counted the same value from zero both ways, two. This is what is known as the absolute value. The absolute value of two and negative two is two, since we moved the same distance from zero each time. Absolute value is the integer's distance from zero, whether it is a positive integer or negative integer. That concludes the tutorial, get ready to play! Level one, begin! Find the opposite integer of seven! The opposite must be equidistant, or equal distance, from zero, so we count seven values to the left of the zero, which is negative seven. The opposite of seven is negative seven. Now find the opposite integer of negative eleven! The opposite must be equidistant from zero, so we count eleven values to the right of the zero, which is eleven. The opposite of negative eleven is eleven. Level one complete! Level two, begin! Find the opposite of six, and name the absolute value! The opposite of six is negative six. Since we counted six values either direction from zero both times, the absolute value is six. Now find the opposite of negative ten, and name the absolute value! The opposite of negative ten is ten. Since we counted ten values either direction from zero, the absolute value is ten. For a bonus, in this problem, what is the opposite of the opposite? Since the opposite of negative ten is ten, the opposite of the opposite is the original value, negative ten. Phew! You won the game, but before you return, let's summarize. All integers have a positive value and a negative value, except for zero. Zero is neither positive or negative, and is it's own opposite. The opposite of any integer is equidistant from zero on a number line. The distance positive and negative integers are from zero is known as the absolute value. "Woah. I feel very negative about that experience!" "Well I'm absolutely positive that we shouldn't do that again. Let's cover it back up Kai!"
Integers and their Opposites exercise
-
Connect the terms and definitions.
Hints1, -1, 2, -2 and so on are all integers.
1.5, -1.5, 2.75, -2.75 are NOT integers.
What is the difference in these groups of numbers?
5, 6,10, and 20 are all positive integers.
-5, -6, -10, and -20 are all negative integers.The absolute value of 10 and -10 is 10.
The absolute value of 25 and -25 is 25.
The absolute value of 42 and - 42 is 42.
What is the definition of absolute value?Solution- Two or more things that are the same distance from a set point are equidistant.
- Another name for a whole number is integer.
- Whole numbers with a positive value more than zero are positive integers.
- Whole numbers with a negative value less than zero are negative integers.
- The distance from an integer to zero on a number line is the integer's absolute value.
-
Identify the true sentences.
HintsThe absolute value is the distance from an integer to zero on a number line.
Example: The absolute value of 10 and -10 is 10.Both 10 and -10 are integers.
10.5 and -12.6 are NOT.-12 and 12 are opposite integers.
They are both 12 away from zero on a number line.There are 3 correct choices and 2 false.
SolutionTRUE
- All integers have a positive or negative value except for zero.
- The opposite of any integer is equidistant from zero on a number line.
- Both positive and negative integers have the same absolute value.
- All integers have a positive value.
- Only positive integers have an absolute value.
-
Find opposite integers.
HintsThe opposite of any integer is equidistant from zero on a number line.
Integers are whole numbers.
There are 2 correct choices and 4 false.
SolutionCORRECT
These are opposite integers.- 10 and -10
- 15 and -15
- 5, -10 are not equidistant from zero.
- 20, 20 are the same number and cannot be opposites.
- -17, 7 are not equidistant from zero.
- 2.5, -2.5 are not whole numbers and therefore not integers.
-
Find the opposite integer and absolute value.
HintsThe opposite of any integer is equidistant from zero on a number line.
The absolute value is the distance from an integer to zero on a number line.
Example: The absolute value of 10 and -10 is 10.If an integer is positive, its opposite integer must be negative.
If an integer is negative, its opposite integer must be positive.Solution5
The opposite integer is -5 because this is equidistant from zero on the number line.
The absolute value is 5 because this is the distance from zero.17
The opposite integer is -17 because this is equidistant from zero on the number line.
The absolute value is 17 because this is the distance from zero.-99
The opposite integer is 99 because this is equidistant from zero on the number line.
The absolute value is 99 because this is the distance from zero.-22
The opposite integer is 22 because this is equidistant from zero on the number line.
The absolute value is 22 because this is the distance from zero. -
Find the absolute value.
HintsAbsolute value is a number's distance from zero on a number line.
Both negative and positive numbers will have the same absolute value if they are opposite.
Example: 20, and -20 have the same absolute value.SolutionThe absolute value of 5 and -5 is 5.
This is because they are both 5 away from zero on the number line. -
Determine the opposite integer and absolute value.
HintsIf an integer is positive, its opposite integer must be negative.
If an integer is negative, its opposite integer must be positive.The absolute value is the distance from an integer to zero on a number line.
Example: The absolute value of 10 and -10 is 10The opposite of any integer is equidistant from zero on a number line.
Solution43
The opposite integer is - 43 because this is equidistant from zero on the number line.
The absolute value is 43 because this is the distance from zero.- 5
The opposite integer is 5 because this is equidistant from zero on the number line.
The absolute value is 5 because because this is the distance from zero.38
The opposite integer is - 38 because this is equidistant from zero on the number line.
The absolute value is 38 because this is the distance from zero.- 47
The opposite integer is 47 because this is equidistant from zero on the number line.
The absolute value is 47 because this is the distance from zero.

Even and odd numbers

Divisibility Rules - 3, 6, 9

Divisibility Rules - 7

Divisibility Rules - 4, 5, 8, 10

Prime Numbers
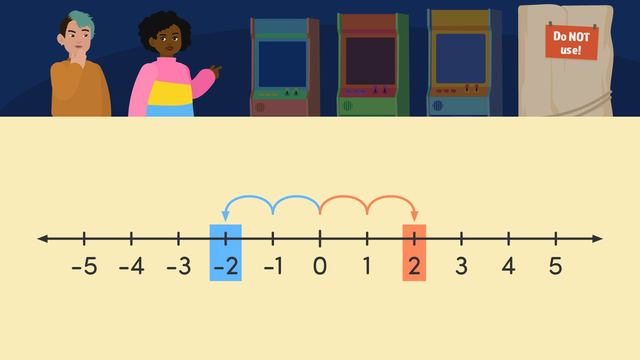
Integers and their Opposites
Least Common Multiples
Prime Factorization

Adding and Subtracting Rational Numbers on a Number Line
Ordering Rational Numbers
Cube Roots

Rational and Irrational Numbers
Ordered Pairs on the Coordinate Plane
Finding the Greatest Common Factor
Adding and Subtracting Decimals
Comparing Fractions
Equivalent Fractions
Simplifying Fractions
Temperature Conversion

Decimal Expansions
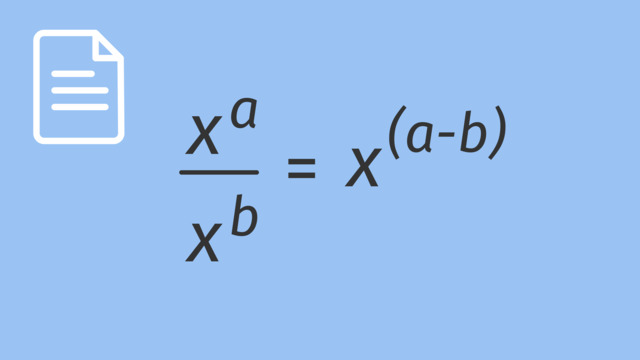
Division with Exponents

How to Convert Decimals Expansions
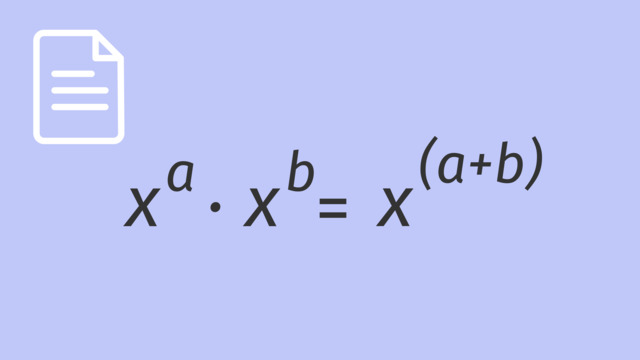
Multiplication with Exponents
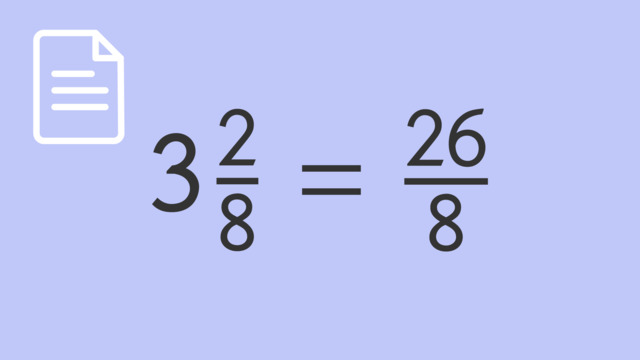
Improper Fractions and Mixed Numbers

Multiplying Mixed Numbers: Word Problems












