Equivalent Fractions

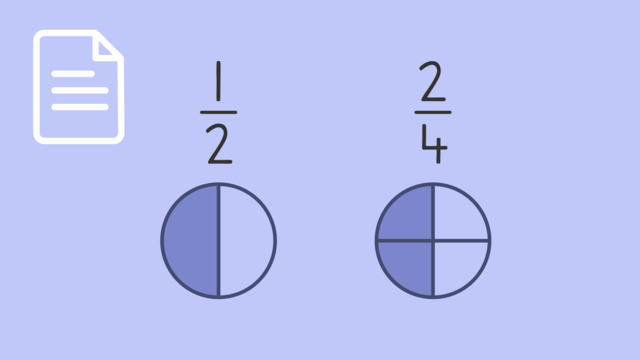
Equivalent Fractions exercise

• What is the correct notation?

  Hints

  The number on the top of the fraction indicates how many parts are taken.

  The number on the bottom of the fraction shows the number of total parts in the whole.

  For example, $\frac{1}{2}$ means $1$ part out of $2$ total parts.

  The fraction notation words are Numerator and Denominator. One of them is the number of parts and belongs at the top and one is the total parts and belongs on the bottom.

  As an example we can see the shaded parts out of the total parts.

  Solution

  $\mathbf{\frac{Numerator}{Denominator}}$

  Numerator indicates how many parts are taken.

  Denominator shows the number of total parts in the whole.

• Find equivalent fractions.

  Hints

  For equivalent fractions they should both have the same value.

  We multiply the numerator and denominator by the same number. For example,

  If we were given both denominators $\frac{1}{2}$ and $\frac{?}{6}$ we would multiply by $3$ to get from $2$ to $6$.

  Therefore, we multiply $1$ by $3$ also, to get the equivalent numerator.

  Answer is $\frac{1}{2}$ = $\frac{3}{6}$.

  Solution

  $\mathbf{\frac{1}{6}}$ = $\mathbf{\frac{3}{18}}$

  $\mathbf{\frac{2}{7}}$ = $\mathbf{\frac{6}{21}}$

  $\mathbf{\frac{4}{11}}$ = $\mathbf{\frac{28}{77}}$

  $\mathbf{\frac{6}{13}}$ = $\mathbf{\frac{24}{52}}$

  For example, If we were given both denominators $\frac{1}{6}$ and $\frac{?}{18}$ we would multiply by $3$ to get from $6$ to $18$.

  Therefore, we multiply $1$ by $3$ also, to get the equivalent numerator.

• Which ones are equivalent?

  Hints

  A fraction is equivalent when you have multiplied the numerator and denominator by the same multiplier.

  For example,

  An example of an equivalent fraction is:

  $\frac{1}{5}$ = $\frac{3}{15}$ (we have multiplied by $3$)

  There are $3$ correct answers here!

  Solution

  Correct answers are:

  • $\mathbf{\frac{6}{9}}$ ($\frac{2}{3}$ multiplied by $3$)

  • $\mathbf{\frac{14}{21}}$ ($\frac{2}{3}$ multiplied by $7$)

  • $\mathbf{\frac{10}{15}}$ ($\frac{2}{3}$ multiplied by $5$)

  The ones that do not fit are $\frac{5}{7}$, $\frac{10}{12}$ and $\frac{4}{5}$ because, there is no one multiplier which will make them equivalent to $\frac{2}{3}$.

• Find the equivalent numbers of red apples.

  Hints

  The fraction of red apples is $\frac{9}{12}$.

  We are looking for equivalent fractions to this.

  Equivalent is when we multiply the numerator and denominator by the same number.

  Example of an equivalent fraction to $\frac{9}{12}$.

  Multiply the numerator and denominator by $10$.

  9$\times$10 = $90$

  12$\times$10 = $120$

  An equivalent fraction is $\frac{90}{120}$.

  There are $2$ correct answers.

  The fraction could be smaller also!

  For example, $\frac{6}{8}$ can be divided by $2$

  This gives $\frac{3}{4}$, which is equivalent to $\frac{9}{12}$ when multiplied by $3$.

  Solution

  $\mathbf{\frac{45}{60}}$ and $\mathbf{\frac{6}{8}}$ are correct!

  We want fractions which are equivalent to $\frac{9}{12}$.

  If we multiply by $5$ we get the answer $\frac{45}{60}$.

  If we divide by $3$ then multiply by $2$ we get the answer $\frac{6}{8}$.

• What is the equivalent fraction?

  Hints

  Equivalent fractions represent the same value.

  Therefore, we multiply or divide both the numerator and denominator by the same number to make this true.

  For example:

  What do we need to multiply $4$ by to get to $20$?

  We then multiply the numerator by this number too.

  $20\div4 = 5$.

  Therefore, we multiply the numerator by $5$.

  $3\times5 = 15$

  Solution

  15

  We notice that the denominators are $4$ and $20$.

  We multiply $4$ by $5$ to get to $20$.

  Therefore, we must multiply the numerator by $5$ also.

  $3 \times 5 = 15$

• Evaluate expressions to find equivalent fractions.

  Hints

  When n = $3$.

  n + $1$ = $3$ + $1$ = $4$

  Replace n by $3$ into each fraction to find its equivalent fraction.

  When we have substituted n = $3$ into each fraction we look to find its equivalent.

  For example, if we got $\frac{3}{n+5}$ we substitute n = $3$ into it.

  n = $3$ and n + $5$ = $3$ + $5$ = $8$.

  The equivalent fraction would be $\frac{3}{8}$

  Solution

  Solution is illustrated above.