Adding Integers

Basics on the topic Adding Integers
Have you ever wondered how to add a negative number to a positive number? Negative and positive numbers, including zero, are called integers. Addition of integers is not that hard, once you understood the main concept behind it. The number line can help you understand how adding integers works.
There is a special case in the addition of positive and negative numbers: adding opposite numbers. Opposite numbers are numbers that have the same distance from zero, also called absolute value. If you look at a number line, -3 and 3, for example, have the same distance from zero. Opposite numbers are always numbers that look similar, apart from the positive or negative sign.
The sum of opposite numbers always equals zero. To show this on the number line, think of negative numbers as going to the left directionally, whereas positive numbers means going to the right directionally. If you start at zero and go 3 steps to the right for 3, going 3 steps in the opposite direction for -3 makes you end up at zero again. So always remember: if adding opposite numbers, regardless of order, you get 0 as the solution.
But what about integers that are not opposite numbers? To understand this case you should look at a number line as well. Again, for positive numbers start at zero and go the amount of steps to the right; adding a negative number means you go those number of steps to the left. Wherever you end up on the number line after doing so will be the solution.
The video shows you adding intergers the easy way, step by step and in an entertaining setting. Enjoy!
Apply and extend previous understandings of numbers to the system of rational numbers. CCSS.MATH.CONTENT.6.NS.C.5
Transcript Adding Integers
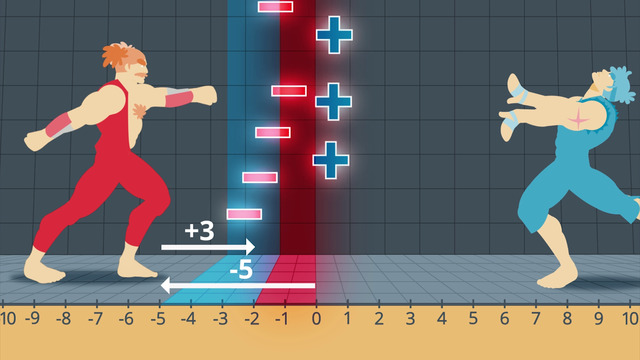
Meet Elena and Noah. Given a choice, they would rather play video games after school than do homework! Can you believe that?
They're playing a fighting game called Adding Integers. Elena is Negatron, who attacks with negative numbers and Noah is Positron, who attacks with positive numbers. Let's take a look!
Adding Opposite Numbers with the same Absolute Value
You already know integers. These are the whole numbers on the positive and negative side of the number line. They are separated by zero, just like the opponents in the game.
OK let's look at the number line to see what happens when we have to add opposite numbers with the same absolute value. Noah attacks first with a positive 3 and Elena counters with a negative 3.
You go 3 steps left for negative 3 and then 3 steps right from that point for positive 3. Adding equal and opposite numbers always equals zero. Or, if we write this as an equation: −3 + (+3) = 0.
So remember: Adding numbers with opposite signs equals zero.
Adding two Negative Numbers
Let's watch Elena and Noah play another round. This time, Elena uses Negatron's power up of negative 2. Now we have to add two negative numbers.
Negative 3 plus negative 2 means you go 3 steps left for negative 3 and then 2 more steps left for negative 2, ending up with negative 5.
Adding two negative numbers equals a negative sum.
Adding a Negative and a Positive Number
Oh no! Noah forgot to use Positron's power up! He uses the regular attack of positive 3. So now we have negative 5 and positive 3.
Again, you go 5 steps left for negative 5 and from that point 3 steps right for positive 3. Adding both arrows gives you negative 2.
We can write the equation as: −5 + (+3) = −2.
When adding a positive and a negative number, the sign of the number which has the greater absolute value "wins."
Adding Integers exercise
-
Determine the correct value of each arrow on the number line.
HintsThere are two important things to consider:
- First, focus on the length of the arrow.
- Then, take a look at the direction in which the arrow is pointing.
There are two arrows of the same length, but whose directions differ.
SolutionAs we know, the number line consists of a positive side and a negative side. The positive and negative numbers are separated by zero on the number line. We can display arrows on the number line that differ in both length (number of units) and orientation (if they are positive or negative).
When we examine the different arrows, we can see that two arrows can have the same length. The only difference is their orientation. One points to the left while the other points to the right, depending on whether the number is positive or negative. Additionally, it doesn't matter where on the number line we find the arrow.
Let's take a closer look at the arrows in the picture from top to bottom. We have three different arrows:
- The first arrow differs in its orientation from the other two. It's pointing to the right, in the positive direction, with a length of $3$ units. The correct value for this arrow is $+3$.
- The next arrow is a little shorter. Its length is $2$, but since it's pointing to the left, in the negative direction, the correct value is $-2$.
- The last arrow has a length of $3$ units. It's also pointing to the left, also in the negative direction, so the correct value is $-3$.
-
Explain why Negatron's power up decides the game.
HintsMoving left on the number line indicates a negative number.
Moving right on the number line indicates a positive number.
SolutionWhat happens when we add a positive and a negative number with the same absolute value? (Remember, absolute value is the value of a number without the sign.) To answer this question, we can imagine moving along the number line. Let's try this with $+3$ and $-3$:
- $+3$ means moving three steps to the right on the number line.
- $-3$ means moving three steps to the left on the number line.
A power up of $-2$ means moving two steps further to the left on the number line. Since we have already moved three steps left, the power up can be represented by the expression $-3 + (-2)$. In this example, power up means we have to add two numbers. Evaluating this expression gives us $-3-2=-5$. Now we can confidently say that adding two negative numbers equals a negative sum. When adding a positive and a negative number together, the sign of the number with the greater absolute value wins.
-
Assign the value of the arrows to expressions using addition of intergers.
HintsThe lower arrow represents the left number while the upper arrow represents the right number.
Take a closer look at the algebraic sign of each number.
Adding two negative numbers equals a negative sum.
SolutionThe arrows on the number line represent both a calculation and the result. Let's take a look at this example. The upper arrow goes from $-3$ to $-4$. Its length is $1$ unit and it is negative because the arrow is pointing to the left. We can see that the lower arrow goes from $0$ to $-3$. So it has a length of $3$ units. Because the arrow points to the left, it also represents a negative number. This represents the mathematical expression $-3+(-1)$ which can be simplified to $-3-1$. Evaluating this expression gives us $-4$.
These are the other expressions represented in the pictures from left to right:
- The second picture represents $-1+(-2)=-1-2=-3$.
- The third picture represents $+2+(-3)=+2-3=-1$.
- The fourth picture represents $-2+(+1)=-2+1=-1$.
-
Evaluate the expressions by adding the integers.
HintsAdding opposite numbers equals zero:
- $-1+(+1)=0$
- $+5+(-5)=0$
When adding a positive and a negative number, the sign of the number with the greater absolute value 'wins.' Absolute value is the value of a number without the sign.
SolutionWe can simplify all mathematical expressions where negative and positive numbers are added into a single number. Let's take a closer look at $+7+(-3)$. We can imagine moving seven steps to the right from $0$ and then moving three steps to the left. Doing so leaves us with $+4$, or simply $4$.
Here are the results of the other expressions from left to right:
- $-3+(-4)=-3-4=-7$
- $-2+(+2)=-2+2=0$
- $+5+(-2)=+5-2=3$
-
Find the missing numbers on the number line.
HintsYou can move to the left or to the right of a known number.
Moving left means adding a negative number, while moving right means adding a positive number.
SolutionThere are some numbers missing on our number line. But that's no problem since we can determine them by moving to the left or to the right.
There are two known numbers: $-3$ and $6$. From one of these numbers, we can move left or right to find out which number belongs in each blank. It makes sense to start with a number we already know.
From $-3$ we can go three steps to the right to reach one blank. Moving three steps to the right means adding $+3$. So that gives us $-3+(+3)=-3+3=0$. In the same way, we can move one step to the left from $6$ to reach another blank. This is represented by the expression $6+(-1)=6-1=5$. So the unknown number was $5$.
The other missing numbers were $-6$, $-2$ and $3$.
-
Determine the value of these expressions by adding the intergers.
HintsWhen adding a positive and a negative number, the sign of the number with the greater absolute value 'wins.' Absolute value is the value of a number without the sign.
Calculate from left to right.
Imagine moving along the number line. Adding a negative number means moving left, while adding a positive number means moving right.
SolutionWe had to calculate each expression before we could arrange them in order. For each, imagine walking on the number line: adding a negative number means walking left and adding a positive number means walking right. We could do that for the expression $+2+(-5)+(-1)$: starting at zero, we walk two steps to the right, reaching $2$. Then, we walk $5$ steps left getting to $-3$. Finally, we come to $-4$ after going $1$ final step to the left.
These are the other results arranged in the right order:
- $-3+(+2)+(-1)=-2$
- $+6+(-3)+(-3)=0$.
- $-7 + (+8)+(+2)=3$
In general, we can remember that adding two negative numbers equals a negative sum. When adding a positive and a negative number, the sign of the number with the greater absolute value defines the sign of the result.

Variables
Simplifying Variable Expressions
Evaluating Expressions

How to do Order of Operations?

Distributive Property

Adding Integers
Subtracting Integers

Multiplying and Dividing Integers
Types of Numbers
Transforming Terminating Decimals to Fractions and Vice Versa

Transforming Simple Repeating Decimals to Fractions and Vice Versa
Rational Numbers on the Number Line
Standard and Scientific Notation

Using Operations with Scientific Notations












i love the game music!!
wow!
Great video!