Divisibility Rules - 7


Basics on the topic Divisibility Rules - 7
Introduction to the Video Divisibility Rules 7
A number is divisible by 7 if it has a remainder of zero when divided by 7. Examples of numbers which are divisible by 7 are 28, 42, 56, 63, and 98. Divisibility by 7 can be checked by using long division, although this process can be quite time-consuming. Especially when faced with a very large number. Thus, knowledge of divisibility rules for 7 can be very helpful for determining if a number is divisible by 7 or not quickly.
Divisibility by 7 – Rules
Here are two rules which can be utilized to test divisibility by 7: Rule 1: Remove the last digit, double it, subtract it from the truncated original number and continue doing this until only one digit remains. If this is 0 or 7, then the original number is divisible by 7. For example, to test divisibility of 12264 by 7, we simply perform the following manipulations: 1226 - 8 = 1218 121 - 16 = 105 10 - 10 = 0 Thus, 12264 is divisible by 7.
Rule 2: Take the digits of the number in reverse order, that is, from right to left, multiplying them successively by the digits 1, 3, 2, 6, 4, 5, repeating with this sequence of multipliers as long as necessary. Then add the products. If the resulting sum is divisible by 7, then the original number is divisible by 7. For example, to test divisibility of 12264 by 7, we simply check 4(1) + 6(3) + 2(2) + 2(6) + 1(4) = 4 + 18 + 4 + 12 + 4 = 42, a two-digit number divisible by 7. Hence, 12264 must also be divisible by 7.
Gain familiarity with factors and multiples.
Common Core Reference
CCSS.MATH.CONTENT.4.OA.B.4
Divisibility Rules 7 Exercise
Start the following exercises regarding the topic Divisibility Rules 7 and test your knowledge now!
Transcript Divisibility Rules - 7
Mirror, mirror, on the wall, who's the cleverest of them all? The evil witch, Snow White, or her 7 friends Stay tuned to see how this story ends The witch has a notion she thinks is terrific to make a potion that's also a soporific. With Snow White in dreamland, the evil witch can unveil her plan. There's just one glitch, a hitch for the witch To avoid a one-way ticket to heaven, Snow White leans on the Divisibility Rules for the number 7.
In an attempt to trick Snow White, the witch offers her a basket filled with 15, delicious-looking apples. Snow White doesn’t know the apples are laced with a sleeping potion, but she rejects them regardless. Why? Because she can’t divide the 15 apples among the 7 dwarfs evenly, and she doesn't play favorites. The witch is not discouraged. So the very next day, she returns. This time, she has a cart full of apples. The witch doubts that Snow White can calculate such a large quotient quickly and will simply decide to accept the cart and its poisonous contents. The witch proudly declares that she has 543 apples, more than the dwarves and Snow White can ever eat. Again, Snow White refuses because she can’t divide the number of apples evenly into groups of 7. How did she determine this so quickly?
Divisibility by 7
Snow White is a master of the divisibility rule for the number 7, so she doesn’t have to always rely on long division. To check if a number is evenly divisible by 7: Take the last digit of the number, double it Then subtract the result from the rest of the number If the resulting number is evenly divisible by 7, so is the original number. Let’s try the trick on the number of apples in the cart, 543. The last digit is 3, double that to make 6, subtract from 6 from the remaining digits. 54 minus 6 is equal to 48. 48’s not evenly divisible by 7, so 543 isn't evenly divisible by 7 either.
Let's check, just to make sure. 7 goes into 54 seven times. Subtract 49 from 54, bring down the 3, 7 goes into 53 seven times, subtract 49 from 53, which leaves us with a remainder of 4. So we were right! 543 isn't evenly divisible by 7!
Foiled again. What's an evil witch to do? Has Snow White simply outsmarted her?
The evil witch doesn't give up. She gathers all the apples in the kingdom, 2478 to be exact, and delivers them to Snow White. Let’s see.Ok. The last digit is 8. Double it, and we get 16. Subtract 16 from 247. The difference is 231. That’s still a big number, so we just do the same steps again. Double the last digit, that's equal to 2 and 23 minus 2 is equal to 21. 21 is evenly divisible by 7, so the ginormous pile of apples must also be evenly divisible by 7! 7 goes into 24 three times, subtract 21 from 24, bring down the 7, 7 goes into 37 five times.
Subtracting from 37 gives us 2 and 7 goes into 28 exactly 4 times. Whaddya know? Snow White was correct! 2478 IS evenly divisible by 7!
While we were busy calculating, 77 pies are now ready and waiting. Prepared by Snow White with love and care, her pies are famous far, wide and everywhere. And because she's so super sweet, she offers the witch a pie that can't be beat.
Divisibility Rules - 7 exercise
-
State how to use the divisibility rules of the number $7$.
HintsThe first step in checking if a number is divisible by $7$ is doubling the last digit of that number.
After doubling the last digit of the number you are checking, you subtract this new number from what is left of the original number.
For example, with $827$, you double $7$ to get $14$. You then subtract $14$ from $82$ to get $68$. Is $68$ divisible by $7$?
SolutionHere is how Snow White can use the divisibility rules of the number $7$:
To check if a large number is divisible by $7$, she first takes the last digit of the number, and doubles it. In this case, with $543$ apples, she takes $3$, and doubles it to get $6$.
Then she subtracts the new number from the rest of the original number. In this case she subtracts $6$ from $54$, and gets $48$.
If the result is divisible by $7$, so is the original number. If it is not divisible by $7$, the original number wasn't divisible by $7$ either.
In this case, $48$ is not divisible by $7$ because $7$ is not a factor of $48$. Therefore, $543$ is not divisible by $7$ either.
-
Summarize the divisibility rule for $7$.
HintsThere is one mistake in each of the three steps.
Pay careful attention to which operations should be used in each step.
Solution- The first step is to double the last digit, not triple it.
- In the second step, you subtract the result from the remaining digits. You do not use addition.
- Lastly, if the difference is divisible by $7$, then the original number is also divisible by $7$.
-
Explain how to use the divisibility rule of $7$ with large numbers.
HintsThe divisibility rule of $7$ results in a smaller number. If that smaller number is divisible by $7$, then the original number is also divisible by $7$. If the number that the divisibility rules gives you is still very big, then you can repeat the divisibility rule over and over. Eventually you will get a number that is small enough to see if it is divisible by $7$ without having to do long division.
It might help to write out the steps of the divisibility rule of $7$. Comparing your calculations to the steps will help you check for errors.
SolutionSnow White uses the divisibility rules of $7$ to check if $9827$ is divisible by $7$.
She starts by using the divisible rules of $7$ on $9827$, just like she would with a smaller number. She takes the last digit off, doubles it, and subtracts the result from what is left of the original number.
She takes $7$ off of $9827$. Doubling $7$, she gets $14$. She then subtracts $14$ from $982$ to get $968$.
Snow White now has a number that is smaller than what she started with, but is still very big. She knows that she can repeat the same steps, to check if this new number is divisible by $7$. She does not need a new technique or a calculator.
She takes $8$ off of $968$. Doubling $8$, she gets $16$. She subtracts $16$ from $96$ and gets $80$. We then have that $80$ is not divisible by $7$, so she knows that $9827$ is not divisible by $7$ either.
-
Determine which offers are divisible by $7$.
HintsYou can use the the divisibility rules of the number $7$ to check if the offered number of each snack is divisible by $7$.
If you get a large number after using the divisibility rules of the number $7$, you can repeat the steps to get a smaller number.
To check if a large number is divisible by $7$, first take the last digit of the number, and double it.
Then subtract the new number from the rest of the original number.
If the result is divisible by $7$, so is the original number.
SolutionSnow White checks the offered number of each snack to see if it is divisible by $7$. She starts with the $1083$ peanuts.
- She takes the last digit off, doubles it, and subtracts the result from what is left of the original number. Here, Snow White takes $3$ off of $1083$. Doubling $3$, she gets $6$. She then subtracts $6$ from $108$ to get $102$.
- She is not sure if $102$ is divisible by $7$, so she repeats her steps. Snow White takes $2$ off of $102$, and doubles it to get $4$. She subtracts $4$ from $10$ to get $6$.
- Six is not divisible by $7$, so she knows that $1083$ is not divisible by $7$. So she cannot buy the peanuts for her friends.
- $596$ is not divisible by $7$, so she cannot buy the cherry tomatoes.
- $826$ and $7406$ are divisible by $7$, so she can buy the almonds and the blueberries.
-
Explain what it means if a number is divisible by another number.
HintsIn order for two numbers to be called divisible, there cannot be a remainder after you divide them.
Do the divisibility rules take you a longer time to do than doing long division?
Division is reverse multiplication, and factors are numbers we can multiply together to get another number.
Solution- Given two numbers, if you can divide the first by the second without any remainder, the first number is divisible by the second. ✓ True.
- One number is divisible by another, even if you get a remainder when you do the division operation. ✗ False. If you get a remainder, the number is not divisible by the number you used.
- If a large number of apples is divisible by $6$, you can put all the apples into 6 even piles, and have no apples left over. ✓ True. This is equivalent to saying "if a large number is divisible by six, you will not get a remainder when dividing it by 6".
- A divisibility rule is a very long way of determining whether a given number is divisible by a fixed divisor without performing the division. ✗ False. A divisibility rule is a shorthand, or typically faster, way of figuring out divisibility without actually dividing.
- If one whole number is divisible by another number, then the second number is a factor of the first number. ✓ True. Factors are numbers we can multiply together to get another number.
-
Determine which numbers are divisible by $7$.
HintsYou can use the the divisibility rules of the number $7$ to check if the offered number of each supply is divisible by $7$.
When using the divisibility rules of the number $7$ with large numbers, you will need to perform the steps several times in a row.
To check if a large number is divisible by $7$, first take the last digit of the number, and double it.
Then subtract the new number from the rest of the original number.
If the result is divisible by $7$, so is the original number.
SolutionThe dwarfs start off by checking if $89273$ nails are divisible by $7$.
- They take the last digit off, double it, and subtract the result from what is left of the original number. Doubling $3$, they get $6$. Subtracting $6$ from $8927$, they get $8921$.
- This number is still quite large, so they repeat the process until they get a one or a two digit number. Doubling $1$, they get $2$. Subtracting $2$ from $892$, they get $890$. Doubling $0$, they get $0$. Subtracting $0$ from $89$, they get $89$.
- They know that $89$ is not divisible by $7$. So $89273$ must not be divisible by $7$, either.
✓ The $83489$ screws are divisible by $7$.
✓ The $39823$ sticks are divisible by $7$.
✗ The $72382$ planks are not divisible by $7$.
✓ The $29827$ bolts are divisible by $7$.
✗ The $19723$ washers are not divisible by $7$.

Even and odd numbers

Divisibility Rules - 3, 6, 9

Divisibility Rules - 7

Divisibility Rules - 4, 5, 8, 10

Prime Numbers
Integers and their Opposites
Least Common Multiples
Prime Factorization

Adding and Subtracting Rational Numbers on a Number Line
Ordering Rational Numbers
Cube Roots

Rational and Irrational Numbers
Ordered Pairs on the Coordinate Plane
Finding the Greatest Common Factor
Adding and Subtracting Decimals
Comparing Fractions
Equivalent Fractions
Simplifying Fractions
Temperature Conversion

Decimal Expansions
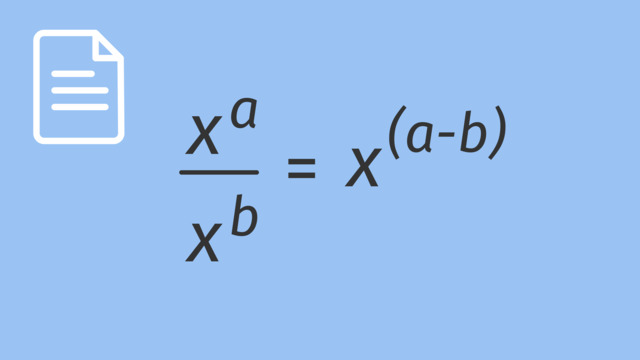
Division with Exponents

How to Convert Decimals Expansions
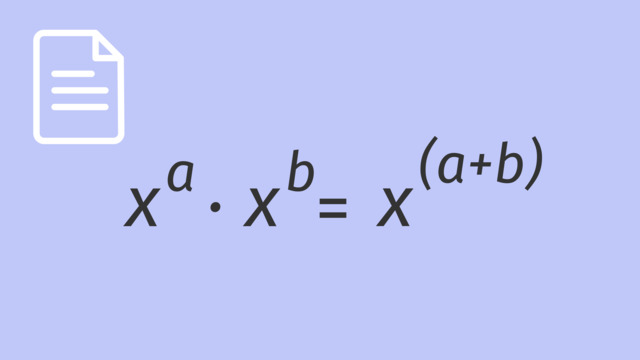
Multiplication with Exponents
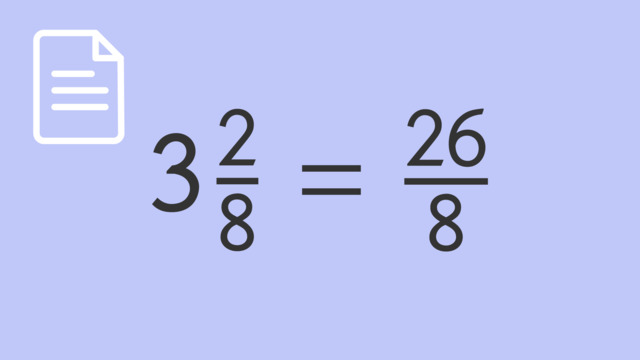
Improper Fractions and Mixed Numbers

Multiplying Mixed Numbers: Word Problems









