Prime Numbers


Basics on the topic Prime Numbers
A prime number is a number that is only divisible by one and itself. Prime numbers are used in cryptography or in creating algorithms that have important security applications. Prime numbers are also useful in generating Pseudo-Random numbers and in creating error correcting codes in computers. Other practical applications of prime numbers are seen in developing machine tools and in designing the metal racks of microwave ovens where utilizing primes help avoid the possibility of harmonics that could harm these machines. Learn how to determine whether a number is prime or not by helping Roscoe, the world’s foremost diamond hunter, identify the rocks which contain diamonds with the aid of his machine that test for prime numbers. Common Core Reference: CCSS.MATH.CONTENT.4.OA.B.4
Transcript Prime Numbers
Meet Roscoe. He’s the world’s foremost diamond hunter and a big fan of prime numbers. No wonder his last find was a 113 karat diamond!
For as long as anyone can remember, other diamond prospectors have been using hammers to look for prime diamonds. However, Roscoe invented a quicker method of identifying which rocks contain diamonds.
He built a machine that tests for prime numbers. The only catch is his machine only works up until the number 120.
Roscoe puts all the rocks he gathered for the day into his machine. His machine then perfoms a series of tests on each rock to see if they are prime.
Roscoe knows that prime numbers are very special. A prime number is only divisible by one and itself. It cannot have any other factors!
Numbers that are not prime are composite numbers, which means they're composed of more factors than one and itself.
For example, 11 is a prime number because its factors are one and 11. While 10 is a composite number. Its factors are 1, 2, 5, and 10.
So, Roscoe’s machine first checks if the numbers on the rocks are divisible by 2. Two is the first prime number. It’s also the only even prime. Because every even number must also be divisible by 2, Roscoe’s machine removes all the rocks that are even numbers greater than 2.
It does not remove the two because the factors of two are just one and itself, and therefore it's prime.
The next number Roscoe’s machine checks for is the number 3.
Just like before, if a number is also a multiple of 3, it means the number is not prime because at the very least it has a factor of 1, 3, and itself. Three is a prime number, so it remains, while all other multiples of three are removed.
After removing all numbers greater than 3 that are divisible by 3, Roscoe’s machine moves on to the number 5. The machine removes all numbers greater than 5 that are also multiples of 5 before moving on to the last step.
Again, the 5 stays because its factors are only 1 and itself.
The last step for Roscoe’s machine is to remove all numbers greater than 7 that are multiples of 7. It makes quick work of these numbers as well.
Let’s take a look at what Roscoe has left over:
All of these numbers are prime except for one special number, which seems to have gotten stuck in the seive.
With one last shake, Roscoe's machine removes the number one, which is by definition NOT a prime number nor a composite.
Now, if we look for factors of each of these numbers that are still remaining, we'll find that all the numbers are only divisble by 1 and themselves.
Roscoe checks each one of the rocks and sure enough, there're diamonds in dem dar rocks!
Roscoe plans to treat himself and spend some of his newfound riches. If only he were a little closer to civilization…
Prime Numbers exercise
-
Determine which numbers are prime.
Hints11 is a prime number, as its factors are 1 and 11. 10 is not a prime number, as its factors are 1, 2, 5, and 10.
There is only one number which is neither prime or composite.
Each number divisible by 2 is at least divisible by 1, 2, and itself. So it can't be a prime number.
But what about 2 itself?
SolutionA prime number is defined as a number which is divisible only by 1 and itself.
Each number which has more factors than 1 and itself is called a composite number.
Remember, though, that 1 is neither a prime or a composite number.
The smallest prime number is 2 and it's the only even prime number. All other even numbers are divisible by 2 as well, and thus are composite numbers.
To determine all prime numbers from 1 up to 120, you can proceed as follows:
- First, sift out 1.
- Next, look at all multiples of 2: you can sift out all those multiples except 2 itself.
- Next, take all the multiples of 3 and sift all of them out except 3.
- Now sift out the multiples of 5, except 5 itself.
- Lastly, sift out the multiples of 7, except 7 itself.
Roscoe checks one of the rocks left over. He takes a hammer and brakes it to find a diamond inside!
-
Use the sieve to find all the prime numbers.
HintsThe numbers which are not highlighted are prime numbers.
All numbers with a green cross are prime numbers. There are five more prime numbers in the sieve.
A prime number is a number which is only divisible by $1$ and itself.
SolutionAll numbers with a green cross are prime numbers.
To cancel out composite numbers step by step:
- First, cancel out the multiples of $2$, i.e. $4$, $6$, $8$, $10$, $12$, $14$, $16$, $18$, $20$, $22$, $24$
- Next, cancel out the remaining multiples of $3$, i.e. $9$, $15$, $21$
- Lastly, cancel out the only remaining multiple of $5$, i.e. $25$
-
Decide if each number is prime or composite.
HintsThe only even prime number is $2$.
Any number ending in $5$ or $0$ is divisible by $5$.
If the sum of the digits of a number is divisible by $3$, then so is the number itself.
Let's have a look at the following example: $1236$.
The sum of the digits is $1+2+3+6=12$, and $12$ is divisible by $3$. Thus, $1236$ is divisible by $3$ too.
SolutionHow can you tell if a number is composite or prime?
Check the divisibility rules:
- Every even number is divisible by $2$.
- Every number whose digits sum to a number divisible by $3$ is also divisible by $3$.
- Each number ending in $5$ or $0$ is divisible by $5$.
- The multiples of $7$ are $7$, $14$, $21$, $35$, $42$, $49$, ...
- The multiples of $11$ are $11$, $22$, $33$, $44$, $55$, $66$, ...
- The multiples of $13$ are $13$, $26$, $39$, $52$, ...
For $\mathbf{124}$, it is an even number and thus divisible by $2$.
For $\mathbf{55}$, it ends in $5$ and so is divisible by $5$.
For $\mathbf{17}$, it is neither divisible by $2$ nor by $3$ nor by $5$, so it's only divisible by $1$ and itself. $17$ is a prime number.
For $\mathbf{37}$, it is neither divisible by $2$ nor by $3$ nor by $5$ nor by $7$ nor by $13$ nor by $17$, so it's only divisible by $1$ and itself. $37$ is a prime number.
For $\mathbf{127}$, it is not divisible by $2$, nor $3$, nor $5$, ... $127$ is a prime number.
For $\mathbf{53}$, we can check it in the same way and see that it is prime as well.
-
Decide the possible side lengths.
HintsKeep in mind the area of a rectangle is given by $A_{\text{rectangle}}=(\text{length})(\text{width})$.
A prime number is a number which is only divisible by $1$ and itself.
If the area is given by a prime number then there is only one choice of side lengths.
If the given area is a composite number then there exists more than one choice of side lengths.
SolutionThe possible side lengths depend on if the area is a prime or composite number:
- If the number is prime there only exists one combination.
- If the number is composite there exist more than one combination.
$\mathbf{15}$ has the factors $1$, $3$, $5$ and $15$. The possible combinations are $1$ and $15$ as well as $3$ and $5$.
$\mathbf{21}$ has the factors $1$, $3$, $7$ and $21$. The possible combinations are $1$ and $21$ as well as $3$ and $7$.
-
Explain what "being divisible by" means.
HintsThe multiples of $3$ are: $3$, $6$, $9$, $12$, and so on...
Keep in mind that $12\div 3=4$.
SolutionIf a number $a$ is a multiple of another number $b$, then we can say that $a$ is divisible by $b$.
In other words, if $a\div b=c$, then
- $a$ is the dividend.
- $b$ is the divisor.
- $c$ is the quotient.
For instance, $14$ is divisible by $7$ if $14\div 7=2$. But $10$ is not divisible by $4$ because $10\div 4=2$ remainder $2$.
-
Check the following statements.
HintsLook for a contradiction if you think that a statement isn't true.
A prime number has no other factors besides $1$ and itself.
SolutionLet's check the statements about prime numbers.
- The product of two prime numbers can't be a prime number because the resulting product has $1$ and itself as factors, as well as the two prime numbers in which it is a product of.
- The sum of two prime numbers could be a prime number, for example $2+5=7$. But in general it isn't. With the exception of $2$, all prime numbers are odd. The sum of two odd numbers is an even number and thus not prime.
- The factors of a composite number are prime numbers. For example, $24=2\times 2\times 2\times 3$.
- There exist pairs of prime numbers whose difference is $2$. For instance, $3$ and $5$, $5$ and $7$, $11$ and $13$, and $17$ and $19$. Such pairs are called twin primes.
- For the last statement, we look for a contradiction. Lets take $13$. It is a prime number and $2\times 13+1=26+1=27$. $27$ is divisible by $3$ and thus not prime. So, this statement isn't true.

Even and odd numbers

Divisibility Rules - 3, 6, 9

Divisibility Rules - 7

Divisibility Rules - 4, 5, 8, 10

Prime Numbers
Integers and their Opposites
Least Common Multiples
Prime Factorization

Adding and Subtracting Rational Numbers on a Number Line
Ordering Rational Numbers
Cube Roots

Rational and Irrational Numbers
Ordered Pairs on the Coordinate Plane
Finding the Greatest Common Factor
Adding and Subtracting Decimals
Comparing Fractions
Equivalent Fractions
Simplifying Fractions
Temperature Conversion

Decimal Expansions
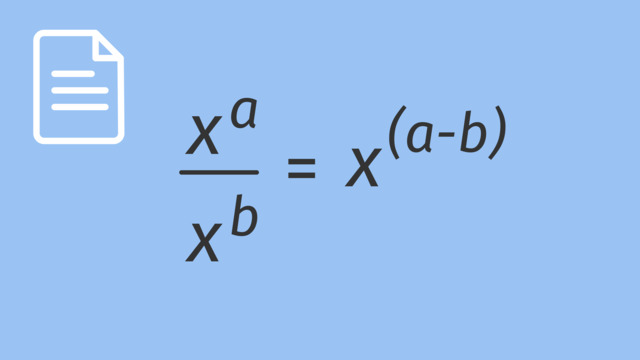
Division with Exponents

How to Convert Decimals Expansions
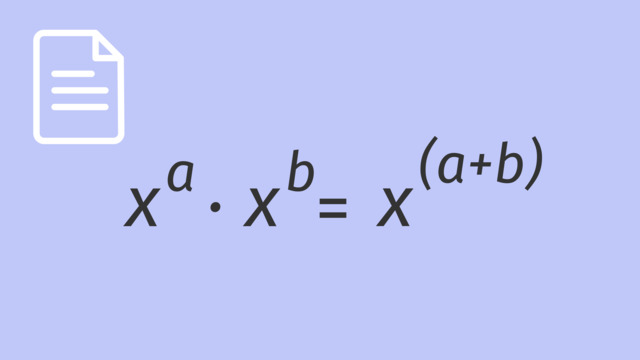
Multiplication with Exponents
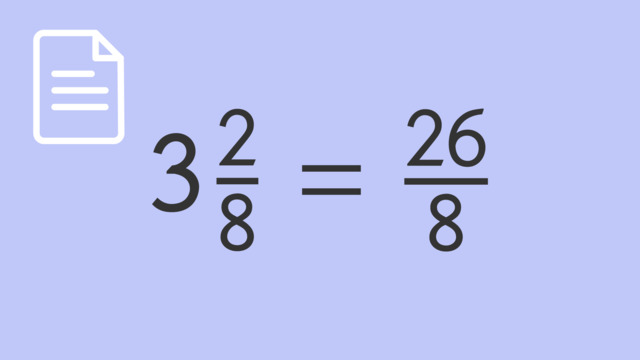
Improper Fractions and Mixed Numbers

Multiplying Mixed Numbers: Word Problems












Are the factors of a composite number prime numbers? That’s what is says in the bonus problem...confusing. The factors of 27 are 1, 3, 9, and 27. 9 is not a prime number.