Transforming Simple Repeating Decimals to Fractions and Vice Versa


Basics on the topic Transforming Simple Repeating Decimals to Fractions and Vice Versa
When you learned about fractions and decimals, you may have noticed that some fractions such one-half, one-fourth, one-eighth, and so on divided out to nice terminating decimals such as 0.50, 0.25, 0.125, and so on. The decimals terminated meaning they ended or stopped.
Other fractions such as 1/3, 1/6, and 1/9 - when you used long division to divide out these numbers to make their equivalent decimals, the remainder just went on and on… repeating over and over, and you indicated the repeating digits with a horizontal bar placed above the repeating digits. Problem solved. But, for repeating decimals, how do you go in the other direction, from decimal to fraction? How do you deal with the repeating digits? What happens to that extra little piece – is it just lost forever?
To change from a repeating decimal into a fraction, there is a trick, and it involves the number 9. Put the repeating number or numbers in the numerator then put the same number of 9s in the denominator and simplify. To see this trick in action and figure out what to do with numbers that go on and on and on, so you can switch from fraction to decimal and back again, watch this video.
Apply and extend previous understandings of operations with fractions. CCSS.MATH.CONTENT.7.NS.A.1
Transcript Transforming Simple Repeating Decimals to Fractions and Vice Versa
The brothers Zooey, Louie and Phooey formed a band called the Musical Triplets and entered their school’s annual Battle of the Bands contest. Look at that?! The Triplets beat last year's winner, the Math Bros. and win first place! The grand prize is a one hundred dollar bill! They plan to divide the prize money evenly amongst themselves.
They go to their Uncle Huge, who's as good as a bank, to exchange their one hundred dollar bill for 10 ten dollar bills. After dividing the money evenly, they're left with one ten dollar bill. They then ask their uncle to change the last ten dollar bill into ten, one-dollar bills. Each boy now gets three dollars, leaving one one-dollar bill. This goes on and on...they exchange dollars for dimes and dimes for pennies until...
Using long division
They divided all the money, except for one last penny. What if they want to split that one remaining penny? We know how to split one cent into three even parts with math. We write this as a fraction one-third but how much is this, exactly? You already know the fraction bar indicates division, so one third is the same as one divided by three.
Do you remember how we calculate the quotient? That's right! We use long division! Now do the math. Do you see a pattern? Since we'll always have a remainder, we call this number, and numbers like this, a repeating decimal. Instead of writing the repeating part again and again, we can use a horizontal bar to indicate the digits that repeat.One third is equal to one divided by three, which we rewrote in long division form. While evaluating this problem, we get zero point three, three, three, three, three, three. Oh, sorry.
Converting a repeating decimal into a fraction
Okay, so you can use division to see if a fraction has a repeating decimal, but how do you convert a repeating decimal into a fraction? Changing a repeating decimal in which all numbers after the decimal repeat, such as zero point one repeating, zero point two repeating, etc. and zero point 73 repeating, all the way up to zero point 123456789 repeating, into a fraction, might seem daunting at first. But I'll show you a little trick that'll amaze your friends or maybe just your math teacher. Use place value to determine the denominator. To write these numbers as fractions, first find out how many numbers repeat after the decimal. Here, there are one, two and three digits to the right of the decimal that repeat, respectively.
Next, you write the repeating part in the numerator like so. For each of the denominators we need to write the same number of 9s as there are numbers in the numerator. So one 9 here, two 9s here and three 9s here. Now all we have to do is simplify! Two-ninths is already in its reduced form, so we don't have to do anything to it. The greatest common factor of 36 and 99 is 9. So we can divide the numerator and denominator by 9. Doing so leaves us with four-elevenths. The greatest common factor of 459 and 999 is 27. So we can divide the numerator and denominator by 27. Doing so leaves us with seventeen-thirty-sevenths. Let's see if the Triplets have figured out the trick to splitting the last penny. Uh-oh, it looks like Uncle Huge is gonna keep that penny! Well, a penny saved is a penny earned!
Transforming Simple Repeating Decimals to Fractions and Vice Versa exercise
-
Explain how to divide 100 dollars evenly between three people.
HintsDivide $9$ by $3$:
$\frac93=\frac{3}{1}=3$
Subtract $9$ from $10$ to get $10-9=1$
- one $\$100$ bill is equal to ten $\$10$ bills
- one $\$10$ bill is equal to ten $\$1$ bills
- one $\$1$ bill is equal to ten dimes
- one dime is equal to ten pennies
SolutionHow can we divide $\$100$ evenly amongst the three band members?
First, we exchange the $\$100$ bills for ten $\$10$ bills.
Each band member gets three $\$10$ bills, but one $\$10$ bill is left.
Next, we have to exchange the last $\$10$ bill for ten $\$1$ bills.
Again, everyone gets three $\$1$ bills and, again, one $\$1$ bill is left.
In the same manner, we can proceed until only one penny is left.
We can describe this situation with a fraction $ \frac13$, which can be written as $1\div3$. This is the part of a penny each member is entitled to.
-
Define a repeating decimal.
Hints$1\div 3=0.3333333........$ is an infinite but periodic decimal.
$0.\bar3$ is a repeating decimal.
SolutionDividing $1$ by $3$ gives us $0.3333...$ with infinite $3$s behind the decimal point. Numbers with one or more repeating decimals are infinite, or without end, and are called repeating decimals.
The number of repeated decimal places can vary:
$\begin{array}{lcl} 0.\bar2 &&1 \text{ repeating number after the decimal}\\ 0.\overline{36} &&2 \text{ repeating numbers after the decimal}\\ 0.\overline{459} &&3 \text{ repeating numbers after the decimal} \end{array}$
All these numbers are written with a horizontal bar above the decimals that are repeated.
For example $0.\overline{459}=0.459459459459.....$.
Repeating decimals are infinite.
-
Decide how much money each band member should receive.
HintsFirst, take a look at the amount of money they will be paid at each location. Then, see how you can divide that amount amongst the $3$ boys.
For example, if the boys got paid $\$110$ for a concert in Plano, Texas, the equation would look like this:
- $\dfrac{\text{total money}}{\text{number of boys}}=\dfrac{\$110}{3}$
SolutionLucky for them, the Musical Triplets are not only excellent singers, they are also very talented mathematicians, so they are able to calculate how much each band member will receive for each gig.
- $\dfrac{\text{total money}}{\text{number of boys}}$
$\begin{array}{rcl} \dfrac{\$160}{3}&=&\$53\frac13\\ \end{array}$
Since we know that $1\div3=0.\overline{3}$, we can say that each band member will be paid $\$53.\bar3$.
Chicago
$\begin{array}{rcl} \dfrac{\$200}{3}&=&\$66\frac23\\ \end{array}$
Since $2\div3=0.\overline{6}$, each band member will be paid $\$66.\bar6$.
Memphis
$\begin{array}{rcl} \dfrac{\$205}{3}&=&\$68\frac13\\ \end{array}$
Each band member will be paid $\$68.\overline{3}$.
Atlanta
$\begin{array}{rcl} \dfrac{\$130}{3}&=&\$43\frac13\\ \end{array}$
Each band member will be paid $\$43.\overline{3}$.
-
Write repeating decimals as fractions.
HintsSimplify the fraction by dividing the numerator and the denominator by their GCF (Greatest Common Factor).
SolutionYou can write a repeating decimal as a fraction as follows:
$0.\overline{123}=\frac{123}{999}$
- We place the repeated numbers in the numerator.
- We place as many $9$s in the denominator as the number of digits that repeat in the numerator.
$\frac{123}{999}=\frac{123\div3}{999\div 3}=\frac{41}{333}$
$~$
1. We can write the repeating decimal $\mathbf{0.\overline{45}}$ as a simplified fraction:
$\begin{align*} 0.\overline{45}&=\frac{45}{99}\\ &=\frac{45\div 9}{99\div 9}\\ &=\frac{5}{11}\\ \end{align*}$
2.We can write the repeating decimal $\mathbf{0.\overline{324}}$ as a simplified fraction:
$\begin{align*} 0.\overline{324}&=\frac{324}{999}\\ &=\frac{324\div 27}{999\div 27}\\ &=\frac{12}{37}\\ \end{align*}$
3.We can write the repeating decimal $\mathbf{0.\overline{18}}$ as a simplified fraction:
$\begin{align*} 0.\overline{18}&=\frac{18}{99}\\ &=\frac{18\div 9}{99\div 9}\\ &=\frac{2}{11}\\ \end{align*}$
4.We can write the repeating decimal $\mathbf{0.\overline{132}}$ as a simplified fraction:
$\begin{align*} 0.\overline{132}&=\frac{132}{999}\\ &=\frac{132\div 3}{999\div 3}\\ &=\frac{44}{333} \end{align*}$
-
Convert the fraction $\frac13$ into a decimal by using long division.
HintsYou have to do the same steps over and over again.
To show that a decimal is a repeating decimal, place a horizontal line over the repeating numbers.
SolutionYou already know that a fraction bar indicates a division.
So we can say that $\frac13=1\div3$.
But how can you divide $1$ by $3$? On the right, you can see the problem worked out.
If we use long division, we will always have a remainder of $1$. We can repeat this process over and over and OVER again.
$1\div 3=0.33333......$.
We call this a repeating decimal and indicate the repeating portion by placing a horizontal line over the numbers:
- $0.\overline3$
- $0.\overline{623}$
- $0.\overline{1025}$
-
Determine the repeating decimal equivalent for each given fraction and vice versa.
HintsThe factors
- of $99$ are: $1$, $3$, $9$, $11$, $33$, and $99$
- of $999$ are: $1$, $3$, $9$, $27$, $37$, $111$, $333$, and $999$
Is $37$ a factor of $99$?
Is $37$ a factor of $999$?
You can write every repeating decimal in fraction form in which the denominator has as many $9$s as the number of digits that repeat in the numerator.
For example $0.\overline{1234}$ would look like this as a fraction:
- $\dfrac{1234}{9999}$
SolutionWhen transforming fractions into repeating decimals...
- You can use long division
- Or you can multiply the fraction so that the denominator is $9...9$
For the fraction $\frac{46}{111}$:
If we multiply both the numerator and the denominator by $9$ we get:
$\begin{array}{rcl} \frac{46}{111}&=&\frac{46\times 9}{111\times 9}\\ &=&\frac{414}{999}\\ &=&0.\overline{414} \end{array}$
$~$
For the fraction $\frac{4}{27}$:
We multiply both the numerator and the denominator by $37$ to get:
$\begin{array}{rcl} \frac{4}{27}&=&\frac{4\times 37}{27\times 37}\\ &=&\frac{148}{999}\\ &=&0.\overline{148} \end{array}$
$~$
To transform repeating decimals into fractions:
- write all repeating numbers in the numerator
- in the denominator, place as many $9$s as there are repeating digits
- reduce the fraction by dividing the numerator and denominator by the GCF
For the repeating decimal $0.\overline{81}$:
$\begin{array}{rcl} 0.\overline{81}&=&\frac{81}{99}\\ &=&\frac{81\div9}{99\div 9}\\ &=&\frac{9}{11} \end{array}$
$~$
For the repeating decimal $0.\overline{63}$:
$\begin{array}{rcl} 0.\overline{63}&=&\frac{63}{99}\\ &=&\frac{63\div9}{99\div 9}\\ &=&\frac{7}{11} \end{array}$

Variables
Simplifying Variable Expressions
Evaluating Expressions

How to do Order of Operations?

Distributive Property
Adding Integers
Subtracting Integers

Multiplying and Dividing Integers
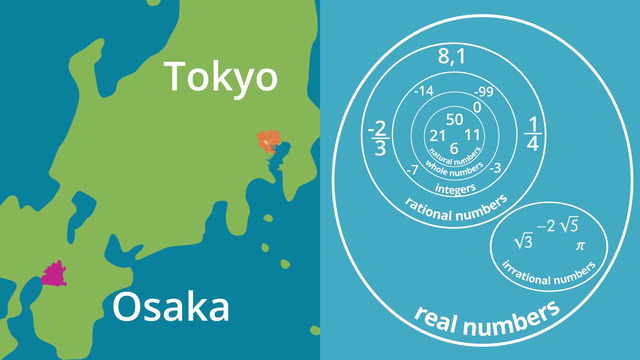
Types of Numbers
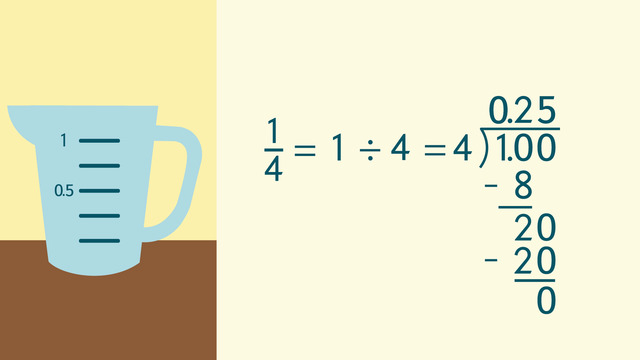
Transforming Terminating Decimals to Fractions and Vice Versa

Transforming Simple Repeating Decimals to Fractions and Vice Versa
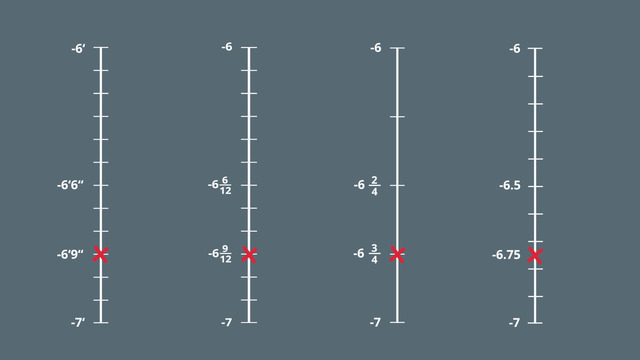
Rational Numbers on the Number Line
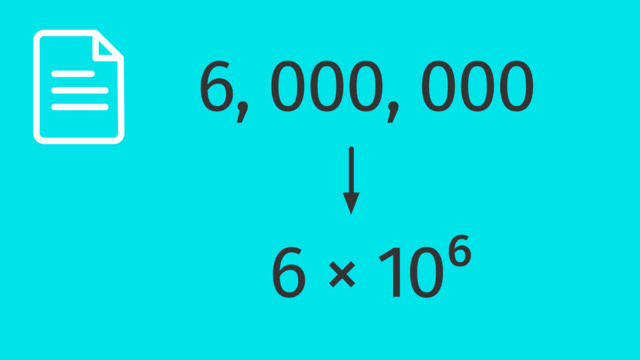
Standard and Scientific Notation

Using Operations with Scientific Notations











