Variables


Basics on the topic Variables
In math, you often use variables: letters of the alphabet (and sometimes even Greek letters) that represent an unknown value, or a quantity that can vary.
With variables, you can represent variable quantities, such as a price that varies, the number of items you wish to buy, or a gym membership where the overall costs vary depending on the number of months you are willing to be a member, and so on.
So in math, expressions and equations often use variables. But variables are also fundamental components of functions, where one input, x, has only one output, usually y. Any letter or symbol can represent a variable, or unknown, as they're sometimes called; it makes no difference whether you use a, k or x.
But there are some conventions in math. Certain variables are used in specific contexts, like y = mx + b or ax² + bx + c in Algebra. For example, in Geometry, Greek letters are often used for angles, capital letters are used for vertices and lowercase letters are used for sides.
In mathematical rules, laws, theorems, and standardized formulas, x usually represents the unknown value you have to solve for, while variables like a, b, c, or m are used as parameters or coefficients, where the rule always works the same, no matter which integer or real number you substitute in. In functions, you'll find that x and y are used most of the time, where y stands for a value that depends on the value of the variable x.
Interpret the structure of expressions. CCSS.MATH.CONTENT.HSA.SSE.A.1
Transcript Variables
Sarah is kicking Eric`s butt at a card game. The goal of the game is to make a straight with five cards.
Sarah draws another card. She got lucky, it's a wildcard. Let's have a look at her hand. Sarah has a good hand. She already has a two, three, four, and five. And with the wildcard she's the winner! The wildcard can represent the one card or it can also represent the six card. A wildcard can take on the value of any card in the deck.
Variables in Algebra
In Algebra, there is a concept that is similar to the wildcard. Variables are letters or other symbols used to represent unknown numbers in algebraic equations and expressions.
We can say: A variable is a letter or symbol that represents a quantity that can vary. For example x, y or a or whatever you choose. A variable can represent an unknown number in an equation. In this example subtraction equation, the variable, x, is equal to two.
Using Variables in Algebraic Expressions
Let's see how variables can be used in algebraic expressions.
Imagine you have a smartphone contract. The monthly charge for your cell phone is the fixed, monthly cost plus fifteen dollars per gigabyte of data. The variable x is equal to the number of gigabytes. You can write this as an algebraic expression: 25$ + 15$ · x. With this expression, you can calculate your monthly charge depending on what data plan you need.
Who is the mystery person with whom Sarah keeps texting?
Variables exercise
-
Complete the definition of a variable.
HintsThe wild card could represent a $1$ or a $6$.
Sarah has a choice of which value the wild card should take.
SolutionThe words filled in are value, algebra, variables, equation, and value
Sarah's playing cards against Eric. The goal is to make a straight. This means the value of all five of the cards should be consecutive: for example, $1$, $2$, $3$, $4$ and $5$.
The four cards Sarah already has have consecutive values from $2$ to $5$, inclusive.
Sarah draws another card. It's the wild card. What does a wild card mean? A wild card can represent any card in the deck. Considering Sarah's cards, the wild card could represent the $1$ or $6$, therefore completing her straight.
In Algebra, there exists a concept similar to the wild card, variables are unknowns in an expression or equation. Variables can be $x$, $y$, $a$ or any other letter.
Have a look at the mathematical expression $7-x$. The variable is $x$. We can plug in different values for $x$:
- When $x=3$, $7-3=4$ or
- When $x=1$, $7-1=6$ ...
-
Find the appropriate expression that describes the cost of Sarah's mobile phone service.
HintsStart with the fixed costs.
Sarah has to pay the fixed monthly costs each month.
The cost for data usage depends on the number of gigabytes Sarah uses each month.
- $1~GB$ costs $\$~15$,
- $2~GB$ costs $\$~30$,
- $3~GB$ costs $\$~45$,
- ...
In mathematics, numbers are typically written to the left of the variable(s) when indicating multiplication.
i.e. $4x$, $12xy$, $123x^2y$ etc.
not: $x4$ $y12x$, etc.
SolutionThe monthly cost of Sarah's mobile phone:
- Fixed monthly cost: $\$25$
- Each GB of data used: $\$15$
- The variable $x$ represents the number of gigabytes used by Sarah.
$\$25+\$15\times x$
...or without the multiplication operator:
$\$25+\$15x$
Since addition is commutative, we can also change the order like so:
$\$15x+\$25$
Here, we can replace $x$ with any value, for example $x=4$. The meaning of $x=4$ is that Sarah has used $4\text{GB}$ during a given month. So now we can compute the cost of her mobile phone bill for the month:
$\begin{array}{rcl} \$25+\$15\times 4 &=&\\ \$25+\$60 &=& \$85 \end{array}$
-
Calculate which rate plan is better for Sarah.
HintsSarah has to pay the fixed costs no matter what.
The variable cost depends on the number of gigabytes used in a month, $x$, and the cost per gigabyte of data used by Sarah.
Plug in different values for $x$, the number of gigabytes Sarah uses in a month.
The fixed cost of choice (A) is higher than that of choice (B).
On the other hand, the variable cost of choice (B) is higher than that of choice (A).
Therefore, we know that choice (B) would be more favorable if Sarah plans to use $2$ gigabytes of data or less. Choice (A) would be more favorable if Sarah uses $2$ or more gigabytes per month.
SolutionThe expression for choice (A) is the same as seen in the video: a fixed cost of $\$25$ and $\$15$ for each GB used by Sarah gives us the expression:
$\$25+\$15\times x$
Similarly, for choice (B), the fixed cost is $\$15$ and $\$20$ for each GB used by Sarah in a month, giving us
$\$15+\$20\times x$
Now we can plug in different numbers for $x$:
If Sarah uses 1 gigabyte of data, she has to pay
- (A) $\$25+\$15\times 1=\$40$
- (B) $\$15+\$20\times 1=\$35$
If Sarah uses 2 gigabytes of data, she has to pay
- (A) $\$25+\$15\times 2=\$55$
- (B) $\$15+\$20\times 2=\$55$
If Sarah uses 5 gigabytes in a month, she has to pay
- (A) $\$25+\$15\times 5=\$100$
- (B) $\$15+\$20\times 5=\$115$
So we can conclude the following:
- if Sarah uses 1 GB of data, choice (B) is better...
- if Sarah uses 2 GB it doesn't matter which choice she chooses and...
- if Sarah uses 3 or more gigabytes of data, then choice (A) is better.
-
Calculate the value for the variable $x$.
Hints$x$ is the total number of eaten pancakes. So you have to add all the pancakes eaten by Mrs. Harper and her family.
Thanks to the Commutative Property of Addition, you can change the order in which you add the numbers.
For example:
- $a+b=b+a$
You cannot, however, change the order of subtraction.
For example:
$\begin{array}{rcl} 3-2&=&1\\ &\neq&\\ 2-3&=&-1 \end{array}$
SolutionMrs. Harper makes $20$ pancakes.
We can figure out how many pancakes her family eats by adding all the numbers:
$x=4+6+3+3=16$
The number of pancakes left over is given by the expression $20-x$. Now we can plug in $x=16$ into this expression and we get:
$y=20-16=4$
So now we know that there are only $4$ pancakes left.
-
Determine which of the following are variables.
HintsA number isn't a variable.
A variable is a letter or a symbol that represents a quantity that can vary.
An operator is also not a variable.
SolutionA variable is a letter or a symbol that represents a quantity that can vary like $x$, $y$ or $a$, just to name a few.
In the expression $7-x$, the variable is $x$. $7$ is a number and therefore not a variable. The operator $-$ and the equal sign $=$ aren't variables either.
Depending on what we plug in for the variable $x$, we get different results. For example, if we plug in $3$ for the variable $x$ we get:
$7-3=4$
If we plug in $6$ we get:
$7-6=1$
And so on. As you can see, the result depends on the number we plug in for the variable $x$.
-
Identify the correct value for $x$ for each equation.
HintsPlug in the different values of $x$ to see if you get a correct statement.
SolutionVariables are used in equations quite often.
Equations always include an equal sign and state that the expression on the left side and the expression on the right side are equal.
In the equations we used, there is only one possible value for $x$ that, if plugged in for $x$, makes each statement correct.
Here, the best way is to simply plug in all the values and see if you get a correct answer where both sides of the equal sign are the same, like $7=7$. If you get a statement like $3=7$, the value for $x$ is incorrect.
Later on, the goal isn't to guess a value for the variable, but to solve the equation.

Variables
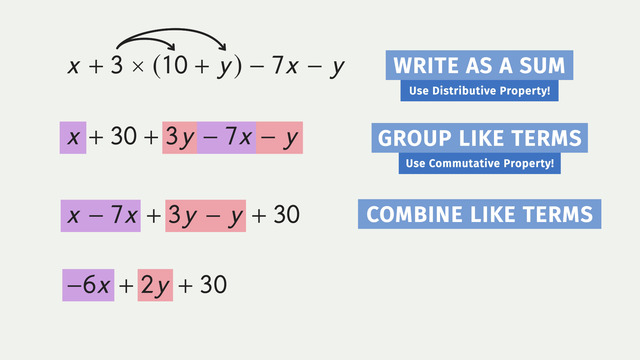
Simplifying Variable Expressions
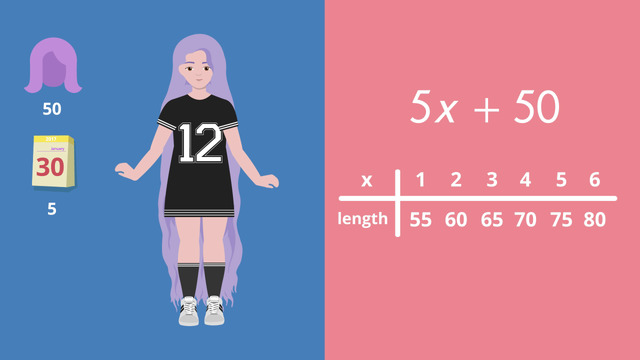
Evaluating Expressions

How to do Order of Operations?

Distributive Property
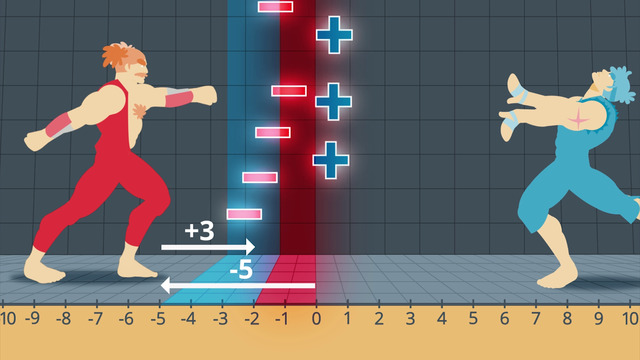
Adding Integers
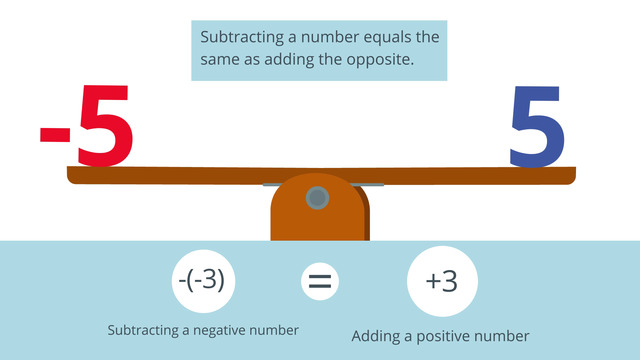
Subtracting Integers

Multiplying and Dividing Integers
Types of Numbers
Transforming Terminating Decimals to Fractions and Vice Versa

Transforming Simple Repeating Decimals to Fractions and Vice Versa
Rational Numbers on the Number Line
Standard and Scientific Notation

Using Operations with Scientific Notations












@Austin O. it really does
honestly what even is this
this video reminds me of a low-budget anime
heheh Please, don't do that! We're always looking for new ways to explain math using stories. I hope you'll keep coming back to see what we've done...and hopefully, you'll learn something new!
This is a wonderful video! I almost wish I could forget what I learned to learn it again!