Simplifying Variable Expressions

Basics on the topic Simplifying Variable Expressions
Before evaluating expressions or solving equations, your goal is to always simplify as much as possible. To do this, you should follow some simple steps, especially where variables are concerned. You should know how to use the Distributive Property as well as the rules for combining variables.
If you have complex expressions or equations, you first look for addition or subtraction inside the parentheses. Then, you can simply follow the rest of the order of operations (PEMDAS, BEDMAS, BODMAS, BIDMAS). Afterwards, you group like terms before combining like terms.
In case you were wondering what like terms and unlike terms are and how to group and combine like and unlike terms when variables are involved, this video will enlighten you. If you had problems with what to combine, always keep in mind, that different variables should be treated like different species in real life. That being said, you will master simplifying variable expressions in no time, promised.
Don't try to sneak past this topic in maths if you have difficulties; simplifying variable expressions is one of the most important issues for all your future math topics and in our video it will be explained step by step to you. Relax, lean back, watch and learn.
Use properties of operations to generate equivalent expressions. CCSS.MATH.CONTENT.7.EE.A.1
Transcript Simplifying Variable Expressions
Algebraic expressions with more than one variable can look scary. To make variable expressions easier to solve, simply simplify! Don't be frightened; I will walk you through the steps.
You can only add and subtract like Terms
This expression has two different variables: 3x + 2y. Hmm… How do you simplify this expression? Maybe you're thinking 3x + 2y = 5xy. STOP! This is wrong! You can't simplify this expression anymore. Combining terms with x and terms with y is not possible.
It's almost as wrong as Dr. Evil attempting to create a “Sharkuine” – by combining a shark with a penguine! Just like sharks and penguins, variables such as x and y are not the same. Repeat to yourself: You can only add and subtract like terms! When adding and subtracting variables, never, ever combine unlike terms. Never, ever!
Example how to simplify Variable Expressions
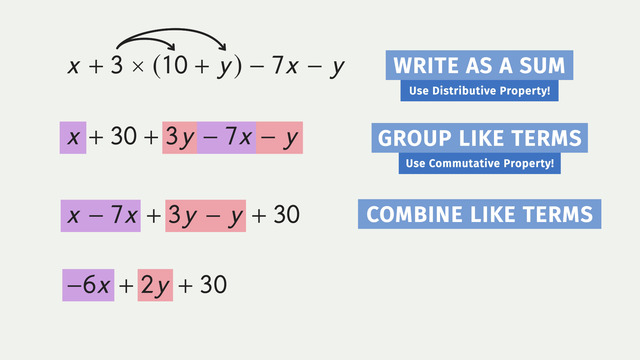
Let’s try out this example. We will go step by step. x + 3 · (10 + y) − 7x − y.
- Step 1 is to write out the expression as a sum. See the parentheses? You will need to use the Distributive Property to multiply the three times the binomial contained inside the parentheses. Now you have: x + 30 + 3y − 7x − y.
- Step 2 is to group the like terms. You can use the Commutative Property to move the terms. Place the x’s with x’s, the y’s with y’s, and so on. This will make the next step easier: x − 7x + 3y − y + 30.
- Now, Step 3 is to simplify the expression by combining the like terms. For terms that have numbers and variables, remember the coefficient tells us how many there are of each variable: −6x + 2y + 30.
Ok, let’s go over this one more time:
- Step 1: Write the expression as a sum. If there are parentheses, use the Distributive Property.
- Step 2: Move the terms around to group the like terms by using the Commutative Property.
- And lastly, Step 3, simplify the expression by combining the like terms.
Most important: Never, ever try to combine unlike terms. Here is your reminder: Don’t create sharkuins! Don’t make the same mistake as Dr. Evil. He had to learn the hard way.
Simplifying Variable Expressions exercise
-
Determine whether or not the terms can be combined.
HintsIn math, you are only allowed to combine like terms.
Variables that are not the same are called unlike terms.
Only two of the choices have terms that you can combine.
SolutionWe can't combine a shark and a penguin to make a sharkuin or a penguishark.
In math, this is like the concept of only being able to combine like terms. We only can combine terms that have the same combination of numbers or variables.
$3x+2y$
The addends have different variables, so we can't combine them.$x-7x$
Both of these terms have an $x$, so we can combine the two to become $-6x$.$x+30$
The addends in this expression are unlike terms because the $30$ is missing an $x$ and therefore are not able to be combined.$3y-y$
We see that we can combine those terms by subtracting the coefficients. Which leaves us $2y$.$-7x+3y$
The terms have different variables and therefore are not able to be combined. -
Simplify the given expression.
HintsUse the Distributive Property to transform $3\times(10+y)$ to $3\times 10+3y=30+3y$.
Use the Commutative Property. This means:
$a+b=b+a~~$ or $~~a-b=-b+a$Remember, you can only combine like terms.
For example, $x$ and $3y$ aren't like terms.
Solution$\begin {array} {rcl} x+3\times(10+y)-7x-y & = & ~~~x + 3\times 10 + 3y - 7x - y\\ & = & ~~~x+30+3y-7x-y\\ & = & ~~~x-7x+3y-y+30\\ & =& -6x+2y+30 \end {array}$
To simplify the expression...
- We remember that we can only combine (add or subtract) like terms.
- Since we have to follow PEMDAS, we have to use the Distributive Property to rewrite the expression as a sum.
- The next step is grouping like terms. Here, we use the Commutative Property.
- Finally, we combine like terms by adding or subtracting their coefficients.
- This gives us the simplified expression, seen above.
-
Analyze the expressions for terms that can be simplified.
HintsYou have to identify like terms. For example,
- $3x$ and $x$ are like terms
- $3x$ and $y$ are unlike terms
Two pocket calculators plus three pocket calculators gives you five pocket calculators.
On the other hand, two pocket calculators and three cell phones cannot be combined.
Like terms have the same combination of variables and the exponents are of the same degree.
SolutionWhen we simplify expressions, we first have to identify the like terms.
Always remember: Never ever combine unlike terms.
The like terms will be grouped as follows:
$\begin{align*} 2x-3y+5x+3&=2x+5x-3y+3\\ &=7x-3y+3 \end{align*}$
$\begin{align*} 12+y+x+4y+3y-4x+2+x^2&=x^2+x-4x+y+4y+3y+12+2\\ &=x^2-3x+8y+14 \end{align*}$
$\begin{align*} 2xy-2x-2y-2+7+5x+3y&=2xy-2x+5x-2y+3y-2+7\\ &=2xy+3x-y+5 \end{align*}$
-
Simplify the following terms.
HintsRemember, you only can combine like terms.
Like terms are
- $3x$, $-2x$, $x$, ...
- $y$, $-4y$, $3y$, ...
- $12$, $3$, $-7$, ...
Group like terms. For example:
$\begin{array}{rcl} x - 7 + y - 3y + 4 &=& \\ x + y - 3y - 7 + 4 &=& \\ &=& x - 2y - 3 \end{array}$
If you have located two like terms, you can add or subtract them by adding or subtracting the coefficients. For example:
- $4y + 2y = (4 + 2)y = 6y$
- $3x - 5x = (3 - 5)x = -2x$
SolutionCombining sharks with penguins is similar to adding or subtracting $3x$ and $2y$. This is impossible.
We can only combine, add or subtract, like terms. First, we locate the like terms and put them into groups. Then, we can add or subtract like terms by adding or subtracting the coefficients.
It's important to note the following: Never, ever combine unlike terms.
- $2x + 3x = 5x$
- $2x + 3 + x = 2x + x + 3 = 3x + 3$
- $2x + 3y + 2y = 2x + 5y$
- $2y + 2 + y = 2y + y + 2 = 3y + 2$
-
Identify the correct rule.
HintsYou know $10+5=15$. Therefore, numbers can be added. Numbers are like terms.
$10\times(3+y)=30+3y$ using the Distributive Property. As you can see, the multiplication of a number and a variable $y$ is allowed.
For example, $x-7x=-6x$ but $3x+2y$ can't be simplified.
SolutionNever, ever combine unlike terms.
Phrased a bit differently, this means that only like terms can be combined. Only like terms can be added or subtracted.
$\mathbf{3y - y = 2y}$
- Since these are like terms (both terms contain the variable $y$, the coefficients can be subtracted, leaving us with $3y - 1y = 2y$.
- These terms each have a different variable. No matter how hard we try, they aren't combinable.
-
Examine the following expressions.
HintsRemember: Never, ever combine unlike terms.
To write an expression as a sum, use the Distributive Property:
$a \times (b + c) = a \times b + a \times c$
Combining like terms means adding or subtracting the coefficients of the variables.
SolutionThe three steps for simplifying expressions are:
- Write the expression as a sum using the Distributive Property.
- Group like terms.
- Combine like terms.
$2 \times (x + y) - 2x + 3y + 1$ can be written as a sum as follows:
$2x + 2y - 2x + 3y + 1$
Now, we group like terms to get:
$2x - 2x + 2y + 3y + 1$
Finally, combining like terms leaves us with the simplified term:
$5y + 1$
$~$
Example 2
$7(x + 4) + 2x - 3 + y$ written as a sum using the Distributive Property:
$7x + 28 + 2x - 3 + y$
Grouping like terms leads to:
$7x + 2x + y + 28 - 3$
Finally, we combine like terms to get:
$9x + y + 25$

Variables

Simplifying Variable Expressions
Evaluating Expressions

How to do Order of Operations?

Distributive Property
Adding Integers
Subtracting Integers

Multiplying and Dividing Integers
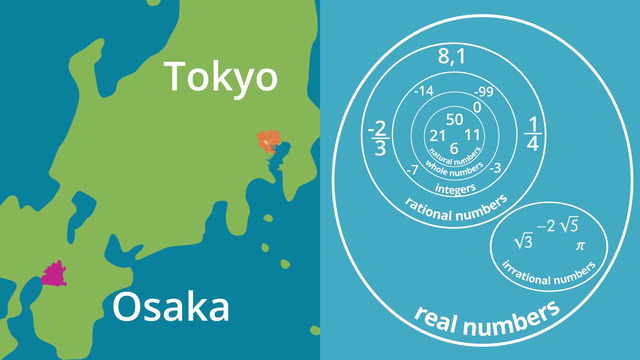
Types of Numbers
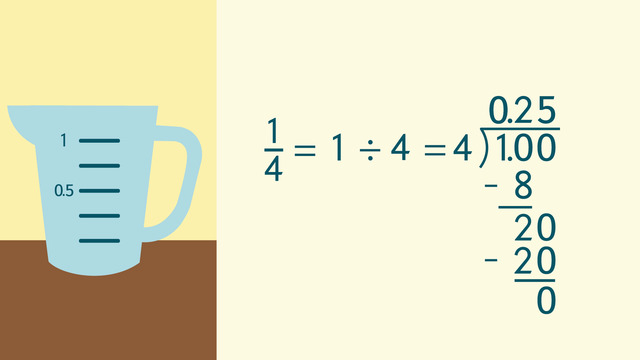
Transforming Terminating Decimals to Fractions and Vice Versa

Transforming Simple Repeating Decimals to Fractions and Vice Versa
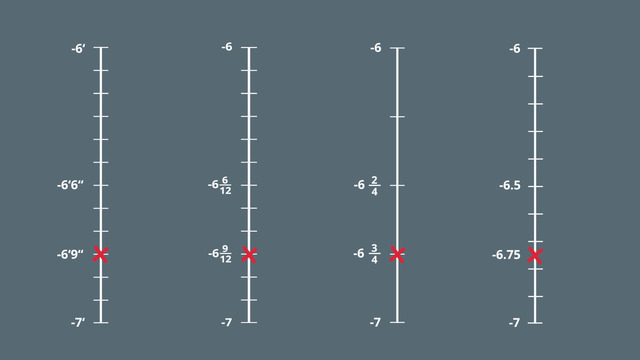
Rational Numbers on the Number Line
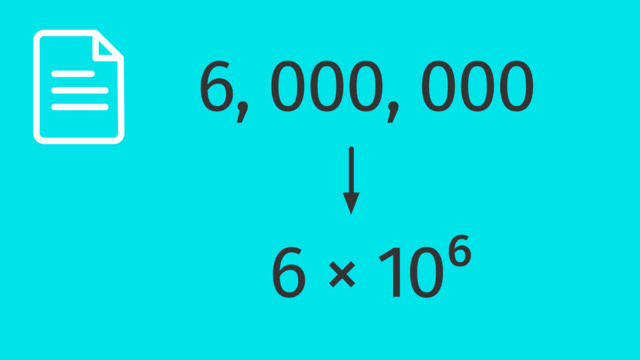
Standard and Scientific Notation

Using Operations with Scientific Notations











