Distributive Property


Basics on the topic Distributive Property
The Distributive Property is an easy rule which you need for all your future math magic.
It helps you with simplifying equations and simplifying expressions, whenever you come across a sum (addition) or difference (subtraction) inside parantheses (brackets) that you have to multiply (multiplication) by a factor.
For example: 2·(3+4).
The Order of Operations, or PEMDAS (BEDMAS, BODMAS), tells you, to first start with the addition inside the parentheses (brackets), giving you 2·(7). Now you multiply by 2 and end up with 14.
But what happens, if instead of simple numbers or like terms you have to operate with unlike terms such as 2·(3-y)? Is there any chance to simplify this expression?
Here, the Distributive Property comes into play. It tells you how to solve any combination of terms looking like a·(b+c) or a·(b-c), where you can put in any number or monomial for a, b, and c. The Distributive Property tells you that if you have to multiply a sum by any factor, you can multiply each summand by this factor and add the resulting products. This works as well with subtraction inside the parentheses!
In this video you will discover the Distributive Property step by step with the help of a real-life example and learn in this lesson how to use it the right way. After watching the video you will never, ever have to be frightened again by scary-looking parentheses with sums or differences of unlike terms inside and with factors in front of them, such as x(a+b) or x(a-b).
With this method, you can simplify unlike terms such as 2·(3-y) into 6-2y. See? This doesn't look as difficult as before, does it?
Do you want to know how it works? Start the video right away and never get lost in expressions or equations with terns like a(b+c) again.
Use properties of operations to generate equivalent expressions. CCSS.MATH.CONTENT.7.EE.A.1
Transcript Distributive Property
The Distributive Property is one of the most frequently used math properties but many students have trouble recalling it. I will tell you a story to help you remember and apply this very important math property.
Distributive Property Example 1
Meet Anton and Bella. They both love ice cream. Imagine, on a hot summer day, each goes to buy scoops of ice cream. First, Anton buys a cone. There are three scoops of ice cream in his cone. You can express this mathematically. The three scoops of ice cream represent the number three, and Anton represents the number one. Three times one is three.
Next, Bella buys a cone. She also has three scoops of ice cream on her cone. You can also express this as the equation three times one. Anton and Bella meet! We can write this as a sum. Three times one plus three times one. They have six scoops of ice cream all together. Let's rewind back to the beginning of the story...
Distributive Property Example 2
Meet Anton and Bella, again. This time they meet each other first. You can express this as a sum. Anton plus Bella. Then, together they go to buy ice cream. You can express this as three multiplied by the sum of one plus one. Altogether they have six scoops of ice cream. Wait, isn't this the same outcome as the first version of this story?! Yes: It doesn't matter if they first buy their ice cream and meet then or first meet and buy it together. The result is the same.
Application of the Distributive Property
Let's write this using math symbols. Let A stand for Anton and B stand for Bella. C is the number of scoops. Now distribute... C times A plus C times B.
Let's try the Distributive Property out, using different numbers: three times the sum of four and five. You can distribute the three to each addend and then add the products. Three times four plus three times five which is equal to twenty-seven. Or you can follow order of operations and multiply the sum of the addends. Three times the quantity four plus five which equals three times nine is also equal to twenty-seven. Try it yourself. Assign different numbers for the variables A, B, and C and observe that the result will be the same, every time. Let's make a note:
- The Distributive Property says that distributing a multiplier over a sum of numbers will result in the same answer as multiplying each addend separately and then summing.
- C times the sum of A and B equals the same as C times A plus C times B.
Next time you need to use the Distributive Property, remember Anton and Bella!
Distributive Property exercise
-
Describe what the Distributive Property means for Bella and Anton.
HintsDon't worry about capital and small letters. They can be used the same way. But remember, you should always be consistent when naming variables.
When mathematical expressions are equal to each other, it does not matter whether you write $a=b$ or $b=a$.
Take a look at both of the longer terms. What do they describe? Do they have a different result?
SolutionThe Distributive Property is very useful for calculating things in everyday life because it helps you simplify terms.
Let's take a look at how Bella and Anton have applied the Distributive Property. As we have seen in previous examples, it does not matter if they buy their ice cream and then meet or first meet and buy the ice cream together.
What does this mean? We can express with the following mathematical expression:
$3 \times 1 + 3 \times 1 = 3 \times (1 + 1) = 6$
In general, we know that the Distributive Property says that distributing a multiplier over a sum of numbers will result in the same answer as multiplying each addend separately and then summing.
-
Explain how to use the Distributive Property for scenarios with more than 3 scoops of ice cream.
HintsFirst check whether it works with more than $3$ scoops of ice cream.
Finally there should be a general formula for the Distributive Property.
SolutionBella has recognized that her equation does not depend on the number of scoops. So she tries to increase the number of scoops to $4$. Let's take a look at what she finds:
- $4 \times (1 + 1) = 4 \times 1 + 4 \times 1 = 8$
- $4 \times (1 + 1) = 4 \times 2 = 8$
Generalizing this, we just have to substitute the numbers with variables. Therefore, we substitute the number of scoops with $c$, Anton with $a$ and Bella with $b$. The following formula describes our situation using the Distributive Property.
- $c \times (a + b) = c \times a + c \times b$
-
Describe how the Distributive Property changes when a third kid buys ice cream.
HintsThe Distributive Property is not limited to a certain number of addends inside the parentheses.
Introducing the variable $d$, which stands for the Dave, allows us to extend the formula to $c \times (a + b + d)=c \times a + c \times b + c\times d$.
SolutionThe Distributive Property is not limited to a certain number of addends inside the parentheses. This enables us to evaluate the number of ice cream scoops even when Dave joins Bella and Anton.
Therefore, the total number of scoops is: $4 \times (1 + 1 + 1)$.
Again, we can solve this in two different ways:
- We could distribute the multiplier over the sum inside the parentheses first. This gives us $4 \times 3 = 12$.
- Alternatively, we could multiply each addend separately and then sum. Using this method we get $4 \times 1 + 4 \times 1 + 4 \times 1 = 4 + 4 + 4 = 12$.
-
Use the Distributive Property to demonstrate what happens when Mrs. Harper bakes fewer pancakes.
HintsThe Distributive Property works with subtraction, too.
We can express that with variables: $c \times (a - b) = c \times a - c \times b$
In general we can say $c \times \left( a \pm b \right) = c\times a \pm c \times b$. The sign $\pm$ stands for either $+$ or $-$.
SolutionThe Harper family really loves pancakes. Every Sunday morning, each member of the Harper family eats $3$ pancakes. Fortunately, Mrs. Harper knows how to use the Distributive Property. Her normal calculation goes like this:
- $3\times ( 1 + 1 + 1 +1 + 1+ 1) = 3 \times 6 = 18$.
- $3 \times ( 1 + 1 + 1 +1 + 1+ 1 - 1 - 1) = 3\times (6-2)$.
- She could calculate the parentheses first. Solving it this way, she gets $3 \times 4$, giving her $12$.
- Alternatively, she can multiply each item inside the parentheses separately. This also gives her $3 \times 6 - 3 \times 2 = 18 - 6 = 12$.
-
Use the Distributive Property to solve the expression: $3 \times (4 + 5)$.
HintsThe Distributive Property says that distributing a multiplier over a sum of numbers will result in the same answer as multiplying each addend separately and then summing.
As a formula, this idea is expressed as follows: $c \times (a + b) = c \times a + c \times b$.
SolutionThere are infinite examples where you can use the Distributive Property. Let's take a look at one of them. The general formula is: $c \times (a + b) = c \times a + c \times b$. So we can replace the $c$ with $3$, $a$ with $4$ and $b$ with $5$. What we're left with is $3 \times (4 + 5)$.
We always have two different possibilities when solving these types of problems:
- We could calculate the sum of $4+5$ first. We get $9$, which has to be multiplied by $3$. This leaves us with $27$.
- The second way is to multiply each addend separately and then sum the resulting numbers, giving us $3 \times 4 + 3 \times 5$. As we can see, the result is the same: $12 + 15 = 27$.
-
Simplify the expression $(27 + 12 - 21) \div 3$ using the Distributive Property.
HintsThe Distributive Property also works with division.
Pay attention to the single operator inside the parentheses.
SolutionThe Distributive Property can include a divisor, too. The difference is that we do not multiply the content of the parentheses, but divide it. As you may have learned in school, you can divide a dividend by multiplying by the reciprocal of the divisor. With a divisor, we also have a multiplier. So the rules for the Distributive Property have not really changed.
Let us take a look at the expression $(27 + 12 - 21) \div 3$. We have two options for solving it:
- First, we can evaluate the parentheses, which equals $18$. Then we divide by $3$ and get $6$.
- On the other hand, we can divide each term inside the parentheses separately, paying attention to each operator. That gives us $27 \div 3 + 12 \div 3 - 21 \div 3 = 9 + 4 - 7$. In the end, we get $6$, too.

Variables
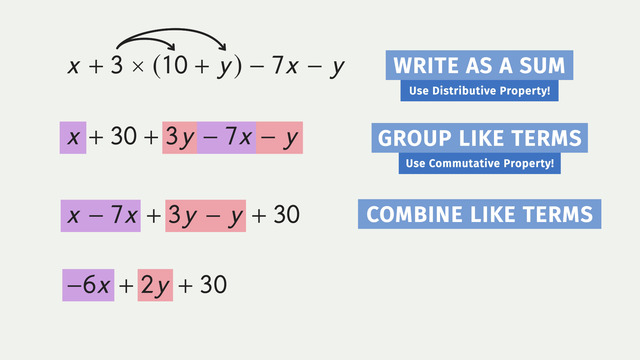
Simplifying Variable Expressions
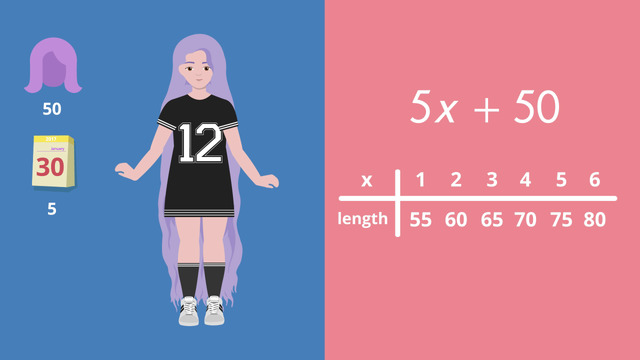
Evaluating Expressions

How to do Order of Operations?

Distributive Property
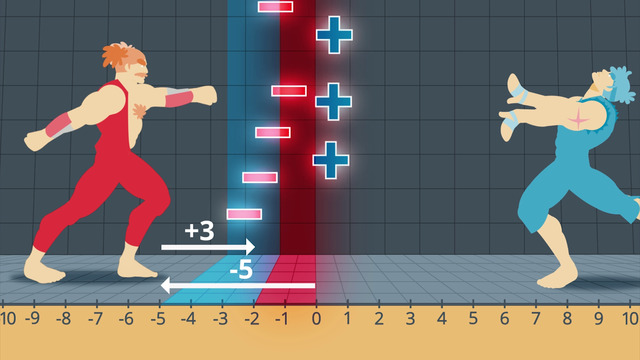
Adding Integers
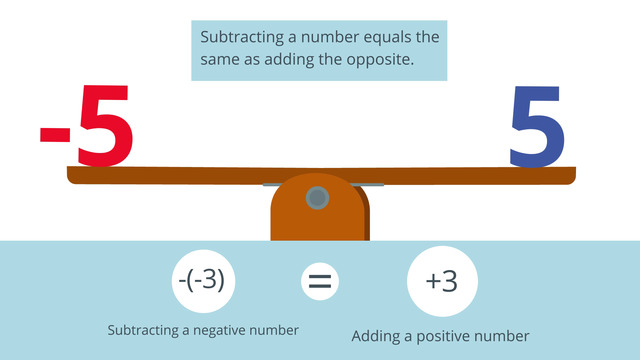
Subtracting Integers

Multiplying and Dividing Integers
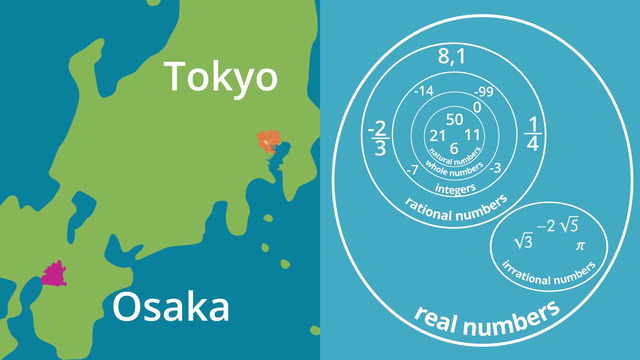
Types of Numbers
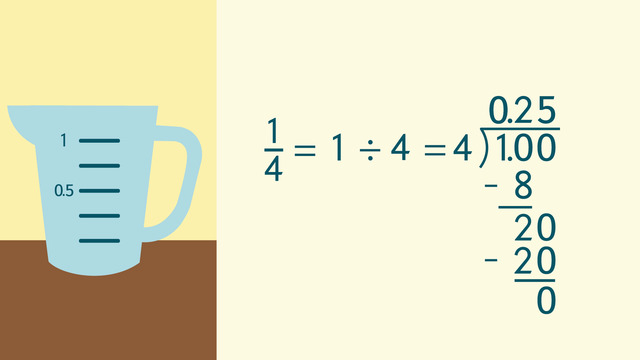
Transforming Terminating Decimals to Fractions and Vice Versa

Transforming Simple Repeating Decimals to Fractions and Vice Versa
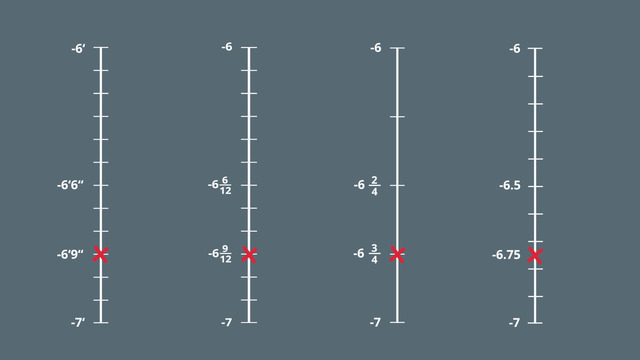
Rational Numbers on the Number Line
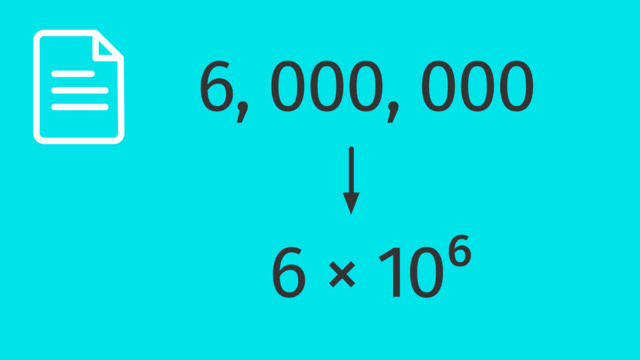
Standard and Scientific Notation

Using Operations with Scientific Notations











