Subtracting Integers
Basics on the topic Subtracting Integers
Subtracting Integers – Definition
Subtracting integers might seem tricky at first, but it's a fundamental skill in mathematics. Integers are whole numbers that can be positive, negative, or zero. In our daily lives, we use the concept of subtracting integers when we calculate differences in temperatures, account balances, elevations, and more.
Subtracting Integers refers to the process of finding the difference between two integers. When subtracting integers, we are essentially adding the opposite (additive inverse) of the number being subtracted.
Understanding Subtracting Integers – Explanation
To understand subtracting integers, let's focus on the concept of adding the additive inverse.
The additive inverse of a number is the number that, when added to the original number, results in zero. For example, the additive inverse of $3$ is $-3$, and vice versa.
When we subtract an integer, we are actually adding its additive inverse. For example, in the subtraction $2 - 3$, we are really doing $2 + (-3)$. This approach simplifies the process of subtracting integers, especially when dealing with negative numbers.
Being familiar with integers and their opposites is incredibly valuable when it comes to operations of integers.
Subtracting Integers – Step-by-Step Process
| Step Number | Directions | Example |
|---|---|---|
| Step 1 | The first number stays the same. Change the subtraction sign to addition. |  |
| Step 2 | Change the sign of the number being subtracted to its additive inverse (or opposite sign). |  |
| Step 3 | Add the numbers together using strategies you know for adding. |  |
Let’s work through another example step by step.
Calculate $-5 - 3$.
- Step 1: Change the subtraction to addition: $-5 + 3$.
- Step 2: Change the sign of the second number to its opposite sign: $-5 + (-3)$.
- Step 3: Add the numbers together! $-5 + (-3) = -8$.
As you can tell, subtracting integers essentially turns any problem into an addition problem which is why it is important to have a strong understanding of adding integers.
Subtracting Integers – Examples
Try these examples on your own using the steps presented above.
Subtracting Integers – Summary
Key Learnings from this Text:
- Subtracting an integer is the same as adding its additive inverse.
- The additive inverse of a number is what you add to the original number to get zero.
- This method simplifies the process of subtracting integers, particularly negative numbers.
Subtracting Integers – Frequently Asked Questions
Transcript Subtracting Integers
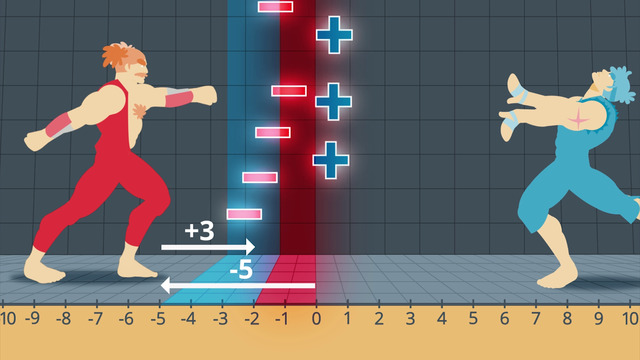
Have you ever tried to balance a seesaw? I will explain to you what this has to do with subtracting integers. How can we bring this seesaw into balance?
We need to put a value here, on the right side, to get it in balance. In this case, a positive 3 balances the seesaw. We can write this as an addition: + 3. Let us go back to the first situation. Can you imagine another way to bring this seesaw in balance? Right!
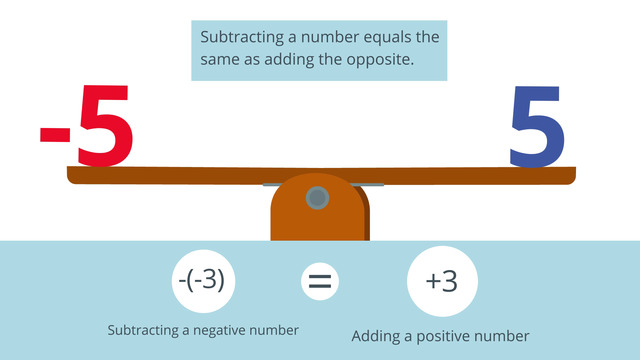
Subtracting a Negative Number – Example 1
Another way is to take something away on the left side. In this case, negative 3 can be taken away from the left side. We can express this mathematically as minus a negative 3. As you can see the seesaw is in balance again, which is the same as in the situation before. Taking negative 3 away equals adding positive 3. Remember: Subtracting a number equals the same as adding the opposite. So, subtracting a negative 3 is the same as adding a positive 3.
Subtracting a Negative Number – Example 2
Now let's look at a different example. Find the value of negative 2 minus negative 4 minus 3. Instead of minus 3 you can also write minus positive 3. You have learned that subtracting a number equals the same as adding the opposite number. In our example minus negative four becomes plus positive four and instead of minus positive three you can write plus negative three. Ok, let's calculate from left to right: −2 + 4 = 2 and 2 + −3 = −1.
You have learned that subtracting a negative number is the same as adding the opposite number. But might this knowledge help our little friend in his situation? Maybe...
Subtracting Integers exercise
-
Find the integer that brings the seesaw back into balance.
HintsWhich of the numbers on the left side of the seesaw do not have an opposite pair on the right side?
The absolute value of the left side is $16$. Remember, absolute value is the value of a number without the sign.
SolutionIf we look at the left side of the equation, we see the integers $-7$ and $-9$. On the right-hand side, we have a $+7$.
The opposite of $-7$ is $+7$, since the absolute value of these numbers is equal.
Since there's also a $-9$ on the left-hand side, we can balance the seesaw by putting a number that is equal to the opposite of $-9$ on the right-hand side.
In this case, a $+9$ would balance the equation (seesaw).
-
Describe how to solve the equation $-2 - (-4) -3$.
HintsSubtracting a negative number is the same as adding a positive number.
Subtracting a positive number is the same as adding a negative number.
Remember to use the proper Order of Operations. (PEMDAS)
SolutionLet's start with the given equation:
- $(-2)-(-4)-3$
- $(-2)+(+4)-3$
- $(-2)+4-3$
- $(-2)+4+(-3)$
- $(-2)+4=2$
- $2+(-3)=-1$
-
Calculate the temperature change by subtracting integers.
HintsSubtracting a negative number is the same as adding a positive number.
To calculate the difference of two numbers they have to be subtracted.
The result is always a positive value.
SolutionThe temperature on day one is $4$ degrees. The temperature on day two is $-3$ degrees. In order to find the difference in the temperatures, you should subtract $-3$ from $4$. We can write this equation as follows:
$4-(-3)$
Since we know that subtracting a negative number is the same as adding a positive number, we can rewrite the equation and solve:
$4+3=7$
So the temperature changed by $7$ degrees.
$\begin{array}{rcl} 4 - (-3) & = & 4 + 3\\ & = & 7 \end{array}$
-
Evaluate John's final score by adding and subtracting integers.
HintsAdding a negative number is the same as subtracting a positive number.
Subtracting a negative number is the same as adding a positive number.
To solve mathematical expressions, follow the rules of PEMDAS.
SolutionLet's look at John's throws:
John's first throw results in a positive $2$. On John's second throw, he hits $(+/-)$ and a $3$ on his third throw. The fourth throw is a $(-/-)$, and he hits a $6$ on his final throw.
That will leave us with $2+(-3)-(-6)$. Using the Order of Operations (PEMDAS), we can now solve from left to right.
$\begin{array}{rcl} 2 + (-3) -(-6) & = & ~~~2 + (-3) + 6\\ & = & -1 + 6 \\ & = & ~~~5 \end{array}$
John's final score is $5$.
-
Identify the correct statements regarding subtracting integers.
HintsTry comparing different combinations of adding and subtracting positive and negative numbers.
SolutionThe statements are provided below:
- 'Subtracting a positive number is the same as adding the negative of the number.' This definition is true. $4-(-4)$ is the same as $4+4$. Try it out!
- 'Adding two numbers always results in a larger number.' This statement is false. If just one of the numbers is negative, this answer is not true. When we add $4+(-3)$, the answer, $1$, is smaller than $4$.
- 'Subtracting two numbers always results in a larger number.' This statement is also false. While this is true if just one of the numbers is negative, this answer is not true. When we add $6-2$, the answer, $4$, is smaller than $6$.
- 'Subtracting a negative number is the same as subtracting a positive number.' This answer is false. In the equation $8-(-1)$, subtracting a positive number will result in $7$, which is incorrect. When we add $8-(-1)$, $9$ is the correct answer.
-
Identify the equivalent expressions.
HintsAdding two positive integers always yields a positive sum while adding two negative integers always yields a negative sum.
However, subtracting a negative number is like adding the same number.
SolutionLet's simplify the signs to find an equivalent equation.
- If we have an equation $7 - (-1) + 3$, and we know that subtracting a negative number is the same as adding a positive number, we can simplify this equation to $7 + 1 + 3$.
- For the equation $-7 + (-1) + (-3)$, we know that adding a negative number is the same as subtracting a positive number. So now we can simplify this equation to $-7 - 1 - 3$.
- Given the equation $7 - 1 - (-3)$, if we remember that subtracting a negative number is the same as adding a positive number, we can simplify this equation to $-7 - 1 + 3$.
- Lastly, for the equation $-7 - (-1) + 3$, we know that subtracting a negative number is the same as adding a positive number, so we can simplify this equation to $-7 + 1 + 3$.

Variables
Simplifying Variable Expressions
Evaluating Expressions

How to do Order of Operations?

Distributive Property
Adding Integers
Subtracting Integers

Multiplying and Dividing Integers
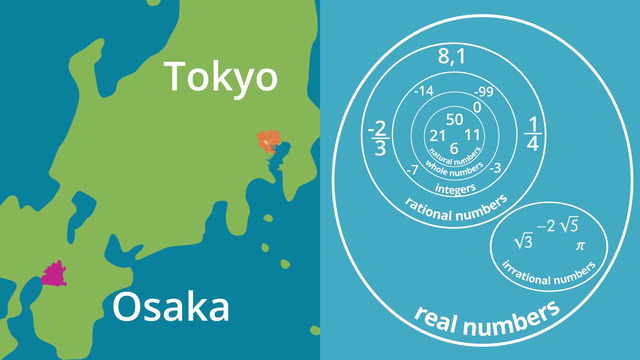
Types of Numbers
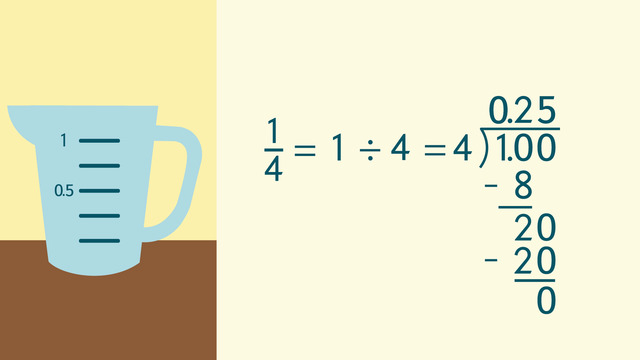
Transforming Terminating Decimals to Fractions and Vice Versa

Transforming Simple Repeating Decimals to Fractions and Vice Versa
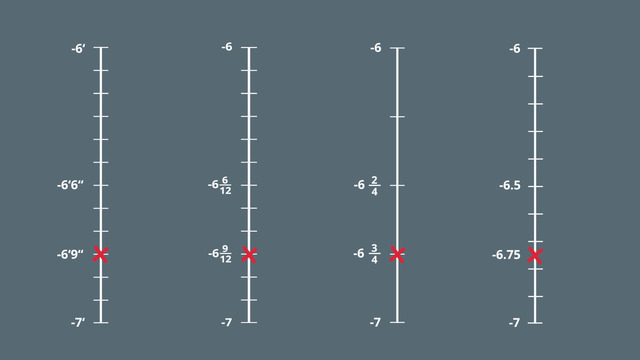
Rational Numbers on the Number Line
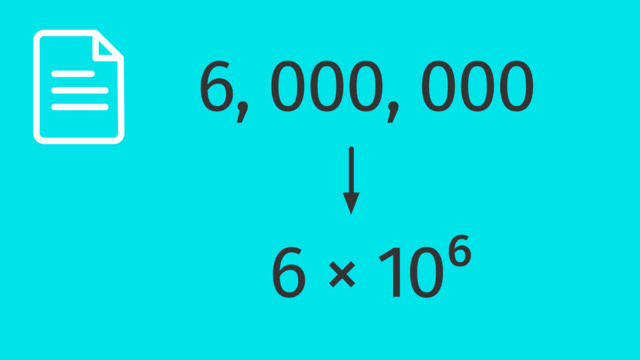
Standard and Scientific Notation

Using Operations with Scientific Notations














hi
hi