Determining Equivalent Ratios

Basics on the topic Determining Equivalent Ratios
After this lesson, you will be able to identify equivalent ratios and find their missing values using a ratio table.
The lesson begins by reviewing the definition of ratios and the three ways of how to write them. It leads you to learn how to complete a table of equivalent ratios. It concludes by teaching you how to determine other values of equivalent ratios using the ratio table.
Learn about determining equivalent ratios by finding out how Junior Moranis restores the size of his shrunk sister.
This video includes key concepts, notation, and vocabulary such as ratio (a relationship between two non-zero quantities or numbers); equivalent ratios (a set of paired quantities or ratios that have the same or equivalent value); and ratio table (which is a table that lists ratios which has the same or equivalent value).
Before watching this video, you should already be familiar with fractions, especially equivalent fractions, fractions written in simplest form, tables, and ratios.
After watching this video, you will be prepared to fill in more complicated ratio tables that use mathematical properties, such as inverses, and to convert ratio tables into double number lines.
Common Core Standard(s) in focus: 6.RP.A.3.A A video intended for math students in the 6th grade Recommended for students who are 11 - 12 years old
Transcript Determining Equivalent Ratios
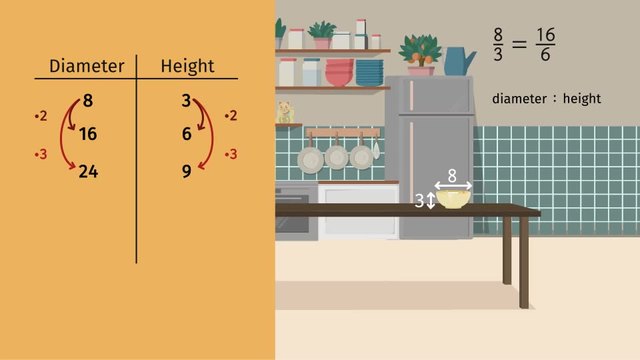
While playing around with some weird science stuff in his dad’s home laboratory, Junior Moranis sort of...might have...maybe...shrunk his little sister. With his mom coming home soon, Junior Moranis notices a growth ray conveniently hanging on the wall . But before he fires it at his sister, he decides to try it out to determine if it maintains equivalent ratios. You already know that a ratio is the relationship between two quantities, which we can call 'a' and 'b'. And you also know there are three ways to write a ratio: as a fraction with a colon or as numbers separated by the word “to.” We can also put this information in a table of equivalent ratios. Let's take this cereal bowl as an example. It has a diameter of 8 inches and a height of 3 inches. So we can say the diameter to height ratio is 8 to 3. If the growth ray maintains equivalent ratios, no matter how big we increase the size of the cereal bowl, this ratio will stay the same. Let's put the cereal bowl dimensions in a table so we can see which other ratios are equivalent to 8 to 3. Just like with equivalent fractions, equivalent ratios can be found by multiplying both numbers by the same value. So, if we double the diameter and height, we get a ratio of 16 to 6. We know the ratios are consistent because 8 thirds and 16 sixths are equivalent fractions. Let’s fill in the rest of the table with a few more ratios. Multiply both 8 and 3 by 3 to get the equivalent ratio 24 to 9. Multiply by 4 to get 32 to 12, and so on. No matter how big we make the cereal bowl, the diameter to height ratio can be simplified to 8 to 3. We can also use equivalent ratios to find missing information. This hair dryer has a 9-inch nozzle and a 5-inch handle. If we use the growth ray to increase the size of the nozzle to 36-inches, how long will the handle be? Just like before, we can use equivalent fractions to fill in the ratio table. Multiply both 9 and 5 by a value of 2 to get the equivalent ratio 18 to 10, then multiply 9 and 5 by a value of 3 to get the equivalent ratio of 27 to 15. We can fill in the final row of the table by multiplying the original ratio of 9 to 5 by a value of 4, which gives us the equivalent ratio of 36 to 20. If we look here, we can see that when the nozzle is 36-inches long, the handle will be 20-inches long. This humongous hair dryer is much bigger than before, but the ratio of the nozzle to the handle has stayed the same. To review... A ratio is a comparison of two different quantities. A table can be used to list pairs of numbers that form equivalent ratios. You can tell that two ratios are equivalent if they also are equal fractions. You can use equivalent ratios to solve real-world (and not so real-world) problems. Now that Junior Moranis is sure the growth ray works, he’s ready to try it out on his sister. Oh no! Look out! It may be fun to play around with math, but that's why you don’t mess with science.
Determining Equivalent Ratios exercise
-
Explain what happens if we enlarge the bowl using ratios and ratio tables.
HintsA ratio is reduced when there are no common factors. Here are some examples:
- The ratio $4:5$ is reduced because there are no whole numbers other than $1$ that divide into both $4$ and $5$.
- The ratio $7:1$ is reduced.
- The ratio $30:12$ is not reduced because we can divide both values by $6$ to get $5:2$.
- $5:2$ is reduced.
We can multiply the values of a ratio to get an equivalent ratio. Here are some examples:
- Given the ratio $4:7$, we can multiply by $3$ to get $12:21$. $12:21$ is equivalent to $4:7$ because $\frac{12}{21}=\frac47$.
- Given the ratio $1:3$ we can multiply by $8$ to get the equivalent ratio $8:24$.
- Given the ratio $4:9$ we can multiply by $\frac12$ to get the equivalent ratio $2:4.5$.
Given two ratios, we can compare them by reducing:
- The ratios $6:10$ and $3:5$ are equivalent. Dividing the numerator and denominator of $\frac{6}{10}$ by $2$ gives us $\frac35$.
- The ratios $45:150$ and $6:20$ are equivalent. $\frac{45}{150}$ and $\frac{6}{20}$ both reduce to $\frac{3}{10}$.
- The ratios $21:49$ and $9:24$ are not equivalent. $\frac{21}{49}=\frac37$ while $\frac{9}{24}=\frac38$.
SolutionThe reduced ratio of the diameter of the bowl to its height can be written $8$ to $3$, $8:3$, or $\frac83$. If we enlarge the bowl by a factor of $2$, the ratio of diameter to height becomes $16:6$. This ratio is equivalent to $8:3$ because $\frac{16}{6}=\frac83$. In fact, all of the ratios in the table are equivalent because all of them reduce to $8:3$. If we multiply $8$ and $3$ by $10$, we get the ratio $80:30$, which is equivalent to $8:3$.
-
Define various key words related to ratios.
HintsA ratio can be written several ways. For example, the ratio $10:3$ can be written:
- $10:3$
- $10 ~\text{to}~ 3$
- $\frac{10}{3}$
Ratios must have positive numbers. Zero and negative numbers are excluded. The number $1$ is not excluded.
- All the ratios in a ratio table are equivalent, otherwise we don't call it a ratio table.
- A ratio in a table may already be completely reduced, in which case it cannot be reduced further.
If we reverse the order of the ratio, we have changed its meaning. For example:
- The ratio of the length of a car to its height is $17~\text{ft}:5~\text{ft}$.
- A ratio of length to height of $5:17$ is very different. That would be a very tall car!
- However, if we are careful to redefine $17:5$ as the ratio of height to length, then we are still talking about the original car.
We are not required to write ratios with the largest number first, or to put them in a particular order. But when we choose an order for a ratio, we cannot change the order without changing the meaning. Here's the one exception to that rule:
- The ratio $1:1$ is the same when it's reversed.
Solution- Ratios are not always written with the largest number first.
- Ratios in a ratio table are always equivalent.
- We cannot create equivalent ratios by multiplying each value in the ratio by a different number.
- Multiplying the values of a ratio by the same number results in an equivalent ratio.
- Enlarging an object by multiplying its dimensions by the same value preserves the ratio of the dimensions.
- All of the ratios in a ratio table are equivalent fractions.
- We can find equivalent ratios by multiplying by a constant.
- There are several ways to write a ratio.
- A ratio table cannot feature negative numbers.
- A ratio can have $1$ as one of its values.
- A ratio cannot have $0$ as one of its values.
- Not every ratio can be reduced (some are already reduced).
- If a ratio is written in reverse order, it is often a different ratio. For example: $4:5\neq5:4$.
- The ratio $1:1$ written in reverse order is the same as the original ratio.
-
Solve the real-world problem using a ratio table.
HintsTo find an equivalent ratio from a given ratio, we can always reduce that ratio, then multiply by a factor to get the desired ratio. Here are some examples:
$\begin{array}{c|c} \text{Sugar} & \text{Cinnamon}\\ \hline ? & 7\\ 6 & 14\\ 30 & ?\\ \end{array}$
- In the above table, we can see that the given ratio $6:14$ can be reduced by dividing by $2$.
- The resulting ratio is $3:7$.
- Multiplying by $10$ gives us $30:70$.
From a given ratio, we can always begin by trying to reduce it to a simpler ratio, then multiplying by a specific factor to get a desired ratio. Finding the correct factor may require division. Here's an example:
$\begin{array}{c|c} \text{Apples} & \text{Bananas}\\ \hline 5 & ?\\ 15 & 33\\ 490 & ? \end{array}$
- From $15:33$, our reduced ratio is $5:11$.
- $490$ divided by $5$ gives us $98$.
- So we multiply $11$ by $98$ to get $1078$.
- The last ratio in the table is $490:1078$.
Given a ratio, we may not need to reduce to create the desired equivalent ratio:
$\begin{array}{c|c} \text{Shirts} & \text{Pants}\\ \hline 6 & 15\\ 12 & ?\\ \end{array}$
- Although the ratio $6:15$ is not reduced in the above example, we can see that $12$ is $6$ times $2$.
- So we multiply $15$ by $2$ to get $30$.
- The last ratio in the table is $12:30$.
SolutionThe ideal ratio of Strawberry bushes to Blueberry bushes in a garden is represented by the incomplete ratio table at right. The smallest garden is represented by the ratio $3:5$. If we want a bigger garden, we must increase the values of the ratio. If we want $12$ Strawberry bushes, we multiply the reduced ratio of $3:5$ by $4$. The resulting ratio will be $12:20$.
Malala keeps track of her hours of Homework and Basketball practice per week. The ratio table shows the ideal relationship between the two. Malala wants to play Basketball for $18$ hours this week. How much time should she dedicate to Homework? The given ratio $4:6$ can be reduced to $2:3$. Multiplying this ratio by $6$ gives us $12:18$. So if Malala wants to play basketball for $18$ hours, she should do math homework for $12$ hours!
The ideal ratio of Blue candies to Red candies is shown in the ratio table. Although the number of candies can increase, the ratio between Blue and Red stays the same. How many Blue candies are needed if we have $150$ Red candies? We don't always have to reduce a given ratio. The given ratio of $60:75$ can be multiplied directly by $2$ to get $120:150$. That means we need $120$ Blue candies to preserve the ideal ratio.
-
Identify which ratios are equivalent to the ratios displayed in the central elements.
HintsTo compare two ratios, we can reduce them:
$\begin{array}{c|c|c} \text{1st Fraction} & \text{2nd Fraction} & \text{Equivalent?}\\ \hline\ &&\\ \frac{6}{14}=\frac37 & \frac{15}{35}=\frac{3}{7} & \text{yes}\\ &&\\ \frac{2}{30}=\frac{1}{15} & \frac{30}{2}=\frac{15}{1} & \text{no}\\ &&\\ \frac{10}{12}=\frac56 & \frac{18}{24}=\frac34 & \text{no} \end{array}$
We can multiply fractions by factors to create equivalent ratios. The ratio $A:B$ below is $3:7$. Multiplying this ratio by the given factors results in equivalent ratios as shown:
$\begin{array}{r|c|c} \text{Multiply} & \text{A} & \text{B}\\ \hline & 3 & 7\\ \times2 & 6 & 14\\ \times3 & 9 & 21\\ \times5 & 15 &35\\ \times10 & 30 & 70 \end{array}$
Sometimes we can go directly from a given ratio that isn't reduced, to another equivalent ratio, as in the example below:
$\begin{array}{c|c} \text{A} & \text{B}\\ \hline 40 & 25\\ ? & 150\\ \end{array}$
- $25\times6=150$.
- So we can compute $40\times6=240$.
- The resulting ratio is $240:150$.
SolutionOne way to simplify this problem is to reduce the ratios for each of the central ratios first. Then focus on those reduced ratios as you are matching the remaining ratios.
$\begin{array}{r|r|r|r} \text{Central Ratio} \rightarrow & 5:12 & 12:8=3:2 & 8:16=1:2\\ \hline & 15:36=5:12 & 6:4=3:2 & 4:8=1:2\\ & 10:24=5:12 & 21:14=3:2 & 200:400=1:2 \\ & 50:120=5:12 & &\\ \end{array}$
-
Decide which fractions are equivalent to each other.
HintsTo reduce a fraction:
- Look for a common factor in the numerator and denominator.
- Divide the numerator and denominator by the common factor.
- Repeat this process until there are no more common factors.
$\begin{array}{c|c|c} \text{Fraction} & \text{Common Factor} & \text{Reduced Result}\\ \hline\ &&\\ \frac{6}{15} & 3 & \frac25\\ &&\\ \frac{21}{42} & 21 & \frac12\\ &&\\ \frac{40}{32} & 8 & \frac54 \end{array}$
Two fractions can be equivalent even though neither of them is reduced. Reduce each fraction and compare their results to determine if they are equivalent. Here are some examples:
\begin{array}{c|c|c} \text{1st Fraction} & \text{2nd Fraction} & \text{Equivalent?}\\ \hline\ &&\\ \frac{12}{21}=\frac47 & \frac{24}{42}=\frac{4}{7} & \text{yes}\\ &&\\ \frac{12}{15}=\frac45 & \frac{15}{18}=\frac56 & \text{no}\\ &&\\ \frac{10}{30}=\frac13 & \frac{30}{60}=\frac12 & \text{no} \end{array}
A whole number can be written as a fraction. $9=\frac91$.
SolutionHere is a table showing reducing to find the equivalent fractions.
-
Complete the Ratio Tables.
HintsWe can reduce a given ratio by dividing by a common factor of each value:
$\begin{array}{r|c|l} \text{Given Ratio} & \text{Common Factor} & \text{Reduced Ratio}\\ \hline 22:11 & \div11= & 2:1\\ 39:26 & \div13= & 3:2\\ 50:75 & \div25= & 2:3 \end{array}$
We can find equivalent ratios by multiplying to get the desired result.
$\begin{array}{r|c|c} \text{Multiply} & \text{A} & \text{B}\\ \hline & 5 & 3\\ \times2 & 10 & 6\\ \times3 & 15 & 9\\ \times5 & 25 &15\\ \times10 & 50 & 30 \end{array}$
Sometimes it can be helpful to divide in order to determine what to multiply the reduced ratio by:
$\begin{array}{c|c} \text{A} & \text{B}\\ \hline 5 & 17\\ ? & 102\\ \end{array}$
- $\frac{102}{17}=6$.
- This means we multiply $5\times6=30$ to complete the second ratio.
- The second ratio is $30:102$.
Solution$\begin{array}{c|c|c} 3:2 & 7:4 & 8:9\\ 15:10 & 21:12 & 40:45\\ 27:18 & 35:20 & 32:36\\ \end{array}$

Determining Equivalent Ratios
Ratio Tables - Additive and Multiplicative Structure

Comparing Ratios Using Ratio Tables

From Ratio Tables to Double Number Lines

From Ratio Tables to Equations

Plotting Ratios on the Coordinate Plane

Reviewing Representations of Ratios
What are Congruence and Similarity?











