Multiplying Tens, Hundreds and Thousands


Basics on the topic Multiplying Tens, Hundreds and Thousands
Multi Digit Multiplication – Introduction
Today is a big day: You are responsible for helping out with the candy sale at school! In preparation, you are given one box of 4 x 10 chocolates, one box of 9 x 100 gummy bears and one box containing 5 x 1,000 marshmallows. Can you calculate how many pieces of each candy you have in total? In order to do that, you need to practice multiplying by 10, multiplying by 100, and multiplying by 1,000. But how exactly do we multiply by 10, 100, and 1,000 and what happens to the digits when multiplying by 10, 100, and 1,000? You can find out the answers to these questions in the following text.
Multi Digit Multiplication – Steps
Below, you will see there are three easy steps you can take to find the product.
| Step # | What to do |
|---|---|
| 1 | Ignore the zeros. |
| 2 | Find the product of the remaining numbers. |
| 3 | Count the number of zeros and add or annex them to the product. |
Now that you are familiar with the theory, let’s take a look at some examples of multiplication by 10, 100, and 1,000.
Multi Digit Multiplication – Examples
What happens to the digits when you multiply by 10? Here, we are multiplying four times ten. When multiplying by 10, the first step is to ignore the zeros. The second step is to find the product of the remaining numbers four and one, which is four. The third step is to add or annex a zero to our answer. When you multiply by 10, you add just one zero to the product because there is only one zero in the problem. The product of four times ten is forty.
Let’s practice multiplying by 100 next.
What happens to the digits when you multiply by 100? To find the product of nine times one hundred, we first ignore the zeros. Next, we find the product of the remaining numbers nine and one, which is nine. Last, we add or annex the zeros to our answer. When you multiply by 100, you add two zeros to the product because there are two zeros in the problem. The product of nine times one hundred is nine hundred:

Now, let’s practice multiplying by 1,000.
What do you think happens to the digits when you multiply by 1,000? To find the product of five times one thousand, we first ignore the zeros again. In the next step, we find the product of the remaining numbers five and one, which is five. Last, we add or annex the zeros to our answer. When you multiply by 1,000, you add three zeros to the product because there are three zeros in the problem. Therefore, the product of five times one thousand is five thousand.
Multi Digit Multiplication – Summary
Remember the three steps whenever multiplying by 10, 100, and 1,000. The solution to find the product always follows the same approach.
| Step # | What to do |
|---|---|
| 1 | Ignore the zeros. |
| 2 | Find the product of the remaining numbers. |
| 3 | Count the number of zeros and add or annex them to the product. |
Now that you know how much of each candy you can sell in total, you will be a master salesperson at your school! Would you like to practice some more? Next to the text you can also find multiplying by 10, 100 and 1,000 worksheets and exercises.
Transcript Multiplying Tens, Hundreds and Thousands
Mr. Squeaks is creating a surprise for Imani behind the curtain. He needs some help calculating how many nails, bolts, and nuts he has. In order to know how many of each are in the boxes we need to assist with multiplying tens, hundreds, and thousands. When you multiply a whole number by tens, hundreds, and thousands the first step is to ignore the zeros. The second step is to find the product of the remaining numbers. The third step is to count the number of zeros and add or annex them to the product. For example, in the problem four times ten, we would ignore the zero and find the product of four times one. Four times one equals four. We notice here that there is only one zero in the problem four times ten, so we add, or annex, one zero to the answer. The product of four times ten is forty. Now that you know the steps needed to multiply by tens, hundreds, and thousands. Let's see how many bolts are in this box for Mr. Squeaks. We need to find the product of nine times one hundred. What is our first step? The first step is to ignore the zeros. What is the second step? The second step is to find the product of the remaining numbers. Nine times one equals nine. Now, what do we do with the zeros that we ignored in the beginning? The third step is to count the number of zeros and annex them to the product. Since there are two zeros in the number one hundred, we annex two zeros to our answer making the product of nine times one hundred, nine hundred. Let's help Mr. Squeaks calculate how many nuts he has in this box. We need to find the product of five times one thousand. What is our first step? The first step is to ignore the zeros. What is the second step? The second step is to find the product of the remaining numbers. Five times one equals five. Now, what do we do with the zeros? The third step is to count the number of zeros and annex them to the product. Since there are three zeros in the number one thousand, we annex three zeros to our answer making the product of five times one thousand, five thousand. Let's calculate how many bolts Mr. Squeaks has in his last box. We need to find the product of six times two thousand. What is our first step? The first step is to ignore the zeros. What is the second step? The second step is to find the product of the remaining numbers. Six times two equals twelve. Now, what do we do with the zeros? The third step is to count the number of zeros and annex them to the product. Since there are three zeros in the number two thousand, we annex three zeros to our answer making the product of six times two thousand, twelve thousand. Remember, when you multiply a whole number by tens, hundreds, and thousands the first step is to ignore the zeros. The second step is to find the product of the remaining numbers. The third step is to count the number of zeros and add or annex them to the product. Phew! Mr. Squeaks has a lot of supplies! I wonder what he made for Imani...
Multiplying Tens, Hundreds and Thousands exercise
-
How many screws are in the box?
HintsFirst, ignore the zeros.
Second, find the product of the remaining numbers.
Finally, count the number of remaining zeros and join them back onto the product.
SolutionIgnore the zeros and multiply: 7 x 1 = 7.
Count the zeros: 700 has two zeros.
Join the zeros back on: 700 x 1 = 700.
-
Solve each multiplication expression.
HintsFor each problem, first ignore the zeros.
Second, find the product of the remaining numbers.
Finally, count the number of remaining zeros and join them back onto the product.
Solution8 x 10
- First, ignore the zeros and multiply: 8 x 1 = 8.
- Count the zeros: 10 has one zero.
- Join the zeros back on: 8 x 10 = 80.
8 x 1,000
- First, ignore the zeros and multiply: 8 x 1 = 8.
- Count the zeros: 1,000 has three zeros.
- Join the zeros back on: 8 x 1,000 = 8,000.
3 x 2,000
- First, ignore the zeros and multiply: 3 x 2 = 6.
- Count the zeros: 2,000 has three zeros.
- Join the zeros back on: 3 x 2,000 = 6,000.
3 x 30
- First, ignore the zeros and multiply: 3 x 3 = 9.
- Count the zeros: 30 has one zero.
- Join the zeros back on: 3 x 30 = 90.
-
Which equations are solved correctly?
HintsFirst, ignore the zeros.
Second, find the product of the remaining numbers.
Finally, count the number of remaining zeros and join them back onto the product.
Solution4 x 30
- First, ignore the zeros and multiply: 4 x 3 = 12.
- Count the zeros: 30 has one zero.
- Join the zeros back on: 4 x 30 = 120. This is correct.
2 x 5,000
- First, ignore the zeros and multiply: 2 x 5 = 10.
- Count the zeros: 5,000 has three zeros.
- Join the zeros back on: 2 x 5,000 = 10,000, not 1,000. This is incorrect.
7 x 60
- First, ignore the zeros and multiply: 7 x 6 = 42.
- Count the zeros: 60 has one zero.
- Join the zeros back on: 7 x 60 = 420. This is correct.
5 x 7,000
- First, ignore the zeros and multiply: 5 x 7 = 35.
- Count the zeros: 7,000 has three zeros.
- Join the zeros back on: 5 x 7,000 = 35,000. This is correct.
9 x 800
- First, ignore the zeros and multiply: 9 x 8 = 72.
- Count the zeros: 800 has two zeros.
- Join the zeros back on: 9 x 800 = 7,200, not 45,000. This is incorrect.
-
Multiply each expression.
HintsFirst, ignore the zeros in both numbers.
Second, find the product of the remaining numbers.
Finally, count the number of zeros from both numbers and join the total number of zeros back onto the product.
SolutionPick a problem to start with: 300 x 30.
- First, ignore the zeros and multiply: 3 x 3 = 9.
- Count the zeros: 300 has two zeros and 30 has one zero.
- So, there are a total of three zeros.
_____________________________________________________________________________________
60 x 40:
- 6 x 4 = 24 and there are a total of 2 zeros, so 60 x 40 = 2,400.
12 x 500:
- 12 x 5 = 60 and there are a total of 2 zeros, so 12 x 500 = 6,000.
2 x 2,400:
- 2 x 24 = 48 and there are a total of 2 zeros, so 2 x 2,400 = 4,800.
100 x 600:
- 1 x 6 is 6 and there are a total of 4 zeros, so 100 x 600 = 60,000.
30 x 30:
- 3 x 3 is 9, and there are a total of 2 zeros, so 30 x 30 = 900.
-
Solve each multiplication equation.
HintsFirst, ignore the zeros.
Second, find the product of the remaining numbers.
Finally, count the number of remaining zeros and join them back onto the product.
Solution6 x 10
- First, ignore the zeros and multiply: 6 x 1 = 6.
- Count the zeros: 10 has one zero.
- Join the zeros back on: 6 x 10 = 60.
9 x 2,000
- First, ignore the zeros and multiply: 9 x 2 = 18.
- Count the zeros: 2,000 has three zeros.
- Join the zeros back on: 9 x 2,000 = 18,000.
3 x 700
- First, ignore the zeros and multiply: 3 x 7 = 14.
- Count the zeros: 700 has two zeros.
- Join the zeros back on: 3 x 700 = 2,100.
2 x 500
- First, ignore the zeros and multiply: 2 x 5 = 10.
- Count the zeros: 500 has two zeros.
- Join the zeros back on: 2 x 500 = 1,000.
-
Put the values in order.
HintsFind the value of each expression and then sort them from least to greatest.
First, ignore the zeros and then find the product of the remaining numbers.
Finally, count the number of remaining zeros and join them back onto the product.
SolutionFirst find the value of each expression, then put them in order from least to greatest:
4 x 10
- First, ignore the zeros and multiply: 4 x 1 = 4.
- Count the zeros: 10 has one zero.
- Join the zeros back on: 4 x 10 = 40.
8 x 50
- First, ignore the zeros and multiply: 8 x 5 = 40.
- Count the zeros: 50 has one zero.
- Join the zeros back on: 8 x 50 = 400.
2 x 300
- First, ignore the zeros and multiply: 2 x 3 = 6.
- Count the zeros: 300 has two zeros.
- Join the zeros back on: 2 x 300 = 600.
7 x 90
- First, ignore the zeros and multiply: 7 x 9 = 63.
- Count the zeros: 90 has one zero.
- Join the zeros back on: 7 x 90 = 630.
5 x 1,000
- First, ignore the zeros and multiply: 5 x 1 = 5,000.
- Count the zeros: 1,000 has three zeros.
- Join the zeros back on: 5 x 1,000 = 5,000.
9 x 600
- First, ignore the zeros and multiply: 9 x 6 = 54.
- Count the zeros: 600 has two zeros.
- Join the zeros back on: 9 x 600 = 5,400.
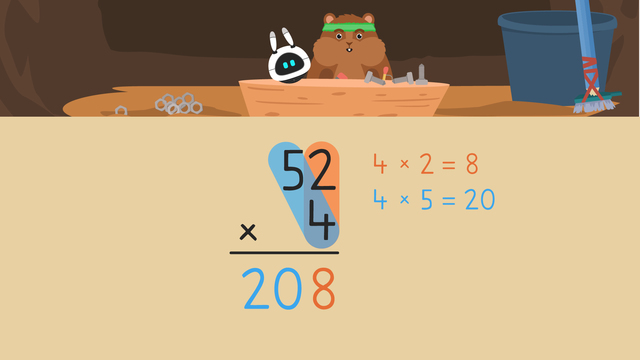
Multiplying up to Three Digits Using the Standard Algorithm
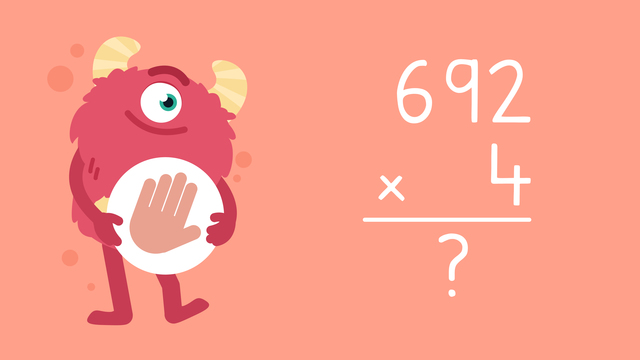
Multiplying up to Three Digits Using the Standard Algorithm—Let's Practice!

Multiplying Two-Digit Numbers by Two-Digit Numbers
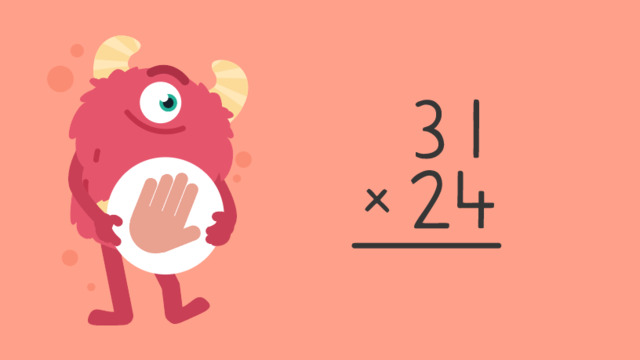
Multiplying Two-digit Numbers by Two-digit Numbers — Let's Practice!
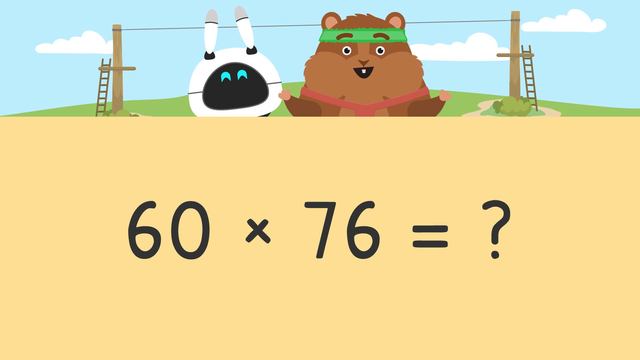
Multiplying 2-Digit Numbers by Multiplies of 10

Multiplying Tens, Hundreds and Thousands

Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model
Multiplying Two-Digit Numbers by Two-Digit Numbers Using an Area Model—Let's Practice













