Adding Tenths and Hundredths — Let's Practice!

Basics on the topic Adding Tenths and Hundredths — Let's Practice!
Adding Tenths and Hundredths – Introduction
We're diving into the world of adding tenths and hundredths. These problems require us to work with fractions that have different denominators. As we embark on this mathematical journey, remember that solving these problems is like unraveling a mystery, and you're the detective!
What Are Tenths and Hundredths?
Tenths and hundredths are fractions where the denominator, the bottom number, is 10 or 100. To add fractions with different denominators, we need to convert them to have the same denominator. This process involves multiplying or dividing the numerators and denominators to create equivalent fractions.
Here are the steps to tackle adding tenths and hundredths:
| Step # | Action |
|---|---|
| 1 | Read the problem and determine what you are asked to find. |
| 2 | Identify the fractions and their denominators. |
| 3 | Convert the fractions to have a common denominator. |
| 4 | Add the fractions. |
| 5 | Simplify the fraction if necessary. |
| 6 | Check your answer to ensure it makes sense with the problem. |
Let's practice understanding tenths and hundredths problems with a couple of exercises.
Solving Tenths and Hundredths Problems – Example
Example 1:
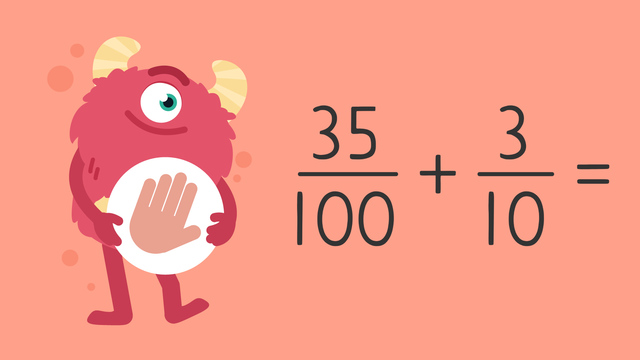
Problem: Solve $\frac{35}{100} + \frac{3}{10}$.
Steps to Solve the Problem:
| Step # | Action | Equation |
|---|---|---|
| 1 | Write an equation for the problem. | $\frac{35}{100}$ + $\frac{3}{10}$ |
| 2 | Convert three-tenths to hundredths. | $\frac{3}{10} \times \frac{10}{10}$ = $\frac{30}{100}$ |
| 3 | Add the fractions. | $\frac{35}{100}$ + $\frac{30}{100}$ = $\frac{65}{100}$ |
| 4 | Combine the fractions. | $\frac{65}{100}$ |
Solution: The sum is $\frac{65}{100}$ or sixty-five hundredths.
Example 2:
Problem: Solve $\frac{3}{100} + \frac{5}{10}$.
Steps to Solve the Problem:
| Step # | Action | Equation |
|---|---|---|
| 1 | Write an equation for the problem. | $\frac{3}{100}$ + $\frac{5}{10}$ |
| 2 | Convert five-tenths to hundredths. | $\frac{5}{10} \times \frac{10}{10} = \frac{50}{100}$ |
| 3 | Add the fractions. | $\frac{3}{100} + \frac{50}{100} = \frac{53}{100}$ |
| 4 | Combine the fractions. | $\frac{53}{100}$ |
Solution: The sum is $\frac{53}{100}$ or fifty-three hundredths.
Example 3:
Problem: Solve $\frac{30}{100} + \frac{2}{10}$.
Steps to Solve the Problem:
| Step # | Action | Equation |
|---|---|---|
| 1 | Write an equation for the problem. | $\frac{30}{100} + \frac{2}{10}$ |
| 2 | Convert thirty-hundredths to tenths. | $\frac{30}{100} \div \frac{10}{10} = \frac{3}{10}$ |
| 3 | Add the fractions. | $\frac{3}{10} + \frac{2}{10} = \frac{5}{10}$ |
| 4 | Simplify the fraction if necessary. | $\frac{5}{10} = \frac{1}{2}$ |
Solution: The sum is $\frac{5}{10}$ or $\frac{1}{2}$.
Solving Tenths and Hundredths Problems – Application
Now, let's put your skills to the test. Solve these problems on your own, and check the solutions when you're ready!
Solving Tenths and Hundredths Problems – Summary
Key Learnings from this Text:
- Solving problems with tenths and hundredths can be achieved by following these steps:
| Step # | Action |
|---|---|
| 1 | Read the problem and determine what you are asked to find. |
| 2 | Identify the fractions and their denominators. |
| 3 | Convert the fractions to have a common denominator. |
| 4 | Add the fractions. |
| 5 | Simplify the fraction if necessary. |
| 6 | Check your answer to ensure it makes sense with the problem. |
- Mastering addition with tenths and hundredths is an important foundational math skill.
Keep practicing these steps, and you'll become a pro at solving tenths and hundredths problems! Check out more fun math challenges and exercises on our website to continue sharpening your skills.
Solving Tenths and Hundredths Problems – Frequently Asked Questions
Transcript Adding Tenths and Hundredths — Let's Practice!
Razzi says get these item ready, because today we're going to practice adding tenths and hundredths. It's time to begin! Solve thirty-five hundredths plus three-tenths. Pause the video to work on the problem, and press play when you are ready to see the solution! We need like denominators to add. Start by converting three-tenths. Multiply the numerator and denominator by ten to get thirty-hundredths. Then, carry over one hundred for the denominator. Thirty-five plus thirty equals sixty-five. Did you also get sixty-five hundredths? Let's tackle the next problem! Solve three-hundredths plus five-tenths. Pause the video to work on the problem, and press play when you are ready to see the solution! We need like denominators to add. Start by converting five-tenths. Multiply the numerator and denominator by ten to get fifty-hundredths. Then, carry over one hundred for the denominator. Three plus fifty equals fifty-three. Did you also get fifty-three hundredths? Let's tackle the final problem! Solve thirty-hundredths plus two-tenths. This time, convert thirty-hundredths to tenths! Pause the video to work on the problem, and press play when you are ready to see the solution! Start by converting thirty hundredths to tenths. Divide the numerator and denominator by ten to get three-tenths. Then, carry over ten for the denominator. Three plus two equals five. Five tenths can be converted to one half! Did you also get five tenths or one half? Razzi had so much fun practicing with you today! See you next time!
Adding Tenths and Hundredths — Let's Practice! exercise
-
Identify the expressions that are equivalent.
HintsTo compare the expressions, you must first create like-denominators.
The fractions, $\frac{55}{100}$ and $\frac{3}{100}$ share like denominators. The fractions, $\frac{55}{100}$ and $\frac{3}{10}$ do not.
SolutionWhen you add tenths and hundredths you need to first make sure the denominators are the same. By multiplying the numerator and the denominator of $\frac{3}{10}$ you make $\frac{30}{100}$, which you can then add to $\frac{35}{100}$. The same applies with $\frac{5}{10}$, $\frac{3}{10}$, and $\frac{4}{10}$.
- $\frac{3}{10}$ x 10 = $\frac{30}{100}$ = $\frac{35}{100}$ + $\frac{30}{100}$
- $\frac{5}{10}$ x 10 = $\frac{50}{100}$ = $\frac{3}{100}$ + $\frac{50}{100}$
- $\frac{6}{10}$ x 10 = $\frac{60}{100}$ = $\frac{60}{100}$ + $\frac{2}{100}$
- $\frac{4}{10}$ x 10 = $\frac{40}{100}$ = $\frac{40}{100}$ + $\frac{45}{100}$
-
Identify the expressions that are equivalent.
HintsTo compare the expressions, you must first create like-denominators.
To compare the expressions, you must first create like-denominators.
The fractions, $\frac{55}{100}$ and $\frac{33}{100}$ share like denominators. The fractions, $\frac{55}{100}$ and $\frac{33}{10}$ do not.When creating like denominators don't forget to divide or multiply the numerator as well!
Solution- $\frac{50}{100}$ ÷ 10 = $\frac{5}{10}$
- $\frac{20}{100}$ ÷ 10 = $\frac{2}{10}$
- $\frac{40}{100}$ ÷ 10 = $\frac{4}{10}$
- $\frac{10}{100}$ ÷ 10 = $\frac{1}{10}$
-
Solve the equations.
HintsUse a pencil and paper to help you keep track of the steps.
With the equation, $\frac{35}{100}$ + $\frac{3}{10}$, and $\frac{3}{100}$ + $\frac{5}{10}$, it is easiest to multiply to create like denominators.
With the equation, $\frac{30}{100}$ + $\frac{2}{10}$, it is easiest to divide to create like denominators.
Do NOT simplify the fractions!
Solution- $\frac{35}{100}$ + $\frac{3}{10}$ = $\frac{35}{100}$ + $\frac{30}{100}$ = $\frac{65}{100}$
- $\frac{3}{100}$ + $\frac{5}{10}$ = $\frac{3}{100}$ + $\frac{50}{100}$ = $\frac{53}{100}$
- $\frac{30}{100}$ + $\frac{2}{100}$ = $\frac{3}{10}$ + $\frac{2}{10}$ = $\frac{5}{10}$
-
Simplify the solutions.
HintsIn the solution to $\frac{40}{100}$ + $\frac{40}{100}$, both 80 and 100 can be divided by 10. This solution can then be further simplified by dividing by 2.
Simplify the answer as many times as necessary.
Solution- $\frac{40}{100}$ + $\frac{40}{100}$ = $\frac{80}{100}$
- $\frac{80}{100}$ ÷ $\frac{10}{10}$ = $\frac{8}{10}$
- $\frac{8}{10}$ ÷ $\frac{2}{2}$ = $\frac{4}{5}$
-
Convert the tenths fraction to hundredths using multiplication.
HintsAlways remember to multiply both the numerator (above) and denominator (below) by the same number.
Multiply or divide to create like denominators before attempting to solve.
Solution- 7 x 10 = 70, 10 x 10 = 100
- $\frac{70}{100}$ + $\frac{8}{100}$ = $\frac{78}{100}$
-
Solve the equations.
HintsIn the equation, $\frac{20}{100}$ + $\frac{5}{10}$, $\frac{20}{100}$ can be divided by 10 to make equal denominators.
Remember to simplify as many times as necessary. If there is a common denominator between the numerator and denominator, divide again!
Solution- $\frac{20}{100}$ + $\frac{5}{10}$ = $\frac{2}{10}$ + $\frac{5}{10}$ = $\frac{7}{10}$
- $\frac{30}{100}$ + $\frac{2}{10}$ = $\frac{3}{10}$ + $\frac{2}{10}$ = $\frac{5}{10}$ = $\frac{1}{2}$
- $\frac{3}{10}$ + $\frac{50}{100}$ = $\frac{3}{10}$ + $\frac{5}{10}$ = $\frac{8}{10}$ = $\frac{4}{5}$
- $\frac{4}{10}$ + $\frac{20}{100}$ = $\frac{4}{10}$ + $\frac{2}{10}$ = $\frac{6}{10}$ = $\frac{3}{5}$
Adding Fractions on a Number Line
Adding Fractions with Like Denominators
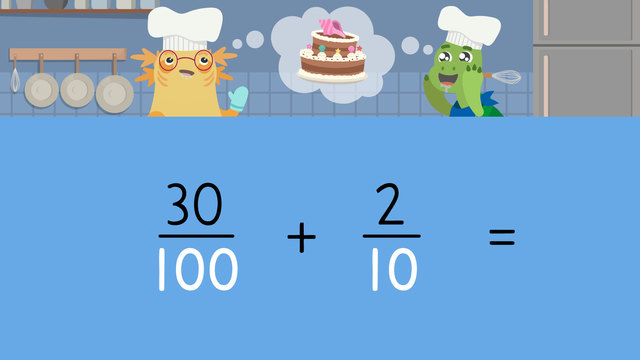
Adding Tenths and Hundredths

Adding Tenths and Hundredths — Let's Practice!
Adding and Subtracting Mixed Numbers
Adding Fractions on a Number Line — Let's Practice!
Adding Fractions with Unlike Denominators
Adding Mixed Numbers with Unlike Denominators









