Adding Fractions with Unlike Denominators

Basics on the topic Adding Fractions with Unlike Denominators
Adding Fractions with Unlike Denominators
Looking for a comprehensive and engaging way to help your 5th grader master the concept of adding fractions with unlike denominators? Look no further than our tutorial video on adding fractions with unlike denominators by Sofatutor!
Designed specifically for 5th graders, this video is the perfect tool to help your child understand the fundamentals of adding fractions with different denominators. With clear explanations, helpful examples, and interactive practice problems, our video is sure to keep your child engaged and motivated as they learn.
Whether your child is struggling with this concept or simply looking for a new way to learn, our tutorial video by Sofatutor is the perfect solution. So why wait? Start your child's journey to math mastery today with our adding fractions with unlike denominators video! With our engaging and informative content, your child will be well on their way to understanding this important mathematical concept in no time.
Transcript Adding Fractions with Unlike Denominators
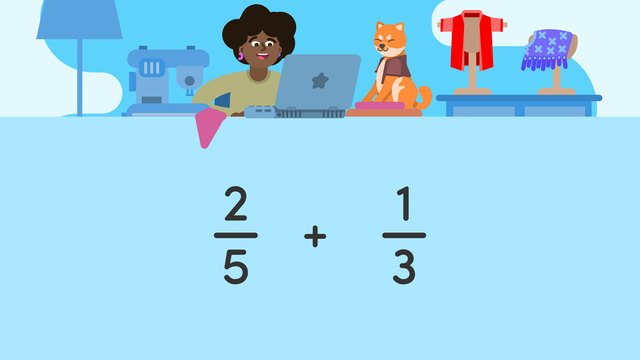
June is preparing to launch her company, Fifi's Fancy Frocks. She is finishing up the final outfits when she realizes she only has a few materials left. She will need to add various fractional amounts to determine how much material she has to work with by... Adding Fractions with Unlike Denominators. Let's do a quick vocabulary review. A least common multiple is the smallest multiple that two or more numbers have in common. A least common denominator is the smallest number that can be used for all denominators of fractions. The greatest common factor is the largest number that can be divided evenly into two or more numbers. Our previous experience with fractions, combined with these terms, will be useful when adding with unlike denominators. June has two spools of red ribbon remaining. The first has two-fifths of a yard, while the second has one-third. To see how much red ribbon there is, we'll add two-fifths and one-third. Before we can add, we must rename fractions with unlike denominators as equivalent fractions with a common denominator. We will find the least common denominator of five and three by thinking about the least common multiple they share. What is the least common multiple of five and three? Fifteen. Now, using the common denominator, fifteen, we rename each fraction. To do this, we multiply two and five by three, and one and three by five. We now have six-fifteenths and five-fifteenths... These fractions can now be added. We bring down the denominator, fifteen because the number of equal parts does not change and add the numerators to get eleven. Two-fifths plus one-third equal eleven-fifteenths. Eleven-fifteenths cannot be simplified because they share only one factor. June has eleven-fifteenths of a yard of the red ribbon. She also has enough flannel material to make another shirt. We can calculate the number of yards available by adding three-sixths and two-twelfths. This time, pause to solve the problem and press play when you're ready to review. The least common denominator of six and twelve is twelve. Because we multiply the numerator and denominator by two, three-sixths becomes six-twelfths. Two-twelfths remains the same because twelve is already in the denominator. To add, bring down the denominator, twelve, and add the numerators, to get eight. The answer is eight-twelfths. Looking at eight-twelfths, we can see that it can be SIMPLIFIED because the numerator and denominator share common factors. FOUR is the greatest common factor of eight and twelve... so they can be divided by four. Eight-twelfths is simplified to two-thirds. June has two-thirds of a yard of the flannel material. To summarize... Before we can add, we must rename fractions with unlike denominators as equivalent fractions with a common denominator. Then, bring down the denominator... add the numerators and, if necessary, simplify the fraction. Fifi's Fancy Frocks is officially open for business!
Adding Fractions with Unlike Denominators exercise
-
Define the terms.
HintsA denominator is part of a fraction.
Look for common words on left and right sides to help you make matches.
Think of the word least as small, and the word greatest as large.
Solution- The Least Common Multiple, or LCM is smallest multiple that two numbers have in common.
- The Least Common Denominator, or LCD is the smallest number that can be used to make equal denominators in fractions.
- The Greatest Common Factor or GCF is the largest number that can be divided evenly into 2 or more numbers.
- The Denominator is the number below the fraction line.
-
Sort the steps.
HintsThe numerator is the number above the fraction line, and the denominator is the number below.
Before adding fractions with unlike denominators, you need to create a common denominator using multiplication or division.
Example: $\frac{1}{2}$ and $\frac{1}{4}$
$\frac{1}{2}$ x $\frac{2}{2}$ = $\frac{2}{4}$
$\frac{2}{4}$ and $\frac{1}{4}$ have common denominators and can now be added together.SolutionThe steps to add fractions with unlike denominators are:
1) Rename fractions with unlike denominators as equivalent fractions with the same denominator ($\frac{1}{12}$ + $\frac{1}{4}$ becomes $\frac{1}{12}$ + $\frac{3}{12}$)
2) Bring the common denominator over to the solution side of the equation ($\frac{1}{12}$ + $\frac{3}{12}$ = $\frac{}{12}$)
3) Add the numerators ($\frac{1}{12}$ + $\frac{3}{12}$ = $\frac{4}{12}$)
4) Simplify the fraction if necessary ($\frac{4}{12}$ becomes $\frac{1}{3}$) -
Add fractions with unlike denominators.
HintsSometimes the least common denominator is already the denominator of one of the fractions in the equation.
Starting in step one, the Least Common Denominator (15) is given. What do you multiply each fraction by to get 15 in the denominator?
You have the solution for $\frac{3}{15}$ + $\frac{2}{15}$ = ? from step 3. Now divide both the numerator and denominator of this fraction by 5 to simplify.
SolutionSTEP ONE
$\frac{1}{5}$ x $\frac{3}{3}$ = $\frac{3}{15}$
$\frac{2}{15}$ x $\frac{1}{1}$ = $\frac{2}{15}$
STEP TWO
$\frac{3}{15}$ + $\frac{2}{15}$ = $\frac{}{15}$
STEP THREE
$\frac{3}{15}$ + $\frac{2}{15}$ = $\frac{5}{15}$
STEP FOUR
$\frac{5}{15}$ ÷ $\frac{5}{5}$ = $\frac{1}{3}$ -
Solve the equations.
HintsIn order to add fractions with unlike denominators, first create equivalent fractions with the same denominator using multiplication or division.
Example:
$\frac{1}{5}$ + $\frac{2}{15}$
$\frac{1}{5}$ x $\frac{3}{3}$ = $\frac{3}{15}$
$\frac{2}{15}$ x $\frac{1}{1}$ = $\frac{2}{15}$
$\frac{3}{15}$ and $\frac{2}{15}$ can be added togetherNext, Bring the common denominator over to the solution side of the equation and add the numerators together.
Example:
$\frac{3}{15}$ + $\frac{2}{15}$ = $\frac{5}{15}$Finally, look for a Greatest Common Factor, and simplify if possible.
Example:
$\frac{5}{15}$ can be simplified to $\frac{1}{3}$ by dividing the numerator and denominator by 5.One of the solutions must be simplified.
Solution1) $\frac{3}{10}$ + $\frac{3}{5}$ = ?
$\frac{3}{10}$ + $\frac{6}{10}$ = $\frac{9}{10}$2) $\frac{1}{4}$ + $\frac{1}{6}$ = ?
$\frac{3}{12}$ + $\frac{2}{12}$ = $\frac{5}{12}$3) $\frac{1}{3}$ + $\frac{2}{9}$ = ?
$\frac{3}{9}$ + $\frac{2}{9}$ = $\frac{5}{9}$4) $\frac{1}{10}$ + $\frac{3}{20}$ = ?
$\frac{2}{20}$ + $\frac{3}{20}$ = $\frac{5}{20}$ = $\frac{1}{4}$ -
Find the least common denominator.
HintsThe Least Common Denominator, or LCD is the smallest number that can be used to make equal denominators in fractions.
Example: The LCD for $\frac{1}{4}$ and $\frac{1}{5}$ is 20.Since all of the fractions have even numbers as denominators, the answer cannot be an odd number.
Try multiplying the greatest denominator (8) by 2 and see if the other denominators can fit into the solution. If not, the answer must be a greater number.
Solution- 4 x 6 = 24
- 6 x 4 = 24
- 8 x 3 = 24
-
Solve the equations.
HintsIn order to add fractions with unlike denominators, first create equivalent fractions with the same denominator using multiplication or division.
Example:
$\frac{1}{5}$ + $\frac{2}{15}$
$\frac{1}{5}$ x $\frac{3}{3}$ = $\frac{3}{15}$
$\frac{2}{15}$ x $\frac{1}{1}$ = $\frac{2}{15}$
$\frac{3}{15}$ and $\frac{2}{15}$ can be added togetherNext, Bring the common denominator over to the solution side of the equation and add the numerators together.
Example:
$\frac{3}{15}$ + $\frac{2}{15}$ = $\frac{5}{15}$Finally, look for a Greatest Common Factor, and simplify if possible.
Example:
$\frac{5}{15}$ can be simplified to $\frac{1}{3}$ by dividing the numerator and denominator by 5.Three of the solutions must be simplified.
Solution1) $\frac{3}{9}$ + $\frac{1}{3}$ = ?
$\frac{3}{9}$ + $\frac{3}{9}$ = $\frac{6}{9}$ = $\frac{2}{3}$2) $\frac{1}{6}$ + $\frac{2}{12}$ = ?
$\frac{2}{12}$ + $\frac{2}{12}$ = $\frac{4}{12}$ = $\frac{1}{3}$3) $\frac{1}{8}$ + $\frac{3}{16}$ = ?
$\frac{2}{16}$ + $\frac{3}{16}$ = $\frac{5}{16}$ This fraction cannot be further simplified.4) $\frac{1}{18}$ + $\frac{1}{6}$ = ?
$\frac{1}{18}$ + $\frac{3}{18}$ = $\frac{4}{18}$ = $\frac{2}{9}$
Adding Fractions on a Number Line
Adding Fractions with Like Denominators
Adding Tenths and Hundredths
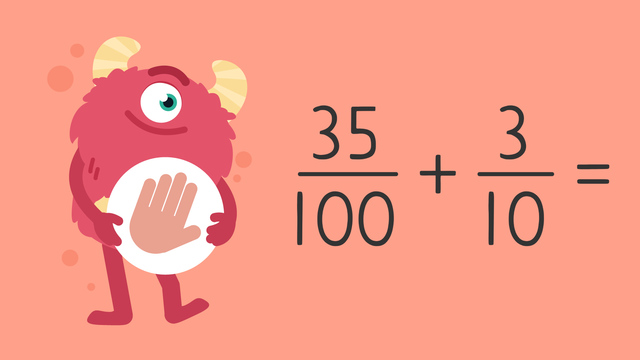
Adding Tenths and Hundredths — Let's Practice!
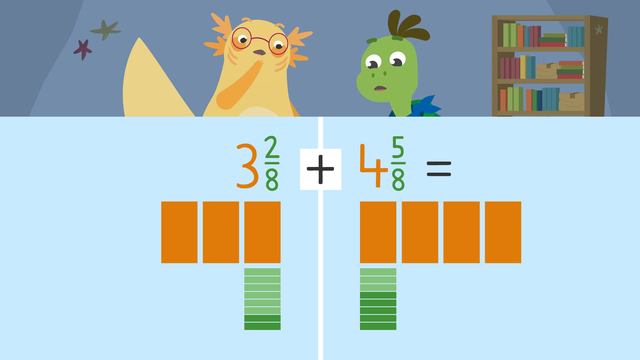
Adding and Subtracting Mixed Numbers
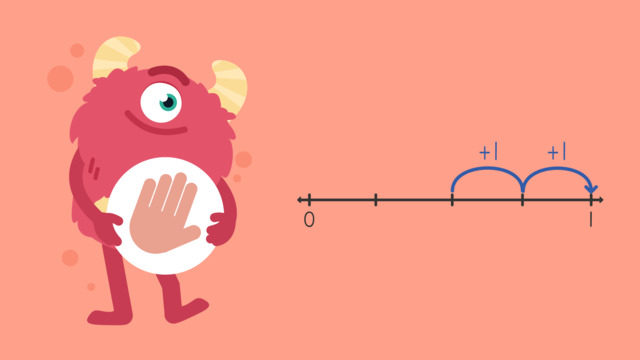
Adding Fractions on a Number Line — Let's Practice!

Adding Fractions with Unlike Denominators
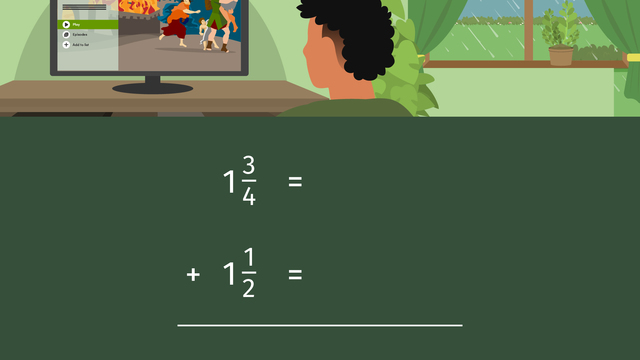
Adding Mixed Numbers with Unlike Denominators










🤨???????????????????????????????????????????????????