Adding Fractions with Like Denominators
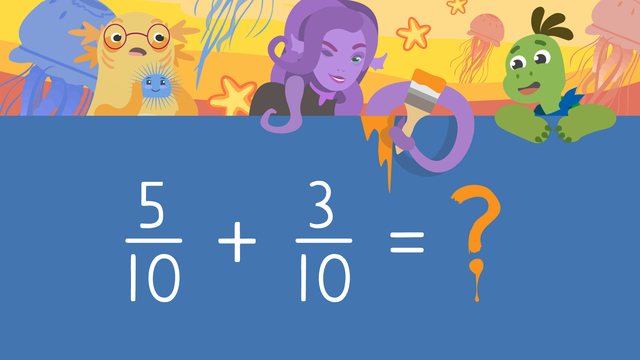

Basics on the topic Adding Fractions with Like Denominators
Adding Fractions with Like Denominators
Adding fractions with the same denominators is similar to addition with whole numbers, but instead we are adding simple fractions. When adding fractions with common or same denominators, there are some rules to adding fractions that need to be followed. To add fractions there are three simple steps:
| Step # | What to do |
|---|---|
| 1 | Check that the denominators are the same, and write it in the total. |
| 2 | Add the numerators. |
| 3 | Write the numerator above the denominator in the total. |
These steps are the rules for adding fractions with like denominators. Many have asked “when adding fractions do you add the denominator?” and the answer is no. The number of parts your whole is broken into does not change, so we never add the denominators when adding two fractions.
Adding Fractions with Like Denominators – Example
Let’s take a look at an example of adding fractions with same denominator and adding fractions and simplifying. Here, we’ll add five-tenths plus three-tenths following the steps and rules for adding fractions with like denominators.
- Step 1: Check that the denominators are the same, and write it in the total
- Step 2: Add the numerators
- Step 3: Write the numerator above the denominator in the total
Last, we can simplify eight-tenths into four-fifths.
Adding Fractions with Like Denominators – Summary
When adding fractions with the same denominators, we keep in mind the rules for adding fractions with like denominators. To add fractions there are three simple steps:
- Step 1: Double check that the denominators are the same, and write it in the total.
- Step 2: Add the numerators.
- Step 3: Write the numerator above the denominator in the total.
Want some more adding fractions practice? In this video you can find adding fractions examples and on this website there is adding fractions with like denominators worksheets along with other activities, and exercises.
Transcript Adding Fractions with Like Denominators
Can you hear that sound? It sounds like Ophelia, the sea witch, crying inside of her lair! Axel and Tank NEED to know what the fuss is about... ...and they find that Ophelia is trying to get ready for a party, but has made a big mess! She needs help counting the jellyfish, starfish, and sea urchin decorations she is painting for a party. Let's practice [title] "adding fractions with like denominators" to count the decorations she is painting. A fraction describes a part of a whole OR a part of a collection.
The top number, or the NUMERATOR, represents how many equal parts of a whole we have. The bottom number, or the DENOMINATOR, represents how many equal parts our whole is divided into. (...) A COMMON or LIKE denominator refers to fractions with the SAME denominators. To start, Ophelia paints THREE out of SEVEN, or three-sevenths, of her jellyfish orange. If she paints two more, how many jellyfish will be painted total? (...) We can find the TOTAL by solving the equation three-sevenths PLUS two-sevenths. When you add fractions with LIKE denominators... the denominator ALWAYS stays the same since the number of parts in our whole does NOT change. That is why our first step is to double check that the denominators are the SAME. Since they are LIKE denominators we can write the denominator of our total HERE. The second step is to add the numerators. Three plus two is five, and we write it HERE. (...) That means, five-sevenths of Ophelia's jellyfish will be painted orange. Next, she paints five-tenths of the starfish yellow. If she paints three more, how many starfish will be painted total? (...) This time we will add five-tenths PLUS three-tenths. What is the first step? (...) First, double check that the denominators are the same, (...) and write it HERE. What is the second step? (...) The second step is to add the numerators. What is five plus three? (...) The sum is eight. (...) Eight-tenths can be simplified to four-fifths... which means, eight tenths OR four-fifths of Ophelia's starfish will be painted yellow. Finally, she paints four-twelfths of the sea urchins red... and she will paint eight more. (...) What is four-twelfths PLUS eight-twelfths? (...) First, double check that the denominators are the same, (...) and write it HERE. Next, add four plus eight to get (...) twelve. Twelve-twelfths can be simplified to one whole... which means Ophelia will paint ALL of her sea urchins red! Remember, (...) when adding fractions with LIKE denominators... The first step is to "double check that the denominators are the same, and write it in the total". When you add fractions with LIKE denominators, the denominators ALWAYS stay the same and DO NOT get added. The second step is to "add the numerators". The third step is to write the numerator above the denominator in the total, simplifying the fraction when you need to. Let's see how Ophelia's party turned out! Looks like she's the light of the party! [Ophelia is lit up on the ceiling as a decoration for the party as well]
Adding Fractions with Like Denominators exercise
-
Adding fractions.
HintsRemember that when we add fractions with the same denominator, we only need to look at the numerators and add these.
When we add fractions with the same denominator, this stays the same.
Solution- $\frac{4}{12}$ + $\frac{6}{12}$ = $\frac{10}{12}$
- $\frac{3}{10}$ + $\frac{5}{10}$ = $\frac{8}{10}$
- $\frac2 7$ + $\frac1 7$ = $\frac3 7$
- $\frac3 9$ + $\frac5 9$ = $\frac8 9$
-
Add the fractions of shells.
HintsThe denominator is how many parts (shells) we have in total, it always remains the same.
How many shells have been painted for each color? This is our numerator because it is the part of the whole.
SolutionThere are 8 shells in total, so this is our denominator.
3 out of 8 are painted yellow, this is $ \frac3 8$.
2 out of 8 are painted red, this is $ \frac2 8$.
In total, there are 5 out of 8 painted, $ \frac5 8$.
-
Painted shells.
HintsRemember that when we find fractions of amounts, the total amount in the group is the denominator (the bottom part of the fraction). In this image there are 5 shells in total so the denominator would be 5.
The parts that we are counting (here, the painted shells) is the numerator. In this example it is 3.
SolutionThere are 6 shells in total. 3 are painted purple and 2 are painted red. The fraction of shells that have been painted is $\mathbf{\frac{5}{6}}$
To find the total fraction of shells that have been painted we need to add $\mathbf{\frac{2}{8}}$ and $\mathbf{\frac{3}{8}}$ to get $\mathbf{\frac{5}{8}}$
There are 5 shells in total. $\mathbf{\frac{1}{5}}$ + $\mathbf{\frac{2}{5}}$ = $\mathbf{\frac{3}{5}}$.
-
Pizza fractions.
HintsRemember that when we add fractions with the same denominator, we only add the numerators together. The denominator stays the same.
How many slices are left? This is the numerator. In this example there is one slice left out of four, so $\frac1 4$ remains.
How many slices was each pizza originally cut into? This is your denominator.
SolutionThe image shows the answer to the pepperoni pizza:
$\frac3 8$ + $\frac4 8$ = $\frac7 8$.
Mushroom pizza:
$\frac2 6$ + $\frac3 6$ = $\frac5 6$.
Olive pizza:
$\frac2 5$ + $\frac2 5$ = $\frac4 5$.
Cheese pizza:
$\frac4 8$ + $\frac2 8$ = $\frac6 8$.
-
Fractions of fish.
HintsWhen we are trying to find $ \frac4 7$ of an amount, we know that there are 7 items in total since this is the denominator.
What is the numerator? This is how many out of the total that are colorful or have color. In this example, the numerator is 4.
SolutionThe tank with $ \frac4 7$ of the fish being colorful, is the tank with 7 fish in the tank -this is the denominator-and 4 fish with color in the tank (3 orange and 1 yellow fish) - this is the numerator.
-
Add fractions.
HintsRemember that we can simplify fractions by dividing the numerator and the denominator by the same factor, to find an equivalent fraction.
Here $ \frac2 4$ has been simplified to $ \frac1 2$ by dividing the numerator and the denominator by 2.
Solution$\mathbf{\frac{2}{3}}$
- $\frac1 3$ + $\frac1 3$ = $\frac2 3$.
- $\frac2 6$ + $\frac2 6$ = $\frac4 6$ .
- We can simplify $\frac4 6$ to $\frac2 3$ by dividing both the numerator and denominator by 2.
$\mathbf{\frac{1}{2}}$
- $\frac2 8$ + $\frac2 8$ = $\frac4 8$.
- We can simplify $\frac4 8$ to $\frac1 2$ by dividing both the numerator and denominator by 4.
- $\frac{4}{10}$ + $\frac{1}{10}$ = $\frac{5}{10}$.
- We can simplify $\frac{5}{10}$ to $\frac1 2$ by dividing both the numerator and denominator by 5.
$\mathbf{\frac{4}{5}}$
- $\frac3 5$ + $\frac1 5$ = $\frac4 5$.
- $\frac{6}{10}$ + $\frac{2}{10}$ = $\frac{8}{10}$.
- We can simplify $\frac{8}{10}$ to $\frac4 5$ by dividing both the numerator and denominator by 2.
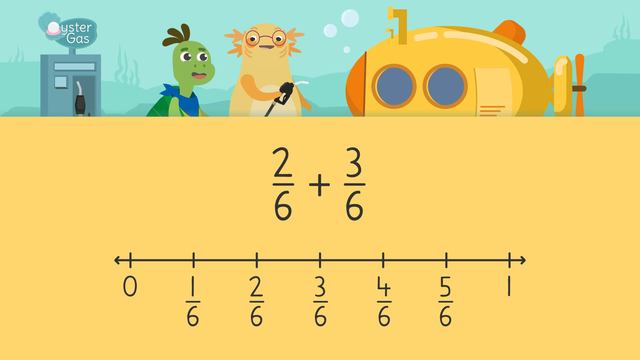
Adding Fractions on a Number Line
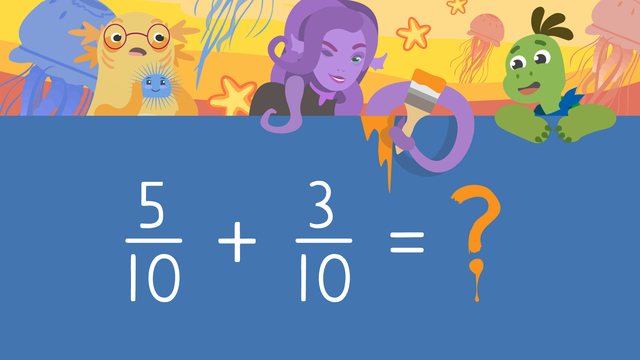
Adding Fractions with Like Denominators
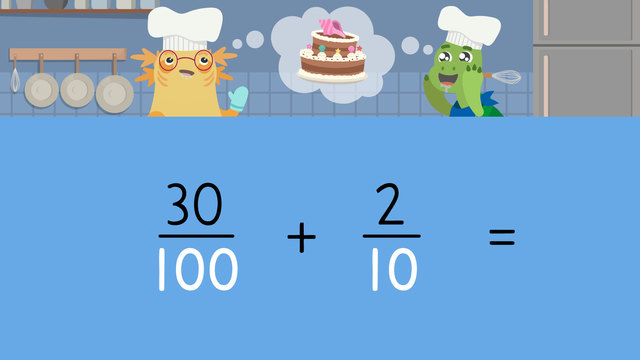
Adding Tenths and Hundredths
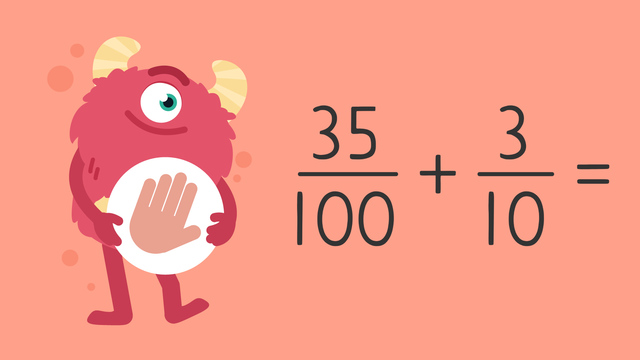
Adding Tenths and Hundredths — Let's Practice!
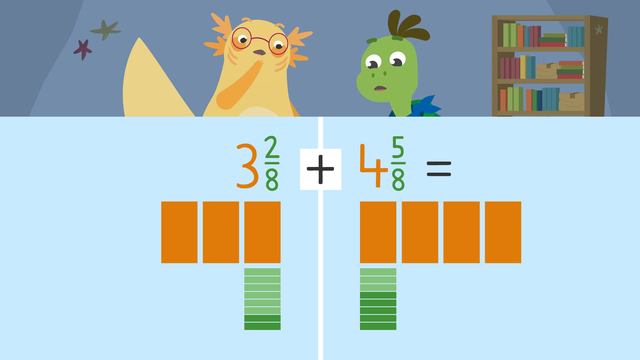
Adding and Subtracting Mixed Numbers
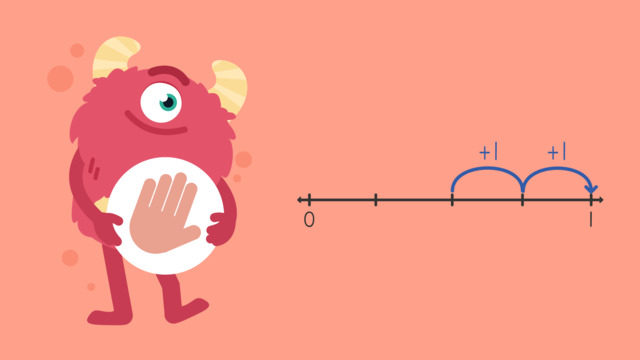
Adding Fractions on a Number Line — Let's Practice!
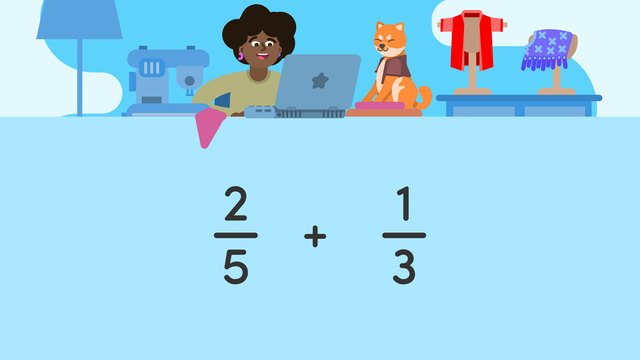
Adding Fractions with Unlike Denominators
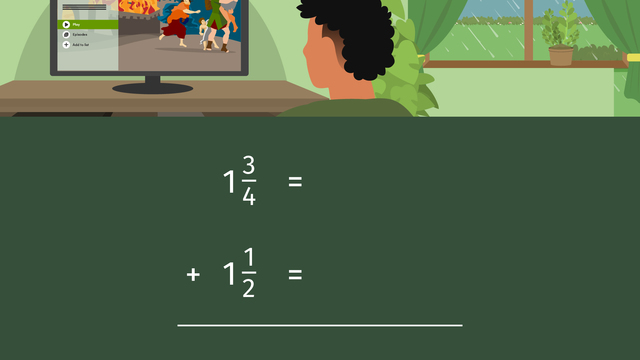
Adding Mixed Numbers with Unlike Denominators














AMAZING!!!
Great video!!!!!!!!