Proportional Relationships and Measuring Rates
- Proportional Relationships and Measuring Rates
- Identifying Proportionality in Tables
- Writing Proportional Relationship Equations
- Applying Proportional Relationship Equations
- Proportional Relationships and Measuring Rates – Summary
- Proportional Relationships and Measuring Rates – Frequently Asked Questions
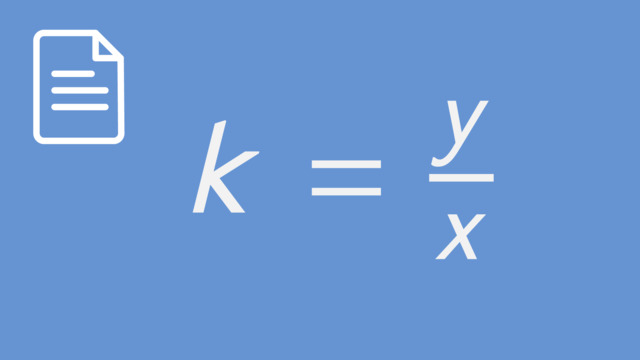
Basics on the topic Proportional Relationships and Measuring Rates
Proportional Relationships and Measuring Rates
Proportional relationships are key to understanding how different quantities relate and influence each other. In math, we explore these relationships to discover how consistently changing one thing can affect another. For instance, think about buying snacks at a movie theater. If one bag of popcorn costs $\$3$, then two bags would cost $\$6$, three bags would cost $\$9$, and so on. This consistent change - where the total cost changes in direct proportion to the number of popcorn bags - is a perfect example of a proportional relationship.
A proportional relationship is when two quantities increase or decrease at the same constant rate, meaning their ratio always remains the same. This consistent ratio shows how one quantity is directly related to the other.
| Concept | Explanation |
|---|---|
| Rate | A rate is a comparison of two different types of quantities, like speed (miles per hour) or density (people per square mile). |
| Ratio | A ratio shows how two quantities are related, like the ratio of cats to dogs in a pet store. |
| Unit Rate | The comparison of a quantity to one unit of another quantity, like if a car travels 60 miles in 2 hours, the unit rate is 30 miles per hour. |
| Constant of Proportionality ($k$) | This constant value shows the relationship between two quantities that are directly proportional to each other, calculated using the formula $k = \frac{y}{x}$. |
Identifying Proportionality in Tables
Finding rates with division is the key process for identifying proportional relationships in tables.
Checking for Proportional Relationships: Divide the '$y$' values by the corresponding '$x$' values. If these ratios are the same, then the relationship is proportional.
Finding the Constant of Proportionality: The consistent ratio value is the constant of proportionality.
Let’s look at some examples to find the proportionality in a table.
A car's fuel efficiency is measured over various distances. We have a table listing the distance traveled and the corresponding fuel used.
Checking for Proportional Relationships:
- For 50 miles: $\frac{2 \text{ gallons}}{50 \text{ miles}} = \frac{2}{50}$
- For 100 miles: $\frac{4 \text{ gallons}}{100 \text{ miles}} = \frac{4}{100}$
- For 150 miles: $\frac{6 \text{ gallons}}{150 \text{ miles}} = \frac{6}{150}$
These ratios simplify to the same value: $\frac{1}{25}$.
Finding the Constant of Proportionality: Since all ratios are equal, the constant of proportionality is $\frac{1}{25}$ gallons per mile.
A baker uses sugar in various batches of cookies. The table shows the relationship between the batches of cookies and the sugar used.
Check for Proportional Relationships:
- For 1 batch: $\frac{3 \text{ cups}}{1 \text{ batch}} = \frac{3}{1}$
- For 2 batches: $\frac{6 \text{ cups}}{2 \text{ batches}} = \frac{6}{2}$
- For 3 batches: $\frac{9 \text{ cups}}{3 \text{ batches}} = \frac{9}{3}$
These ratios simplify to the same value: $3$ cups per batch.
Finding the Constant of Proportionality: The constant of proportionality is $3$ cups per batch, as each ratio equals $3$.
Try some examples on your own!
Writing Proportional Relationship Equations
Representing Proportional Relationships with Equations is key in math. The equation $y = kx$ neatly describes such relationships, where "$k$" represents the constant rate at which quantities change in relation to each other. This equation is vital for translating real-world scenarios into mathematical terms, simplifying problem-solving processes.
Let’s take a look at some real-world examples.
In this scenario, we observe the growth of a plant and how it correlates with the amount of water it receives each week.
Constant of Proportionality ($k$): After dividing the growth by the water for each entry, we find that $k = 3$ cm per liter.
Writing the Equation:
- The general formula for a proportional relationship is $y = kx$.
- Substituting our $k$ value, the equation becomes $y = 3x$.
- This equation represents the relationship: "For every liter of water, the plant grows $3$ centimeters."
Let’s observe the relationship between data usage and the hours spent online.
Constant of Proportionality (k): Here, $k$ consistently works out to $1.5$ GB per hour.
Writing the Equation:
- The proportional relationship is given by $y = kx$.
- Inserting our $k$ value, we get $y = 1.5x$.
- This equation tells us that for each hour spent online, $1.5$ GB of data is used.
Try some on your own!
Applying Proportional Relationship Equations
Once you have the equation for a proportional relationship, you can easily find one quantity if you know the other. This is very handy for solving real-world problems. Let's see how it works with some examples.
We can explore how data usage correlates with time spent online. By using the equation $y = 1.5x$, where $y$ is the data used in GB and $x$ is the number of hours spent online, we can find out the total data usage after a specific period of online activity.
- Given Equation: $y = 1.5x$ (Where $y$ is the data used in GB and $x$ is the number of hours spent online)
- Find: How much data is used after 4 hours online?
- Solution: Substitute $x$ with 4 in the equation: $y = 1.5 \times 4 = 6$. So, after $4$ hours online, $6$ GB of data is used.
In this situation, we're looking at the distance a car can travel over a period of time. Using the equation $y = 60x$, where $y$ represents the distance in miles and $x$ stands for the time in hours, we can determine how far the car travels in a given amount of time.
- Given Equation: $y = 60x$ (Where $y$ is the distance in miles and $x$ is the time in hours)
- Find: How far does a car travel in 3 hours?
- Solution: Substitute $x$ with 3 in the equation: $y = 60 \times 3 = 180$. The car travels $180$ miles in $3$ hours.
Now, try solving these problems on your own!
Proportional Relationships and Measuring Rates – Summary
Key Learnings from this Text:
- Proportional relationships describe how two quantities change consistently in relation to each other.
- The constant of proportionality ($k$) is a key concept, allowing for the creation of simple equations like $y = kx$ to model these relationships.
- Understanding and applying proportional relationships is crucial for solving real-world problems involving rates, ratios, and direct relationships.
Proportional Relationships and Measuring Rates – Frequently Asked Questions
Transcript Proportional Relationships and Measuring Rates
Why is Jackson up so early, and weaving through Los Angeles traffic like that? Well, Jackson is a huge fan of video games and today is Black Friday! He hopes to get to his favorite video game store, Game Another Day, before it opens so he can get some great deals on new games before they sell out. The store is tucked deep inside a gigantic mall and he’s stuck in a line getting into the parking garage! It’s 7:36, and the store opens at 8:00 AM! He’s only got 24 minutes to get there before it opens! He’ll need to measure rates and use proportional relationships if he has any hope of making it! Jackson’s got to think fast — should he stay in this line or ditch the car and make a run for it? His heart’s racing as he counts the cars to determine his place in the line. He’s the 30th car. Argh! He times the cars to find the rate at which they are passing the gate. After 1 minute 2 cars have passed through. After 2 minutes, 4 cars have passed through, and after 3 minutes, 6. We need to find the pattern, so we can calculate how long it will take for all 30 cars to pass. Can you see a pattern? The cars are passing through at a constant rate! But what is the rate? Remember, a rate is a ratio. The first ordered pair in the table tells us 2 cars pass through in 1 minute. That’s 2 cars per minute. Is the rate staying constant as more cars pass through? Well, 4 cars pass every 2 minutes and that reduces to the same unit rate of 2 cars per minute. Finally, 6 cars in 3 minutes also reduces to 2 cars per minute. Cars and minutes are proportional because the rates we calculated from these ordered pairs all reduced to the same, constant value. Therefore, we can call this number a constant of proportionality. It's a constant which represents the relationship between cars and minutes. With this constant, we can write an equation to determine how long it’ll take for Jackson to pass through. Let’s call the number of minutes 'm' and the number of cars 'c'. Notice that multiplying the number of minutes by 2 gets us the number of cars! That means 'c' equals 2 times 'm', or 2 'm'. The constant of proportionality is in our equation! It shows us the proportional relationship between two quantities. In this case cars and minutes. Whenever there is a proportional relationship between two variables, we can write an equation where the coefficient is the constant of proportionality. This equation can help us solve problems, and Jackson needs one solved right away! How long before he passes the gate? Remember, he’s the 30th car. We know 'c', and we want to find 'm'. Substitute 30 for 'c', and solve for 'm'. Dividing both sides by 2 gives us 'm' equals 15! It’ll take him 15 minutes to pass through. He's parked his car and made it into the mall but he's only got 5 minutes left until the store opens. The mall seems to be a maze of escalators he needs to navigate to Game Another Day! Each escalator takes 15 seconds to ride. And there are 12 escalators! What’s the unit rate? That’s right! It's 15 seconds per escalator! Remember, the unit rate is the constant of proportionality, which we represent with k. A short table will help you visualize the equation we need to write. Let’s use the variable 'x' for escalators and 's' for seconds. The unit rate comes from this ratio, 1 to 15. 1 times 15 gets us 15. x' times 15 equals 's', that is, 's' equals 15 'x'. We now have an equation relating seconds to escalators. Remember, Jackson’s got to ride 12 escalators — how long will it take? Substitute 12 for 'x' and we get 's' equals 180 seconds. That’s 3 minutes! Hurry! Jackson’s out of breath, but he’s at the store 2 minutes early. Looks like he's going to get some great deals on some great games. While he waits for the store to open, let's review. The unit rate is found by dividing corresponding values in a ratio table. The unit rate is also called the constant of proportionality, and is often represented by the letter 'k'. An equation in the form 'y' equals 'kx' represents a proportional relationship between 'y' and 'x', where 'k' is the constant of proportionality. These equations are powerful tools for solving problems! Let's check back in with Jackson to see what video games he got. Huh? What's wrong? Where are all the games? Looks like some other shoppers have more experience with Black Friday than Jackson.
cool