Factoring Trinomials with a ≠ 1


Basics on the topic Factoring Trinomials with a ≠ 1
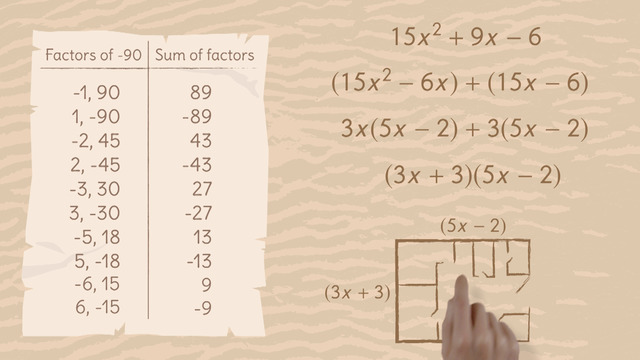
This video lesson will present a more systematic way of factoring trinomials with a≠1; just follow the following steps:
1) Factor out the GCF, if there’s any, and make sure that the trinomial is written in standard form; i.e. ax² + bx + c.
2) Multiply the leading coefficient a and the constant c.
3) Find m and n such that m*n=ac and m+n=b.
4) Rewrite the trinomial by splitting the middle term into two terms; in other words, ax²+bx+c = ax²+mx+nx+c.
5) Group like terms or use the box method.
Let’s look at an example, 12x² + 34x + 10. We have: 1. 12x² + 34x + 10 = 2 (6x² + 17x + 5)
a=6 and c=5. So 6x5=30.
15x2=30. So m=15 and n=2.
2 (6x2 + 17x + 5) = 2 (6x2 + 15x + 2x + 5) = 2 [(6x2 + 15x) + (2x + 5)] = 2[3x(2x + 5) + 1(2x + 5)] = 2(2x + 5)(3x + 1)
Factor a quadratic expression to reveal the zeros of the function it defines.
CCSS.MATH.CONTENT.HSA.SSE.B.3.A
Transcript Factoring Trinomials with a ≠ 1
Today is a big day for Samuel. He wants to join the baseball team, but since he's not a real big athlete he uses his engineering skills to figure out a way to make the team.
Samuel developed a special pair of glasses to analyze his opponents. He uses factoring trinomials to figure out when the ball will hit the ground. Before the player hits the ball, he gets a function of the flight path of the ball to know where it will land. Let's take a look at the view from his glasses. Samuel is standing in right field, ready for anything.
Analyzing the Function
The batter hits the ball with this function: h(x) = -5x² + 14x + 3, where h(x) represents the height of the ball in feet and 'x' represents the time in seconds. We are looking for h(x) = 0, or when the ball hits the ground. Hmm this is not easy to solve, but Sammy's special spectacles can factor things in a jiffy.
As you know, the standard form of a quadratic function is y = ax² + bx + c. In this problem, a = -5, b = 14, and c = 3. First, we have to find the product of a and c, which is -5 times 3, which equals -15. Now, we have to find the factor pairs of -15 (since -15 is negative, only one of the factors should be negative).
Let's think of the possible factors of -15. The factors we have are -1(15), 1(-15), -3(5), and 3(-5). These are the only possible factor pairs of -15. Next, we want to find the pair that sums to b, which, in our case is positive 14. Let's look at the sum of these factors. Remember, we're looking for 14 as the result. The first combination of -1 and 15 equals 14. It looks like we've found the correct factor pair.
Factorization of the trinomial term
To factor this quadratic function, where the right side is a trinomial with 'a ≠ 1', we use the box method. We fill the box with the terms of the quadratic function. Generally, we place the first term in the upper-left-hand corner and the last term in the lower-right-hand corner. Now that we know that 14x is equal to -1x + 15x, we can complete the box with these terms in the other two corners.
We need to find the greatest common factor for each row. For the top row, the greatest common factor is -1x. For the bottom row, the greatest common factor is 3. Next we need to find the greatest common factor for each column. For the first column, the greatest common factor is 5x and 1 for the second. The factorization of the trinomial term is the product of the GCF column sum (5x + 1) and the GCF row sum (-1x + 3) giving us (5x + 1)(-x + 3). We can write our trinomial as a product of two binomials.
Setting h(x)=0
Because we want to know where the ball hits the ground, we set h(x) = 0 because 'h' represents the height of the ball. The equations 5x + 1 = 0 and -x + 3 = 0 represent the two times the ball will hit the ground. The first step is to subtract the integer value from both sides of the equation. The next step is to divide by the coefficient in front of 'x' on both sides of the equation. The solutions to the two equations are -1/5 and 3. We can't use -1/5 because we can't measure time with negative values, so the ball will hit the ground after 3 seconds.
With the help of his glasses, Samuel is in position at the correct time. But maybe he should practice catching a little more...
Factoring Trinomials with a ≠ 1 exercise
-
Factor the quadratic function: $h(x) = 2x^2 + 4x - 6$.
HintsBefore applying the box method, replace $b$ with a sum of two factors of $ac$.
Fill in a box with terms from the quadratic function and then find the greatest common factors of the columns and rows.
SolutionWhen presented with a quadratic function in standard form, the first step in factoring is to identify its coefficients; i.e. $a$, $b$, and $c$.
We then need to find the product of $a$ and $c$, and then find the pair of factors of this product which sum to $b$.
For example, with the equation $h(x) = 2x^2 + 4x - 6$, we have that $a = 2$, $b = 4$, and $c = -6$.
Calculating the product of $a$ and $c$ gives us $ac = 2(-6) = -12$.
So we need to find a pair of factors of $-12$ that sums to $4$.
The pairs of factors of -12 are:
$\begin{array}{rcrcr} 1 &\times & -12 &=& -12 \\ -1 & \times &12 &=& -12 \\ 2& \times & -6& =& -12 \\ -2& \times &6 &=& -12 \\ 3& \times & -4 &=& -12 \\ -3 & \times & 4 &=& -12 \end{array}$
Summing each factor pair, we get:
$\begin{array}{rcrcr} 1 & + & -12 &=& -11 \\ -1 &+& 12 &=& -11 \\ 2 &+& -6 &=& -4 \\ -2 &+& 6 &=& 4 \\ 3 &+& -4 &=& -1 \\ -3 &+& 4 &=& 1 \end{array}$
The factor pair that sums to $b=4$ is $-2$ and $6$. With this pair, we can replace $b$ by their sum in our quadratic function:
$h(x) = 2x^2 - 2x + 6x - 6$.
Then we apply the box method to the trinomial, using the form of our function that has four terms to fill in the quadrants of the box.
We find the greatest common factor (GCF) for each row and each column of the box, and sum the GCFs of the rows and the GCFs of the columns. The product of these sums gives us the factored form of our quadratic equation.
-
Determine when a ball will land by using a quadratic function.
HintsWhat is the height of the ball off the ground, if the ball is touching the ground?
The right hand side of the equation is a product of two terms. When is the product of two terms equal to zero?
Do you think that the flight of the ball is represented by the same function before the batter hits the ball?
If the flight of the ball changes when the batter hits the ball, what does that mean about negative values of time in the equation? Keep in mind that time is equal to zero when the batter hits the ball, so a negative value of time occurs before the batter hits the ball.
SolutionLet's use the example equation given: $h(x) = (5x+1)(-x +3)$.
When the ball hits the ground, its height above the ground will be equal to zero. We can represent this by saying that when the ball hits the ground,
$h(x) = 0$.
In order to find the time that the ball hits the ground, we need to find the value of $x$ that satisfies this equation.
This will occur when $h(x)$ is equal to zero. Since the $h(x)$ is a product of two terms, it will be equal to zero when either (or both) of these terms is equal to zero.
Therefore we can set each of these terms equal to zero and solve for $x$. With the first term, we have
$5x + 1 = 0$.
Subtracting $1$ from both sides, we get
$5x = -1$.
Dividing both sides by $5$, we get:
$5x/5 = -1/5$,
or
$x = -1/5$.
Similarly, with the second expression, we find that $x = 3$.
We know that $h(x)$ is a function representing the height of the ball as a function of time. When the batter hits the ball, its flight changes completely. It doesn't make sense to have a negative value of time in our equation. Because before the batter hits the ball it will have a completely different trajectory that is not described by our function. So we must reject the value of $x$, or time, that is negative. This leaves us with the ball hitting the ground after three seconds.
Whenever applying math to real-life situations it's important to think about your solutions and decide if they are reasonable.
-
Use the box method to factor $h(x)=4x^2 + 7x - 2$.
HintsWhich blanks make the most sense to fill in first?
What pair of factors do you need to find before you can begin to apply the box method?
First, find the pair of factors of $ac$ which sum to $b$. We have that
$ac = 2\times 1 = 2$ and $b = 3$,
so we need the pair of factors of $2$ that sum to $3$.
The pairs of factors of $2$ are
$1$ and $2$, and $-1$ and $-2$.
Summing these pairs give us, $1 + 2 = 3$, and $-1 + (-2) = -3$.
So the pair of factors of $ac$ that sum to $b$ is $1$ and $2$.
SolutionTo apply the box method, we need to know all four terms that fill in the sections of the box. This means that we need to find a pair of factors of $ac$, which sum to $b$, so that we can replace the coefficient $b$ by their sum.
For our particular function, $h(x)=4x^2 + 7x - 2$, we have that $ac = 4\times -2 = -8$.
We must then find a pair of factors for this product that sums to $b$. Let's look at all factor pairs for this product:
$\begin{array}{rcrcr} 2& \times &-4 &=& -8 \\ -2&\times &4 &= &-8 \\ 1&\times &-8 &= &-8 \\ -1&\times &8& = &-8 \end{array}$
We need to find the pair of factors that sums to $b$. We can see that:
$\begin{array}{rcrcr} 2 &+ &-4 &=& -2 \\ -2& + &4 &=& 2 \\ 1& - & 6 & =& -7 \\ -1&+&8& =& 7 \\ \end{array}$
The term $b$ is equal to $7$, so the pair of factors of $ac$ which sum to $b$ is $8$ and $-1$.
This means that our function becomes $h(x) = 4x^2 + 8x - x -2$ and the remaining two terms in our box become $8x$ and $-x$.
We then need to find the greatest common factors of each row and column in our box. Let's put $8x$ in the upper right hand corner, and $-x$ in the lower left hand corner.
The greatest common factor for the top row is $4x$ because this is the largest term that both $4x^2$ and $8x$ are divisible by. Likewise, the greatest common factor (GCF) for the bottom row is $-1$, the GCF for the first column is $x$, and the GCF for the second column is $2$.
Now we find the product of the GCF column sum and the GCF row sum, which gives us the factored form of our function, $h(x) = (4x - 1)(x + 2)$.
-
Factor the quadratic function $h(x) = -3x² + 4x + 4$.
HintsDoes the box method apply to this exercise?
What pair of factors do you need to find before you can apply the box method?
SolutionWe start with the equation, $h(x) = -3x^2 + 4x + 4$.
We need to find its factored form to help Samuel understand when the ball will hit the ground.
The coefficients of $h(x)$'s standard form equation are $a = -3$, $b = 4$, and $c = 4$. The product of $a$ and $c$ is -12. So we know we need to find a pair of factors of $-12$ that sums to $4$. The pair of factors that satisfies these requirements is $6$ and $-2$.
We then replace the coefficient $b$ from the standard form equation with the sum of $6$ and $-2$ and apply the box method using the terms of $h(x)=-3x^2 + 6x -2x + 4$.
We must then find the greatest common factor for each row and column. The greatest common factor (GCF) for the first row is $3x$, because $3x$ is the largest term that both $-3x^2$ and $6x$ are divisible by. Similarly, we find the GCFs of each row and column.
We then find the product of the GCF row sum and the GCF column sum. The product of these sums gives us the factored form of the quadratic equation:
$h(x) =$(sum of the GCF of each row) $\times$ (sum of the GCF of each column)
gives us
$h(x) = (3x + 2)(-x + 2)$.
-
Identify the standard form of a quadratic function.
HintsRemember, the standard form has not been factored.
The standard form is simplified as much as possible.
SolutionThe function $h(x) = ax^2 + bx + c$ is in standard form.
The functions $h(x) = (x-a) (x-b)$ and $h(x) = m (x-a) (x-b)$ are both in factored form. The factored form of a quadratic function provides the values of $x$ at which the function is equal to zero. These are called the roots, and are equal to $a$ and $b$. The second function is a special case of the third function, where $m = 1$.
The function $h(x) = a(x-h)^2 + k$ is in vertex form.
-
Find the factored form of each function written in standard form.
HintsPay close attention to the signs.
Each standard form equation can be factored using the box method.
SolutionLet's use this function $h(x) = 3x^2 + 5x + 2$ as an example.
First, we need to find the pair of factors of $ac$ that sum to $b$. In the case of $h(x)$, we have $ac = 6$ and $b = 5$.
The pairs of factors of $ac$ are:
$\begin{array}{rcrcr} 1& \times &6 &=& 6\\ -1& \times &-6& =& 6\\ 2 &\times &3 &=& 6\\ -2& \times &-3& = &6 \end{array}$
We need the pair of factors that sums to $5$. We can see that
$\begin{array}{rcrcr} 1 &+ &6 &=& 7\\ -1 &+ &-6 &= &-7\\ 2 & + &3 &= &5\\ -2& + &-3 &= &-5 \end{array}$
We than have that $2$ and $3$ is the pair of factors we need. We can now replace the coefficient $b$ with $3 + 2$, giving us the function $h(x) = 3x^2 + 3x +2x + 2$.
Now that we have $h(x)$ written with four terms, we can factor using the box method. First, we fill in the four quadrants of the box, as in the image. Then we find the greatest common factor (GCF) of each row and column.
The greatest common factor of the top row is $3x$, because this is the largest term that divides both $3x^2$ and $3x$. So we write $3x$ off to the left of the top row. Similarly, we find the GCFs of each row and column.
Then we sum the GCFs of the rows and the GCFs of the columns. The product of these sums is our factored function.
So, for our example, summing the row GCFs gives us
$3x+2$,
and summing the column GCFs gives us
$x+1$.
The product of these two sums gives us the factored form of our function:
$h(x) = (3x+2)(x + 1)$.
Similarly, you can find each remaining standard form and factored function pair:
- $h(x) = 3x^2 + 5x + 2 = (3x+2)(x+1)$
- $h(x) = 2x^2 -6x-8 = (2x+2)(x-4)$
- $h(x) = 2x^2 -9x+9 = (2x-3)(x-3)$

Introduction to Polynomials – Naming Polynomials by Number of Terms

Adding Polynomials
Multiplying Polynomials
Multiplying Special Case Polynomials

Factoring out the GCF
Factoring Trinomials with a = 1

Factoring Trinomials with a ≠ 1
Factoring Special Case Polynomials
Factoring by Grouping

Subtracting Polynomials












oof...that's gotta hurt (*o*)