Introduction to Polynomials – Naming Polynomials by Number of Terms


Basics on the topic Introduction to Polynomials – Naming Polynomials by Number of Terms
An algebraic expression is constructed from constants, variables, and the algebraic operations of addition, subtration, multiplication, division, and exponentiation. A polynomial is an algebraic expression where the exponents of variables must be whole numbers, including zero.
Some examples of polynomials are: 5, x, 5y²z, (a + b), (3x – 2y + 5y²z), (a³+ 2b – c + 1). There are four types of polynomials: 1.) monomials: polynomials that have exactly one term (e.g. 5, x, 5y²z), 2.) binomial: polynomials that have two terms (e.g. a + b), 3.) trinomial: polynomials that have three terms (e.g. 3x – 2y + 5y²z), and 4.) multinomial: polynomials that have four or more terms (e.g. a³ + 2b – c + 1).
When working with polynomials, it is important to keep in mind that: 1. All polynomials are algebraic expression but NOT all algebraic expressions are polynomials. 2. A polynomial that is only a variable has a coefficient and exponent that is 1; e.g. x, y, z 3. A polynomial that is a constant has a variable with zero exponent; e.g. 3x0 or 3.
Polynomials, just like numbers, can be simplified by using a set of operations defined on polynomials, which are built from the algebraic operations and rules of arithmetic.
Polynomials are a powerful tool for figuring out the relationships between different patterns. Whether it is finding out how the weather patterns in the Bermuda triangle relate to the weather patterns in Los Angeles, or seeing if the antiaging effects of a drug on mice will tell us something about how the drug will affect humans, polynomials are always involved.
Perform arithmetic operations on polynomials. CCSS.MATH.CONTENT.HSA.APR.A.1
Transcript Introduction to Polynomials – Naming Polynomials by Number of Terms
Today Bella wakes up extra early because she's very excited. Today is the day to plan her Quinceañera, a party to celebrate her 15th birthday. Quinceañeras are a Latin American custom, and they can be lots and lots of fun. Bella is very organized, you know, a real Type A personality. She's working on a list of all the things she needs to do and buy in order to have a fantastic party. She uses polynomials to organize the various costs associated with the party.
Expressing the Cost in a Term
Renting the party space costs is $300 so she writes 300 in her notebook. Invitations cost $4 each, but since she's unsure how many invitations she'll need, she writes 4x to represent the $4 times the unknown number of invites, 'x'. The D.J. charges $50 per hour. Bella hopes the dancing will go on till dawn, but who knows, so she uses the variable 'y' to represent the unknown number of hours and writes 50y. Lastly, but most importantly, is the price of her dress. Bella wants to look like a movie star, and who knows how much that will cost? So she writes the variable 'z' to stand for the unknown dollar amount.
Monomial
Each of these expressions is a term, and a term standing alone is called a monomial. A monomial can be a constant like 300, or a coefficient with a variable--like 4x and 50y. It can also be a variable raised to a positive power like 'z', which is raised to the first power, written simply as 'z'. Remember, all monomials have one thing in common, they all have just the one term.
Binomial
Bella wants to calculate the total cost of the party, so she adds the expenses. Just the cost of the room and the invitations can be represented as 300 + 4x. This expression has two monomials, so it's called a binomial. Get it? Bi means two.
Trinomials
Now add the cost of the D.J., which is 50y The expression now has three terms. You guessed it. This expression is called a trinomial because tri means three.
Polynomials
Next, add in the monomial that represents the cost of the dress, 'z'. 300 + 4x + 5y + z. This expression has more than three terms, so we call it a polynomial. Poly means many.
Summary
Let’s summarize what we've learned so far. There are some simple rules you can follow to help you identify whether or not a term is a monomial. Polynomials are made up of many monomials. Just like all thumbs are fingers, but not all fingers are thumbs, all monomials are terms, but not all terms are monomials.
Monomials are coefficients times a variable or variables that are raised to non-negative, integer exponents. Let's take a look at the chart: Monomials can also be multiplied by negative coefficients, again raised to non-negative, integer exponents, like (-1/3)x². When a variable is raised to the first power, like 1x¹, we don't have to write either number 1, since these are identities, so we just write 'x'. Finally, 5 is really 5 times 'x' raised to the zero power. And since anything raised to the zero power equals one, we're left with the constant. Be careful! Monomials CANNOT have a variable in the denominator or a variable with a negative exponent.
Binomials are made up of two monomials like 4x³ - (⅓)x² or x + 5.
Trinomials are made up of three monomials like 4x³ - (⅓)x² + x and polynomials are any expression made up of one or more monomials, and can also be called binomials or trinomials - depending on how many terms an expression has. As you can see, all the terms are separated by a plus or minus.
Bella has finally finished with her party planning list, and she’s ready to calculate the cost of her party. What’s this? An invitation to Laila Alyssa’s Quinceañera! Are you kidding me! It’s the exact same date that Bella had planned to have her party. Qué horror! Bella is so angry. She’s steaming. She’s hotter than a hot tamale!
Introduction to Polynomials – Naming Polynomials by Number of Terms exercise
-
Identify which of the following statements about monomials are true.
HintsA power is short-hand notation for a product:
$x^n=\underbrace{x\times ...\times x}_{\text{n times}}$.
We can see that $x^1$ has only one factor, $x$.
You can divide two powers with the same base by subtracting their exponents:
${\large\frac{x^{\large 4}}{x^{\large 2}}}=x^{(4-2)}=x^2$.
SolutionMonomials are coefficients times variables raised to non-negative, integer exponents.
$~$
- The variable $x$ itself is a monomial. This is because $x=1x^1$. When a variable is raised to the power of $1$, we don't need to write the exponent.
- Any constant is a monomial. This is because $x$ raised to the power of $0$ is equal to $1$, so $5x^0=5$.
-
Find the term representing the cost of Bella's quinceanera.
HintsKeep the definition of a monomial in mind:
Monomials are coefficients times variables raised to non-negative, integer exponents.
A term consisting of two monomials is called a binomial, and a term consisting of three monomials is called a trinomial.
Constants or variables standing alone are also minomials; for instance, we have:
- $5=5x^0$
- $x=1x^1$
SolutionBella wakes up super early to plan her quinceanera! A very exciting time in a young woman's life.
She starts to put together a list of party expenses:
- The party venue will cost 300 dollars, no question.
- Invitations will cost 4 dollars a pop. Letting $x$ be the number of invitations, the total cost for invitations will be $4x$ all in all.
- The DJ wants 50 dollars per hour. Letting $y$ be the number of hours the DJ will play, she knows that the DJ will cost $50y$ in total.
- Last but not least: Her dress. She absolutely wants to look like a movie star... and there is no telling what the price of looking like fame will be, she just lets $z$ represent the price of her look.
Last she adds all the costs together to get the polynomial, $300+4x+50y+z$.
-
Analyze the following terms.
HintsMonomials are coefficients times a variable or variables that are raised to non-negative, integer exponents.
Binomials are terms with two monomials.
Pay attention: variables raised to negative or rational powers are not monomials.
SolutionMonomials are coefficients times a variable or variables that are raised to non-negative, integer exponents.
The following terms are monomials:
- $34x$
- $-4y^2$
- $123$
Neither $4y^{-2}$ nor $123x^{0.5}$ are monomials, as the exponents aren't positive integers.
$~$
The following terms are binomials, as they are made up of two monomials:
- $34x-4y^2$
- $34x+123$
- $-4y^2+123$
Since $4y^{-2}$ is not a monomial, we know that $34x+4y^{-2}$ cannot be a binomial.
$~$
$34x-4y^2+123$ is a trinomial, so it is neither a binomial nor a monomial. Adding or subtracting some further monomials leads to polynomials. For instance, $4x^3+2x^2-x-6$ is a polynomial as it is a term made up of more than three monomials... so it is also neither a binomial nor a monomial.
-
Establish the polynomial representing the total cost of Bella's next shopping trip.
HintsConstants or variables are monomials.
- A term consisting of two monomials is a binomial.
- A term consisting of three monomials is a trinomial.
- A term consisting of more than three monomials is a polynomial.
SolutionBefore Bella embarks on a day shopping in town, she needs to figure out how much money she will need for the trip!
- She needs $10$ dollars for a bus ticket.
- She plans to buy a new pair of jeans, but doesn't know how much they would cost. So she lets the price be $x$, a variable.
- She then always goes to her favortie ice cream parlor, which sells ice cream at 1.5 dollars a scoop. She's still not sure exactly how many scoops she will want... so she decides to represent the number of scoops she buys by $y$. This gives her a monomial, $1.5y$, representing the cost of the ice cream.
- She also needs three new school notebooks, which cost 2 dollars each... or in other words, $3\times 2=6$ dollars.
-
Decide if the given term is a monomial, binomial, trinomial, or polynomial.
HintsMonomials are coefficients times variables raised to non-negative, integer exponents.
$-\frac13x^2$ is an example of a monomial.
- Mono stands for one.
- Bi stands for two.
- Tri stands for three.
- Poly stands for many.
SolutionMonomials are coefficients times variables raised to non-negative, integer exponents.
- A term of two monomials is a binomial.
- A term of three monomials is a trinomial.
- A term of more than three monomials is a polynomial.
Monomials
- $4x^3$
- $-\frac13x^2$
- $x$
- $5$
- $1$
- $4x^3-\frac13x^2$
- $x+5$
- $4x^3-\frac13x^2+x$
- $4x^3+x+1$
- $4x^3-\frac13x^2+x+5$
- $4x^3-\frac13x^2+x+1$
-
Simplify the monomials and then add them together to make a polynomial.
HintsThink about the meaning of $x^n$:
$x^n=\underbrace{x\times ...\times x}_{\text{n times}}$.
For example, we have that $5x^1=5x$.
SolutionLet's start by simplifying the monomials. We have:
- $1x^5=x^5$
- $22x^0=22\times 1=22$
- $3x^1=3x$
- $1x^3=x^3$
Next we add all of the monomials together to get:
$x^5+22+3x+x^3$.
While writing a sum of monomials in any order you choose is correct, conventionally, such summands are arranged from highest to lowest degree:
$x^5+x^3+3x+22$.

Introduction to Polynomials – Naming Polynomials by Number of Terms

Adding Polynomials
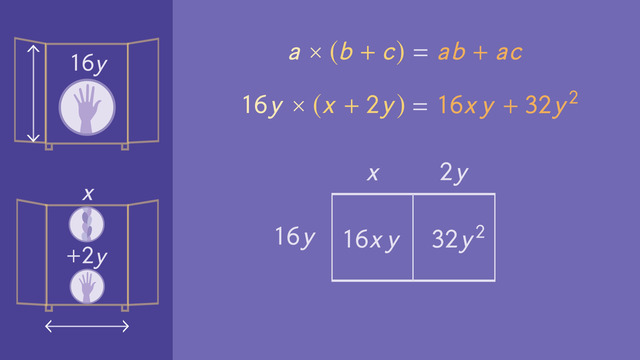
Multiplying Polynomials
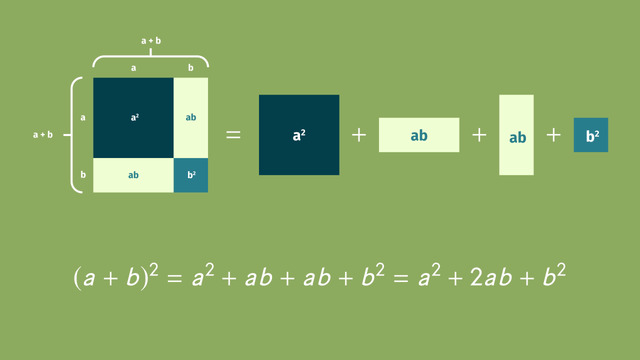
Multiplying Special Case Polynomials

Factoring out the GCF
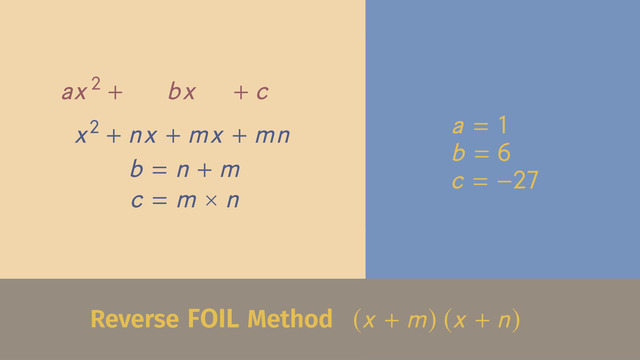
Factoring Trinomials with a = 1

Factoring Trinomials with a ≠ 1
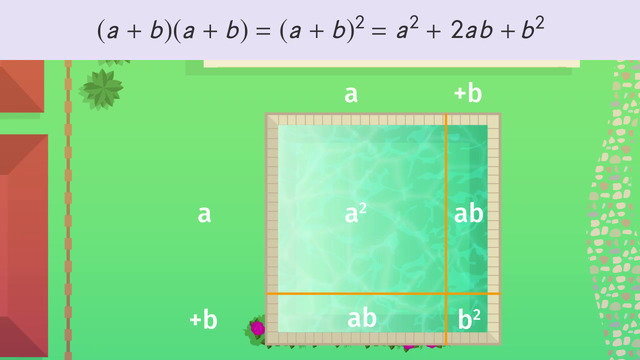
Factoring Special Case Polynomials
Factoring by Grouping

Subtracting Polynomials












YASSSSSSS QUEENNNNNNN!!!!!!
yo