Factoring Trinomials with a = 1

Basics on the topic Factoring Trinomials with a = 1
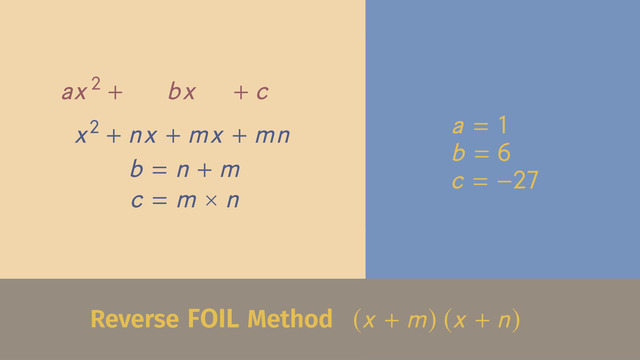
Before you factor a trinomial, a polynomial with only three terms, write it in the standard form of ax² + bx + c. Then, take a look at the leading coefficient. If the leading coefficient, the coefficient to a, is equal to one, you can use the reverse FOIL method to factor the trinomial as two binomials: (x + m)(x + n) which is equal to x² + nx + mx + mn and the same as x² + x(m + n) + mn. Compare this polynomial to the standard form: ax² + bx + c, and you can see the sum of m and n is equal to b and the product of m and n is equal to c.
To figure out how to write the binomial pair, list the factors of c. Most likely the list is not that long. Next, determine which pair of factors will sum to b, and then use the two factors to write the two binomials: (x + m)(x + n). Always use FOIL to verify your answer because it’s easy to make careless mistakes, especially when negative numbers are used.
To help you understand this process, and make it easy for you to factor trinomials with the leading coefficient equal to one, sit back, relax, and enjoy watching this video - while you learn at the same time.
Understand the relationship between zeros and factors of polynomials.
CCSS.MATH.CONTENT.HSA.APR.B.2
Transcript Factoring Trinomials with a = 1
Captain Johnny Redbeard and his parrot Holly are hunting for treasure. Redbeard has heard many stories about the riches to be found on Treasure Island. Legend says that there's a chest no man can open. It has a lock impervious to tools of all kinds and only the right combination of numbers will reveal the treasure hidden inside.
Factoring Trinomials
Eager to take on the challenge, Redbeard quickly sets course for Treasure Island. Taking a closer look at the map, Redbeard, sometimes referred to as the math expert of the seven seas, realizes that the treasure is not only marked by x, but also with a polynomial. He knows just what to do, he needs to factor the trinomial.
To open the treasure chest, Captain Johnny Redbeard has to find the correct set of binomials terms that, when multiplied, equal the the expression on the map, x²+6x-27. So he needs to factor the expression.A standard trinomial is in the form ax² + bx + c. In this puzzle, a = 1, b = 6 and c = -27."
Reverse FOIL Method
Captain Johnny knows the reverse FOIL method to factor trinomials. Let's think of the two binonmials we're looking for as (x+m)(x+n). Remember, if we multiply these binomials using FOIL, we get x²+nx+mx+mn. If we compare this expression with the standard form, 'b' must be the sum of n+m whereas 'c' must be the product of 'm' and 'n'. Remember, you can use the Commutative Property for sums and products.
So, Captain Johnny Redbeard has to find exactly two numbers that follow these rules: The sum of the factors has to be 'b', in this case, 6. And also, the product of the same two numbers has to be 'c', in this case -27. There can't be that many options, but how exactly can we figure out the right numbers? Because there are fewer options for multiplication, let's start with that. Let's think of the possible factors of -27. The factors we have are 1 times (-27), -1 times (27), 3 times (-9), and -3 times (9). These are the only possible values for 'm' and 'n' here.
Let's look at the sum of these factors. Remember, we're looking for 6 as the result. Both 1 + -27 and -1 + 27 are not equal to 6. 3 + -9 = -6 also not equal to 6. So now we check our last combination -3 + 9 = 6. We've found our correct 'm' and 'n' values! We know that -3 and 9 are our 'm' and 'n' values, where m = -3 and n = 9. We can plug these into our parentheses as the factors of x² + 6x - 27. Our factors are (x - 3) and (x + 9).
Well, shiver me timbers! Captain Johnny Redbeard managed to solve the riddle, which no man was able to do before. As he enters the factors into lock on the treasure chest the treasure chest reveals. BLIMEY!!! another map with more difficult looking expressions but at least he's good at math! Weigh anchor and hoist the mizzen! ...er...something like that...anyway, he's off on another adventure!
Factoring Trinomials with a = 1 exercise
-
Explain how Johnny Redbeard can factor the trinomial $x^2+6x-27$.
HintsTo multiply two binomials like $(x + m)(x + n)$, we use:
- First - multiply the first terms: $x$ times $x$
- Outer - multiply the outer terms: $x$ times $n$
- Inner - multiply the inner terms: $m$ times $x$
- Last - multiply the last terms: $m$ times $n$
In this example, $a=3$, $b=4$ and $c=5$.
You are looking for two numbers, $m$ and $n$, that sum up to $b$ and whose product equals $ac$.
SolutionCaptain Johnny Redbeard, is often referred to as the math expert of the seven seas.
He realized he has to factorize the trinomial $x^2 + 6x - 27$ in order to open the treasure chest.
$x^2 + 6x - 27$ is a special case of the standard form $ax^2 + bx + c$. Here we have $a=1$, $b=6$ and $c=-27$.
The factorization of this polynomial will look like $(x + m)(x + n)$.
Using the FOIL method we get the following:
- First: $x\times x=x^2$
- Outer: $x\times n=nx$
- Inner: $m\times x=mx$
- Last: $m\times n=mn$
Comparing this with $x^2 + 6x - 27$, we have to find two numbers, $m$ and $n$, whose sum equals $6$ and whose product equals $-27$.
-
Find all possible factors of $-27$ and add them together to identify $m$ and $n$.
HintsThe factors of $-27$ are $\pm 1$, $\pm 3$, $\pm9$ and $\pm 27$.
SolutionWe've provided a table of the factors of $-27$ and the sum of the factors of $-27$.
Captain Johnny Readbeard already knows he is looking for two numbers, $m$ and $n$ that, when added together, equal $6$ and whose product is $-27$. There can't be that many options.
When given these kinds of problems, always start by figuring out the factors so that there are fewer possibilities. Then, write all combinations of the factors in a table.
Next, calculate the sum of each of the factor pairs. For Johnny Redbeard's treasure, the sum has to be $6$.
Taking a closer look at the table, there is only one possible combination which satisfies both requirements: $m=-3$ and $n=9$ added together equal $6$ and their product is $-27$.
So, the trinomial $x^2+6x-27$ can be factored as $(x-3) (x+9)$.
-
Assign each polynomial to its corresponding factorization.
HintsKeep the reverse FOIL method in mind:
$(x+m)(x+n)=x^2+(m+n)x+mn$
The coefficient of $x$ is $m+n$ and the constant term is $mn$.
First, look at the constant term and determine all possible factors, including the negative ones.
SolutionAll given sets of binomials and trinomials look similar. Pay attention to their signs.
First, we need the reverse FOIL method, $(x+m)(x+n)=x^2+(m+n)x+mn$.
$~$
$x^2-x-6$
- Here we have $mn=-6$. The possible factors of $-6$ are: $\pm 1$, $\pm 2$, $\pm 3$, and $\pm 6$.
- We also know that $m+n=-1$.
- We then see that the only pair of factors that satisfy both requirements is $m=2$ and $n=-3$.
- So $x^2-x-6=(x+2)(x-3)$
$x^2-5x+6$
- Here, we have $mn=6$. The possible factors of $6$ are $\pm 1$, $\pm 2$, $\pm 3$, and $\pm 6$.
- We also know that $m+n=-1$.
- We then see that the only pair of factors that satisfy both requirements is $m=-2$ and $n=-3$.
- So $x^2-5x+6=(x-2)(x-3)$
$x^2+5x+6$
- Here, we have $mn=6$ and the factors are $\pm 1$, $\pm 2$, $\pm 3$, and $\pm 6$.
- We also know that $m+n=5$.
- We then see that the only pair that satisfies these requirements is $m=2$ and $n=3$.
- So $x^2+5x+6=(x+2)(x+3)$
$x^2+x-6$
- Here, we have $mn=-6$, so the possible factors of $-6$ are $\pm 1$, $\pm 2$, $\pm 3$, and $\pm 6$.
- We also know that $m+n=1$.
- We then see that the only pair that satisfies this is $m=-2$ and $n=3$.
- So $x^2+x-6=(x-2)(x+3)$
-
Factor the given polynomials in order to open the treasure chests.
HintsWrite each term in this form:
$(x+m)(x+n)$
Let's look at an example:
$x^2-5x-6=(x-6)(x+1)=(x+1)(x-6)$
The product of $m$ and $n$ is the constant of the polynomial and the sum of $m$ and $n$ the coefficient of $x$.
SolutionFor each given polynomial, we look at the constant term first. Keep in mind that $mn=c$, where $c$ is the constant term. So you have to find all possible factors.
Then, we check for each possible combination of factors if $m$ and $n$ also satisfy the second requirement, $m+n$ is equal to the coefficient of $x$.
$~$
$x^2+8x+12$
- Here, $mn=12$
- The possible factors of $12$ are $\pm 1$, $\pm 2$, $\pm 3$, $\pm 4$, $\pm 6$, and $\pm 12$
- To get the sum $m+n=8$, we have $m=2$ and $n=6$ or, according to the Commutative Property, $m=6$ and $n=2$
- So $x^2+8x+12=(x+2)(x+6)=(x+6)(x+2)$
$x^2-x-12$
- $mn=-12$
- The possible factors of $-12$ are $\pm 1$, $\pm 2$, $\pm 3$, $\pm 4$, $\pm 6$, and $\pm 12$
- To get the sum $m+n=-1$, we have $m=3$ and $n=-4$. It could be the other way around, but here the $+$ is already given for the first binomial
- So $x^2-x+12=(x+3)(x-4)$
$x^2-11x+24$
- $mn=24$
- The possible factors are $\pm 1$, $\pm 2$, $\pm 3$, $\pm 4$, $\pm 6$, $\pm 8$, $\pm 12$, and $\pm 24$
- To get the sum $m+n=-11$, we have $m=-8$ and $n=-3$ or, according to the Commutative Property, $m=-3$ and $n=-8$
- So $x^2-11x+24=(x-8)(x-3)=(x-3)(x-8)$
-
Describe the FOIL method for multiplying binomials.
HintsPay attention to the signs.
FOIL is the mnemonic used to remember how to multiply two binomials.
Keep in mind that the result of the multiplication above is
$x^2+6x-27$
SolutionFOIL multiplication stands for
- First - multiply the both first terms $x\times x=x^2$
- Outer - multiply the outer terms $x\times 9=9x$
- Inner - multiply the inner terms $-3\times x=-3x$
- Last - multiply the last terms $-3\times 9=-27$
$\begin{array}{rcl} (x-3)(x+9) &=& \\ x^2 + 9x - 3x - 27 &=& \\ &=& x^2+6x-27 \end{array}$
-
Write each trinomial as a product of two binomials by factoring.
HintsThe reverse FOIL method tells us that
$m+n=b$ and $mn=c$
First, determine all combinations $m$ and $n$ that satisfy $mn=c$.
Products are commutative, so instead of $(x-3)(x+2)$, you can also write $(x+2)(x-3)$.
SolutionWhen factoring polynomials, we can use the reverse FOIL method to help us more easily solve the equation, $x^2+bx+c=(x+m)(x+n)$:
- First, look at the constant $c$. We must find all possible factors, $m$ and $n$, of $c$.
- Check each combination of $m$ and $n$ that satisfies $mn=c$. Don't forget the negative factors.
- $m$ and $n$ also have to satisfy $m+n=b$.
$x^2+17x+70$
- Looking at the constant term, we know $mn=70$.
- We also know that $m+n$ has to equal $b$. For example, $2\times 35=70$, but $2+35=37\neq 17$.
- Another example is $7\times 10=70$; adding these factors gives us $7+10=17$.
- We can conclude that $x^2+17x+70=(x+7)(x+10)=(x+10)(x+7)$
$x^2-19x+84$
- Looking at the constant term, we know $mn=84$.
- We know that $m+n$ has to equal $b$. For example, $12\times 7=84$, but $12+7=19\neq -19$.
- Let's check. $-12\times -7=84$. Now we get $-12+-7=-19$.
- We can conclude that $x^2-19x+84=(x-12)(x-7)=(x-7)(x-12)$
$x^2+4x-96$
- Here, we have $c=-96$ and so $mn=-96$. So we have either $m=\pm$ and $n=\mp 96$ or $m=\pm 2$ and $n=\mp 48$ ...
- Because $m+n=4$, we have to look at a special combination of $m$ and $n$. In this combination, the difference between the absolute values of $m$ and $n$ must be $4$.
- Applying these rules leads us to $m=\pm12$ and $n=\mp8$.
- $-12\times 8=-96$ and $-12+8=-4$... that can't be the solution.
- $12\times -8=-96$ and $12+-8=4$ $\surd$
- So $x^2+4x-96=(x+12)(x-8)=(x-8)(x+12)$

Introduction to Polynomials – Naming Polynomials by Number of Terms

Adding Polynomials
Multiplying Polynomials
Multiplying Special Case Polynomials

Factoring out the GCF

Factoring Trinomials with a = 1

Factoring Trinomials with a ≠ 1
Factoring Special Case Polynomials
Factoring by Grouping

Subtracting Polynomials












That parrot is real big.