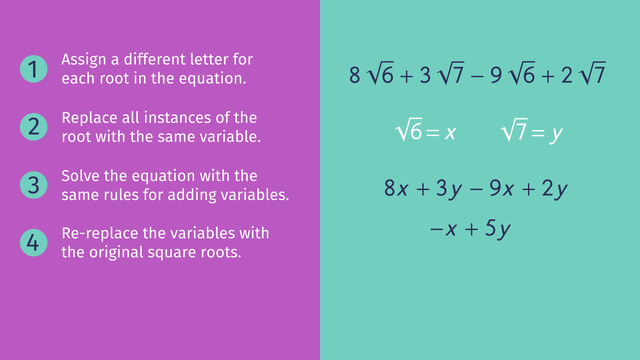Multiplying Radical Expressions

Basics on the topic Multiplying Radical Expressions
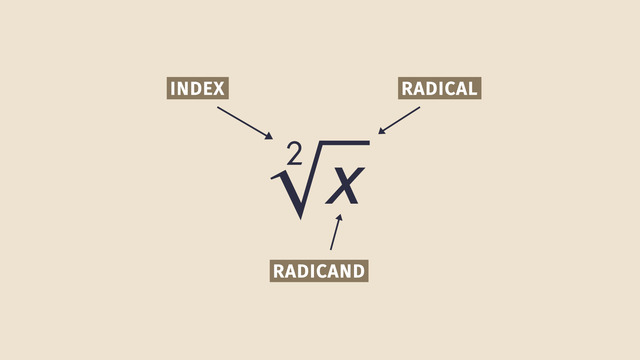
When multiplying radical expressions, it is important to check if the given radicals have the same index (the number at the upper left of the radical symbol) or not. This is because the only radicals which can be simplified further when multiplied together are those which have the same index.
To multiply radical expressions with the same index just separately multiply their radicands (the number inside the radical symbol) and coefficients (the number outside the radical symbol). Then simplify and combine the two products if possible.
For example, consider the radicals (3√2) and (5√8). When multiplying the two, you get 15√16, since 16 is a perfect square whose square root is 4. Then multiplying 4 by 15 gives a final answer of 60.
Expressions and Equations Work with radicals and integer exponents.
CCSS.MATH.CONTENT.8.EE.A.2
Transcript Multiplying Radical Expressions
We join our hero, Snipe, after scaling the Mushroom Mountain, running through the Mushroom Forest and searching for the Mushroom Castle. His journey is almost complete. He needs one more piece to complete the door of wisdom. Snipe has traversed Mushroom Mountain and has finally collected all the lost pieces he needs to enter the Mushroom Castle. He only gets one shot at correctly completing the door of wisdom. Otherwise, something bad will happen. To do this, he must know how to multiply radical expressions.
Multiplying radical expressions
Snipe opens his bag to reveal the stones he’s collected on his journey. If he’s able to open the door of wisdom, he can prove he’s worthy of the riches inside. Each of the stones he collected has markings that look like mathematical equations. If he’s able to solve these equations, maybe they’ll tell him which one belongs in the door of wisdom. Snipe looks at the door and then down at his keys. Surely one of these will open the Door of Wisdom. Snipe examines the first key.
Since both the expressions are in parentheses, Snipe decides to FOIL them. First, Outer, Inner, Last. Snipe multiplies the factors outside the radicals first. He then uses the Multiplication Property of Radicals to simplify the factors under the radical. He does this for all terms, then he combining the like terms, removing any squares from under the radicals and finally, once again combining like terms gives us ew. This is kind of a messy answer. Maybe our hero'll have better luck with the other keys.
First we FOIL until we can't FOIL no mo', then combine like terms. But there are none, so we move on to the next step and remove any squares from under the radicals. Here, you don't see any squares at first glance, but 18 can be factored into 2 and 9, which is a perfect square.
Using the Multiplication Property of Radicals in reverse, we split the radical in two and take the square root of 9. A bit of PEMDAS and that’s the simplified version? This is definetely not the right key. Last key, and the last hope for our hero. FOIL, just like the others: 1. Combine like terms once again 2. Remove any squares from under the radicals
This is looking quite promising! And Done! Woohoo! Behold! The key that unlocks the Door of Wisdom! Snipe identifies the shape needed to open the door and carefully slides it into place.
Multiplying Radical Expressions exercise
-
Explain how to multiply $(15\sqrt5+5\sqrt3)(6\sqrt3-2\sqrt5)$.
HintsFOIL is as way of remembering a method of multiplying two binomials:
- Multiply the First.
- Multiply the Outer.
- Multiply the Inner.
- Multiply the Last.
Here is an example of multiplication using the FOIL method:
$(3+b)(2+b)=6+3b+2b+b^2$
Now you can combine like terms to get:
$6+3b+2b+b^2=6+5b+b^2$
SolutionTo multiply $(15\sqrt5+5\sqrt3)(6\sqrt3-2\sqrt5)$, first use the FOIL method to multiply these binomials:
- F multiply the first $15\sqrt5(6\sqrt3)$
- O multiply the outer $-15\sqrt5(2\sqrt5)$
- I multiply the inner $5\sqrt3(6\sqrt3)$
- L multiply the last $-5\sqrt3(2\sqrt5)$
$(15\sqrt5+5\sqrt3)(6\sqrt3-2\sqrt5)=15\sqrt5(6\sqrt3)-15\sqrt5(2\sqrt5)+5\sqrt3(6\sqrt3)-5\sqrt3(2\sqrt5)$.
Multiply the radicals and combine like terms:
$\begin{array}{rcl} (15\sqrt5+5\sqrt3)(6\sqrt3-2\sqrt5)&=&~90\sqrt{15}-30\sqrt{25}+30\sqrt9-10\sqrt{15}\\ &=&~90\sqrt{15}-10\sqrt{15}-150+90\\ &=&~80\sqrt{15}-60 \end{array}$
There is no further simplification possible, so we have our answer.
-
Calculate $(15\sqrt5+5\sqrt3)(6\sqrt5-2\sqrt3)$.
HintsHere is an example for using the FOIL method:
$\begin{array}{rcl} (3+a)(2+2a)&=&~6+3(2a)+2a+a(2a)\\ &=&~6+6a+2a+2a^2\\ &=&~2a^2+8a+6 \end{array}$
Keep in mind that the square root of a square is equal to the term being squared:
$\sqrt{a^2}=a$.
The square root of a product is the product of the square roots of its factors.
SolutionTo multiply $(15\sqrt5+5\sqrt3)(6\sqrt5-2\sqrt3)$, first use the FOIL method to multiply these binomials:
- F multiply the first $15\sqrt5(6\sqrt5)$
- O multiply the outer $-15\sqrt5(2\sqrt3)$
- I multiply the inner $5\sqrt3(6\sqrt5)$
- L multiply the last $-5\sqrt3(2\sqrt3)$
$(15\sqrt5+5\sqrt3)(6\sqrt5-2\sqrt3)=15\sqrt5(6\sqrt5)-15\sqrt5(2\sqrt3)+5\sqrt3(6\sqrt5)-5\sqrt3(2\sqrt3)$.
We multiply the radicals and combine the like terms to get:
$(15\sqrt5+5\sqrt3)(6\sqrt5-2\sqrt3)=90\sqrt{25}-30\sqrt{15}+30\sqrt{15}-10\sqrt9$.
Now we can simplify the radicals and cancel out the term $-30\sqrt{15}$ with $30\sqrt{15}$, giving us that
$(15\sqrt5+5\sqrt3)(6\sqrt5-2\sqrt3)=450-30=420$.
-
Identify the steps of multiplication.
Hints- F multiply the first $3\sqrt3(4\sqrt3)$
- O multiply the outer $-3\sqrt3(2\sqrt2)$
- I multiply the inner $5\sqrt2(4\sqrt3)$
- L multiply the last $-5\sqrt2(2\sqrt2)$
Here you see an example using the FOIL method.
The second step in the example above is combining like terms:
$5a-2a=3a$.
SolutionUsing the FOIL method we get
$(3\sqrt3+5\sqrt2)(4\sqrt3-2\sqrt2)=3\sqrt3(4\sqrt3)-3\sqrt3(2\sqrt2)+5\sqrt2(4\sqrt3)-5\sqrt2(2\sqrt2)$.
We multiply the radicals,
$(3\sqrt3+5\sqrt2)(4\sqrt3-2\sqrt2)=12\sqrt9-6\sqrt6+20\sqrt6-10\sqrt4$,
and combine the like terms,
$(3\sqrt3+5\sqrt2)(4\sqrt3-2\sqrt2)=12\sqrt9+14\sqrt6-10\sqrt4$.
Now we calculate the square roots of squares:
- $\sqrt9=\sqrt{3^2}=3$
- $\sqrt4=\sqrt{2^2}=2$
And finally we combine like terms again to get
$(3\sqrt3+5\sqrt2)(4\sqrt3-2\sqrt2)=36+14\sqrt6-20=16+14\sqrt6$.
-
Calculate the product.
Hints- F multiply the first $12\sqrt6(12\sqrt6)$
- O multiply the outer $12\sqrt6(3\sqrt5)$
- I multiply the inner $-3\sqrt5(12\sqrt6)$
- L multiply the last $-3\sqrt5(3\sqrt5)$
You still have to add the terms calculated using the FOIL method together.
Keep the following in mind that $\sqrt{a^2}=a$. For example:
SolutionWe use the FOIL method first to get
$(12\sqrt6-3\sqrt5)(12\sqrt6+3\sqrt5)=12\sqrt6(12\sqrt6)+12\sqrt6(3\sqrt5)-3\sqrt6(12\sqrt6)-3\sqrt5(3\sqrt5)$.
Next we multiply the radicals and combine like terms:
$(12\sqrt6-3\sqrt5)(12\sqrt6+3\sqrt5)=144\sqrt{36}+36\sqrt{30}-36\sqrt{30}-9\sqrt{25}$.
Once again, combining like terms and calculating the radicals of squares we get:
$(12\sqrt6-3\sqrt5)(12\sqrt6+3\sqrt5)=144(6)-9(5)=864-45=819$.
-
Decide which expressions can be simplified.
HintsYou can only combine like terms.
For example, $5\sqrt 3+6\sqrt3=11\sqrt3$, but you can't combine $5\sqrt 3+6\sqrt5$.
For example:
$\begin{array}{rcl} 2\sqrt5(5\sqrt3)&=&~2\times 5\times\sqrt5\times \sqrt3\\ &=&~10\times\sqrt{5\times 3}\\ &=&~10\times\sqrt{15} \end{array}$
There are just three terms which can be simplified.
SolutionCombining like terms means that you only can add or subtract terms with the same variable and exponent:
- $a+a=2a$
- $a^2+a^2=2a^2$
Let's have a look at the following example:
$(15\sqrt5+5\sqrt3)(3\sqrt2-5\sqrt6)$.
First we use the FOIL method:
- F multiply the first $15\sqrt5(3\sqrt2)$
- O multiply the outer $-15\sqrt5(5\sqrt6)$
- I multiply the inner $5\sqrt3(3\sqrt2)$
- L multiply the last $-5\sqrt3(5\sqrt6)$
$(15\sqrt5+5\sqrt3)(3\sqrt2-5\sqrt6)=15\sqrt5(3\sqrt2)-15\sqrt5(5\sqrt6)+5\sqrt3(3\sqrt2)-5\sqrt3(5\sqrt6)$.
The radicals can be multiplied by multiplying the terms under the square roots:
- $\sqrt5(\sqrt2)=\sqrt{10}$
- $\sqrt5(\sqrt6)=\sqrt{30}$
- $\sqrt3(\sqrt2)=\sqrt{6}$
- $\sqrt3(\sqrt6)=\sqrt{18}$
We are still able to simplify $\sqrt{18}=\sqrt{9\times 2}=\sqrt9\times\sqrt2=3\sqrt2$ further.
This together with the expression above gives us:
$(15\sqrt5+5\sqrt3)(3\sqrt2-5\sqrt6)=45\sqrt{10}-75\sqrt{30}+15\sqrt6-75\sqrt{2}$
And that's it! We have simplified the expression as much as we can.
-
Find the errors in the calculation.
HintsEvery calculation has an error.
The result of the first multiplication is $-7\sqrt{10}+3$.
Keep in mind that you can only combine like terms.
Here is an example for multiplying radicals:
$\begin{array}{rcl} \sqrt 3\times \sqrt2&=&~\sqrt{3\times 2}=\sqrt6\\\\ \sqrt{18}&=&~\sqrt{9\times 2}=\sqrt9\times \sqrt 2=3\sqrt2 \end{array}$
SolutionLet's first outline the steps for multiplying expressions with radicals:
- Use the FOIL method.
- Rearrange the terms and combine like terms. Remember that $\sqrt a\times \sqrt b=\sqrt{a\times b}$.
- Calculate the radicals if it's possible; for example $\sqrt 4=\sqrt{2^2}=2$.
- Simplify the result as much as possible by combining like terms.
$~$
For $(\sqrt5-3\sqrt2)(2\sqrt2+3\sqrt5)$:
First use the FOIL method:
$(\sqrt5-3\sqrt2)(2\sqrt2+3\sqrt5)=\sqrt5(2\sqrt2)+\sqrt5(3\sqrt5)-3\sqrt2(2\sqrt2)-3\sqrt2(3\sqrt5)$.
Second rearrange the terms and combine like terms:
$\begin{array}{rcl} ((\sqrt5-3\sqrt2)(2\sqrt2+3\sqrt5)&=&~2\sqrt{10}+3\sqrt{25}-6\sqrt4-9\sqrt{10}\\ &=&~2\sqrt{10}-9\sqrt{10}+3\sqrt{25}-6\sqrt4\\ &=&~-7\sqrt{10}+3\sqrt{25}-6\sqrt4. \end{array}$
Third calculate the radicals if possible: $(\sqrt5-3\sqrt2)(2\sqrt2+3\sqrt5)=-7\sqrt{10}+3(5)-6(2)$.
Fourth simplify the result as much as possible: $(\sqrt5-3\sqrt2)(2\sqrt2+3\sqrt5) =-7\sqrt{10}+15-12=-7\sqrt{10}+3$.
$~$
For $(2\sqrt6+3\sqrt5)(3\sqrt6-5\sqrt3)$:
- $(2\sqrt6+3\sqrt5)(3\sqrt6-5\sqrt3)=2\sqrt6(3\sqrt6)-2\sqrt6(5\sqrt3)+3\sqrt5(3\sqrt6)-3\sqrt5(5\sqrt3)$
- $(2\sqrt6+3\sqrt5)(3\sqrt6-5\sqrt3)=6\sqrt{36}-10\sqrt{18}+9\sqrt{30}-15\sqrt{15}=6\sqrt{36}-30\sqrt{2}+9\sqrt{30}-15\sqrt{15}$
- $(2\sqrt6+3\sqrt5)(3\sqrt6-5\sqrt3)=6(6)-30\sqrt{2}+9\sqrt{30}-15\sqrt{15}=36-30\sqrt{2}+9\sqrt{30}-15\sqrt{15}$
- There is no further simplification possible.