Factoring Special Case Polynomials

Basics on the topic Factoring Special Case Polynomials
Writing and factoring polynomials can have special cases. Learning to recognize these special cases is definitely worth your time because it may save you some hair pulling later on.
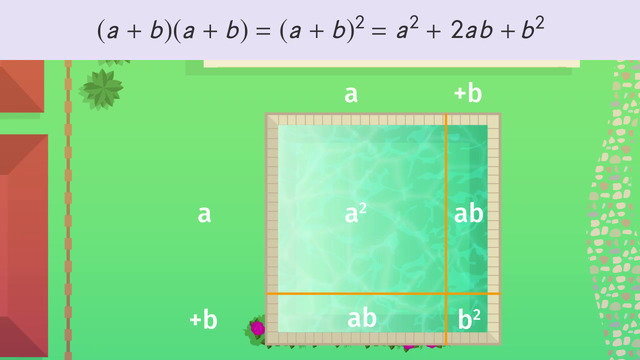
First is the square of binomials sums: (a + b)² = (a + b)(a + b) = a² + 2ab + b².
Similar to this case is the square of binomial differences: (a – b)² = (a – b)(a – b) = a² – 2ab + b².
The third special case is the difference of two squares: (a + b)(a – b) = a² – b².
Pay attention to the patterns of these three special cases and rather than spending time factoring, foiling, and distributing the terms, you will be doing a victory dance to celebrate how well you are doing in your Algebra class.
For example, if your teacher assigns you to factor this problem: x⁴ – 49. Whoa, this looks very difficult. Relax – it’s just the difference of two squares or a DOTS problem. (x²)² is equal to x⁴, and 7² is equal to 49, so to factor the difference of the two squares: (x² – 7) (x² + 7), and when you foil or use the distributive property, you are right back where you started: x⁴ – 49.
To see more examples of special case polynomials and have a laugh too, watch this video.
Understand the relationship between zeros and factors of polynomials.
CCSS.MATH.CONTENT.HSA.APR.B.2
Transcript Factoring Special Case Polynomials
Daniella Feinberg, who recently retired from the fur business, and her husband are trying to enjoy a relaxing afternoon in front of their pool. She wants her husband, who makes a handsome living designing pools, to design one so she can throw a pool party...
What's this? The neighbor's dog just came through the fence and it looks like he has plans to do a little bit of surfing...Uh-oh... it looks like this pug's doggie instincts are taking over and he's ripping up the inflatable pool! I guess he's all about that pug life. Daniella's up in arms about the neighbor's dog. I guess her husband has his work cut out for him
Side lengths of the pool
Mr. Feinberg suggests that they build a square pool with side lengths 'a'. Using what we learned about area, we know we can find the area by multiplying the two side lengths together. Doing so gives us a², but Daniella doesn't like this option because it's too "normal". To make the pool less "normal", Mr. Feinberg suggests side measurements of (a - b), giving them a pool area of (a-b)(a - b) or (a - b)².
The FOIL method
Being the consummate professional, Mr. Feinberg uses the FOIL method to figure out exactly what he's dealing with. First he subtracts the b term from his existing plans. Since he already knows a(a) is a², he can concentrate on the other terms. a(-b) is -ab and since we have this twice, we can write it here...and here.
Finally, he has two '-b's that he needs to multiply together, giving him +b². Now he just adds all the terms together and combines like terms, leaving him with a² - 2ab + b². Daniella thinks it'd be silly if she threw a pool party with this small of a pool, so then Mr. Feinberg suggests (a + b)(a + b) to give Daniella the biggest pool that'll fit in their yard.
Write this mathematically
We can write this mathematically as (a + b)(a + b) or (a + b)². Mr. Feinberg's training is coming in handy, so when he applies the FOIL method to (a + b)², he knows he'll get a positive a² and b² and 'ab' twice gives him 2a making his final expression a² + 2ab + b². Since Daniella doesn't want to give up her rose garden, and since square pools are SO five minutes ago, she rejects this option as well.
Then, in a stroke of genius, Mr. Feinberg comes up with (a + b)(a - b). Mr. Feinberg uses the FOIL method one last time and gets a² one '+ab' one '-ab' and a -b². The two 'ab' terms cancel out, leaving Mr. Feinberg with a² - b². He draws it out for Daniella who looks at him quite curiously. She shows him that he's only cut out a little block from the area, not to mention the pool looks funny!
The area
Eureka again! Mr. Feinberg can move this piece here to make the pool not look as funny! All this talk of As and Bs is making Daniella a bit dizzy. She wants to know the area of the pool so that she can start planning the party. The Special Case Polynomials that Mr. Feinberg suggested were: a², (a - b)², (a + b)², and (a + b)(a - b).
Use PEMDAS for calculation
We can use any numbers for 'a' and 'b', but we'll use 10 feet for 'a' and 5 feet for 'b'. When we plug numbers in, instead of applying FOIL to our expression, we can use PEMDAS to make our calculations easier. Plugging 10 feet in for 'a' and 5 feet for 'b' gives us 100 ft.² for a² 25 ft.² for (a - b)² 225 ft.² (a + b)² and 75 ft.² for (a + b)(a - b). Good things usually come in threes, and Mr. Feinberg has had another brilliant idea...
All in a hard day's work! They're finally done! Now Daniella can throw her blowout pool party! What's this?!? Looks like a pugly situation...
Factoring Special Case Polynomials exercise
-
Determine the area of the pool.
HintsIf you've got any square with the side $s$, then the area is given by $s\times s=s^2$.
Use FOIL for multiplying two binomials. For example, let's factor $(a+2)\times (a+2)$:
First - multiply the first $a\times a=a^2$
Outer - multiply the outer $a\times 2=2a$
Inner - multiply the inner $2\times a=2a$
Last - multiply the last $2\times 2=4$
Adding all of the results together gives us $(a+2)^2=a^2+4a+4$.
You can use $(a+b)(a-b)$ to compute numbers; for example:
$102\times 98=(100+2)(100-2)=100^2-4=9996$.
SolutionWe know the side lengths for each pool Mrs. Feinberg can choose from. We need to figure out the area of each pool so that she can make the most educated decision about which pool she wants for her epic pool party.
$~$
Any square with the side lengths $a$ has the area $a\times a=a^2$. With this fact and the FOIL method for multiplication, we can figure these areas out!
Lets recall the FOIL method for multiplication:
First - multiply the first
Outer - multiply the outer
Inner - multiply the inner
Last - multiply the last
$~$
For the the leftmost pool, the sides are $a\times a=a^2$.
$~$
For the second pool, we need to multiply $(a-b)\times(a-b)$ to find the area.
Using the FOIL method, we have:
(1) "multiply the first" to get $a^2$.
(2) "multiply the outer" to get $-ab$.
(3) "multiply the inner" to get $-ab$.
(4) "multiply the last" to get $b^2$.
Adding all of these together gives us our area: $(a-b)\times(a-b)=a^2-2ab+b^2$.
$~$
Using the FOIL method in the same way for the third pool we get that the area is $(a+b)\times(a+b)=a^2+2ab+b^2$.
$~$
For the fourth pool, we get $(a+b)\times(a-b)=a^2-b^2$.
-
Calculate the area of each pool using the given values for $a$ and $b$.
HintsFirst calculate the the quantity inside the parenthesis and then multiply.
Here is an example.
Remember: PEMDAS; Parenthesis first and then Exponents.
SolutionFrom the leftmost pool to the rightmost:
First Pool:
- This pool is a square with side lengths $a=10~ft$.
- So we have to square $a$ to $(10~ft)^2=100~ft^2$.
- This pool is a square with side lengths $a-b$.
- So first we calculate the difference, $10~ft-5~ft=5~ft$.
- Then we square the difference $5~ft$ to get $(5~ft)^2=25~ft^2$.
- This pool is a square with side lengths $a+b$.
- So we calculate the sum $10~ft+5~ft=15~ft$.
- Then we square it to get $(15~ft)^2=225~ft^2$.
- This last pool is a rectangle with side lengths $10~ft+5~ft=15~ft$ and $10~ft-5~ft=5~ft$.
- To get the corresponding area we multiply these values to get $15~ft\times 5~ft=75~ft$.
-
Calculate the different possible sizes of the rose bed.
HintsCalculate the quantity inside the parenthesis first.
You'll use the following expressions:
- $(a+b)^2$
- $(a-b)^2$
- $(a+b)(a-b)$
Solution(1) The square rose bed with side lengths $a$ has area $a\times a=a^2=(15)^2=225~ft^2$.
(2) The square rose bed with side lengths $a+7$ has area $(a+7)^2=(15+7)^2=22^2=484~ft^2$.
(3) The square rose bed with side lengths $a-7$ has area $(a-7)^2=(15-7)^2=8^2=64~ft^2$.
(4) A rectangular rose bed with one side length of $a+3$ and another side length of $a-3$ has area $(a+3)(a-3)=(15+3)(15-3)=18\times12=216~ft^2$.
-
Find the carpet sizes using the FOIL method.
HintsFirst write down the different binomial products for the area of each carpet.
After writing down all the binomial products, calculate the quantities inside the parenthesis for each one.
SolutionLet's start with the smallest carpet with side lengths $12~ft+3~ft$ and $12~ft-3~ft$.
The size of this carpet is $15\times 9=135~ft^2$.
$~$
Next we have the square carpet with side lengths $16~ft-3~ft$.
The size of this carpet is $13^2=169~ft^2$.
$~$
The rectangular carpet with side lengths $15~ft+1~ft$ and $15~ft-1~ft$ is
$16\times 14=224~ft^2$.
$~$
The square carpet with the side lengths $12~ft$ is
$15^2=225~ft^2$.
$~$
The biggest carpet is the rectangular one with side lengths $16~ft+3~ft$ and $16~ft-3~ft$.
So it is $19\times 13=247~ft^2$.
-
Explain the FOIL method for multiplication.
HintsThe F step using the FOIL method for $(a+b)(a+b)$ is $a\times a=a^2$.
The I step using the FOIL method for $(a+b)(a+b)$ is $b\times a=ab$.
SolutionTo explain FOIL method for multiplication, let's have a look at the following example:
To multiply the binomials $(a+2)(b+3)$,
- First - multiply the first $a\times b=ab$
- Outer - multiply the outer $a\times 3=3a$
- Inner - multiply the inner $2\times b=2b$
- Last - multiply the last $2\times 3=6$
-
Use the product of binomials to calculate $43\times 37$.
HintsUse one of the equations:
- $(a+b)^2=a^2+2ab+b^2$
- $(a-b)^2=a^2-2ab+b^2$
- $(a+b)(a-b)=a^2-b^2$
Decide what the values of $a$ and $b$ need to be.
We have to solve to equations $a+b=43$ and $a-b=37$.
SolutionWe can use the formula $(a+b)(a-b)=a^2-b^2$ to help us solve $43\times 37$.
$~$
With this formula, we know that we have to solve the equations $a+b=43$ and $a-b=37$.
Adding these equations together, we get $2a=80$.
Then dividing by $2$ gives us $a=40$, and we can see that $b=3$.
Let's check: $(a+b)(a-b)=(40+3)(40-3)=43\times 37$ $\surd$
$~$
Looking at the right-hand side of the equation, we have $a^2-b^2$.
Substituting $a=40$ and $b=3$, we get $240^2-3^2=1600-9=1591$.
$~$
With this trick, multiplying numbers can seem like magic! Keep this in mind for the next time you want to impress your friends with your mental math skills.

Introduction to Polynomials – Naming Polynomials by Number of Terms

Adding Polynomials
Multiplying Polynomials
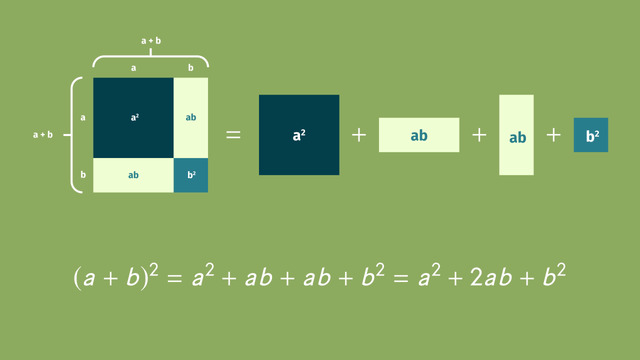
Multiplying Special Case Polynomials

Factoring out the GCF
Factoring Trinomials with a = 1

Factoring Trinomials with a ≠ 1

Factoring Special Case Polynomials
Factoring by Grouping

Subtracting Polynomials











