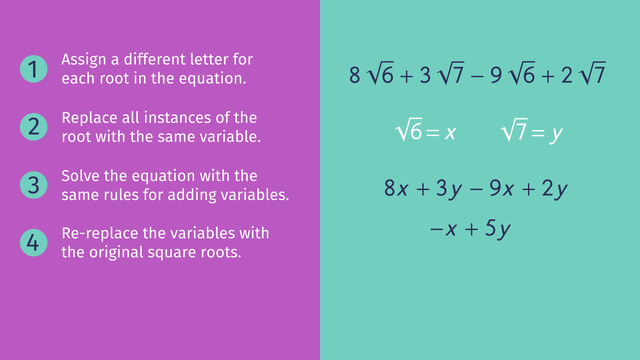Dividing Radical Expressions

Basics on the topic Dividing Radical Expressions
In order to divide radical expressions, we must simplify them. So knowing tools for simplifying radical expressions is key to dividing them.
The most important tool for dividing radical expressions is the quotient property of square roots.
This property states that √a / √b =√(a/b).
The quotient property of square roots can be used when simplifying or performing operations like division in radical expressions.
Specifically, √a / √b = √(a/b) can be used when a and b are not perfect squares and a/b is a rational number.
Also, √(a/b) = √a / √b can be used when a/b is not a perfect square, but separately a and b are rational numbers.
It is important to remember that the final result of dividing radical expressions must be in simplest radical form as well.
This video will show examples that explain how to use the quotient property of square roots for division of radical expressions.
Expressions and Equations Work with radicals and integer exponents.
CCSS.MATH.CONTENT.8.EE.A.2
Transcript Dividing Radical Expressions
Meet the man they call Wendelin
the path he takes is bafflin'.
The loyal, royal courier is he,
who expresses himself quite radically.
With no time to wander,
his travels take him yonder,
trundlin' packages for his Lord and Lady.
For a full day,
he travels the way
from Arden to Barton via Circuity.
Taking the long way 'round,
there's sure to be found,
a way shorter than his route currently.
What do I spy?
Is there a new bridge nearby?
Is it really a shortcut, Wendelin wonders.
To figure this out,
and leave no doubt,
we answer questions that divide radical expressions.
Rather than traveling two-thirds of a day to Circuity, to trip trap 'cross the bridge, and then travel an additional one-third of a day from there to Barton, Wendelin can simply cross the new bridge and travel directly from Arden to Barton! How's that, you ask?
The Pythagorean Theorem
Remember the Pythagorean Theorem? ‘a’ squared plus ‘b’ squared is equal to ‘c’ squared. Side 'c' is called the hypotenuse and is the longest side! Using the Pythagorean Theorem to solve for the missing measurement, Wendelin knows how to set up the equation to solve for a positive rational expression, but he needs help simplifying the radical expression.
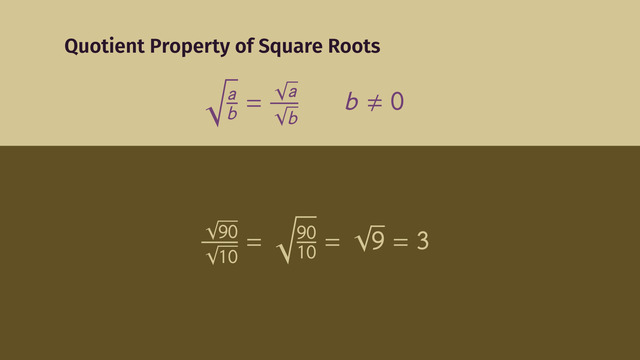
The Quotient Property of Square Roots
To solve this type of problem, use the Quotient Property of Square Roots: The square root of a quotient is equal to the quotient of the square roots of the numerator and denominator. For all positive real numbers 'a' and 'b' - 'b' does not equal zero. Take a look at how we can apply the quotient property of square roots to solve this problem: The square root of 90 divided by the square root of 10. This is the same as the square root of the fraction 90 over 10. We can simplify this to the square root of 9, and then solve. The quotient is 3, since squaring this number gives you 9.
Let’s try another problem: the square root of the fraction 49 over 9. Hmm, simplifying the fraction won't help, but we can split up the parts and take the square roots. The square root of 49 is equal to 7, and the square root of 9 is equal to 3 giving us 7 over 3. For more complex fractions such as this, note how we separate the coefficients from the radicals, and then apply the quotient property to simplify the square root. The fraction under this radical is simplified to the square root of 4, and the rest is easy.
Getting back to the original problem. Wendelin uses what he’s learned to simplify the radical expression. Using the Quotient Property of Square Roots, the solution is the square root of 5 over 3. Compared to the old route, the new route will save him one-quarter of a day!
Wendelin makes his merry way,
along his newfound pathway
to try out the new overpass.
He notices a troll,
who's blocking his goal,
with a lot of zeal and a lot of sass.
Radical as can be,
Wendelin solves the problems effortlessly,
but tomorrow's another story.
Dividing Radical Expressions exercise
-
Calculate the time it takes to travel the dotted path.
HintsThe Pythagorean Theorem states that
$a^2+b^2=c^2$,
where $c$ is the length of the hypotenuse and $a$ and $b$ are the lengths of the legs.
The quotient property of square roots:
To use the Pythagorean theorem first consider the hypotenuse and the legs.
SolutionThe given situation shows a right triangle with the two legs of lengths $\frac23$ and $\frac13$. The length of the hypotenuse $c$ is what we want to find.
We can use the Pythagorean Theorem to find $c$:
$a^2+b^2=c^2$
with
- $a=\frac13$
- $b=\frac23$
$c^2=\left(\frac13\right)^2+\left(\frac23\right)^2$.
Taking the square root, we get
$c=\sqrt{\left(\frac13\right)^2+\left(\frac23\right)^2}$.
We then have that
$\begin{array}{rcl} c&=&~\sqrt{\left(\frac13\right)^2+\left(\frac23\right)^2}\\ &=&~\sqrt{\frac19+\frac49}\\ &=&~\sqrt{\frac59} \end{array}$
And now we use the quotient property of square roots,
$\sqrt{\frac ab}=\frac{\sqrt a}{\sqrt b}$,
to get
$c=\frac{\sqrt5}{\sqrt9}=\frac{\sqrt5}{3}\approx 0.75$.
Thus we find that the new path is $0.75$ as long as the older one. Or, the other way round, Wendelin saves $0.25$ of a day.
-
Explain how to simplify $(-6\sqrt{20})\div(2\sqrt 5)$.
HintsKeep in mind that $\frac{a\times b}{c\times d}=\frac{a}{c}\times\frac{b}{d}$.
The quotient property of square roots:
The square root of any square is the squared term itself: $\sqrt{a^2}=a$.
SolutionWe can simplify this complicated looking fraction in a few steps:
- First we separate the coefficients from the radicals.
- Next apply the quotient property of square roots to simplify the square roots.
- Lastly simplify all terms as much as possible.
- $\frac{-6\sqrt{20}}{2\sqrt5}=\frac{-6}2\times\frac{\sqrt{20}}{\sqrt5}$
- $\frac{-6\sqrt{20}}{2\sqrt5}=-3\times\sqrt{\frac{20}5}$
- $\frac{-6\sqrt{20}}{2\sqrt5}=-3\times\sqrt{4}=-3\times 2=-6$
-
Find the length of the hypotenuse $c$.
Hints$c$ is the length of the hypotenuse. The lengths of the legs are given.
Here you see the Pythagorean theorem:
$a^2+b^2=c^2$.
Take the square root last.
The square root of any square is the squared term itself: $\sqrt{a^2}=a$.
SolutionThe tool we need is the Pythagorean theorem, which states that
$a^2+b^2=c^2$,
where $a$ and $b$ are the lengths of the legs of a right triangle and $c$ is the hypotenuse.
In all the examples above the hypotenuse is wanted.
We are asked the hypotenuse of several right triangles, where the lengths of the legs are known:
- $a=4$ and $b=3$: So we get $c=\sqrt{4^2+3^2}=\sqrt{16+9}=\sqrt{25}=5$
- $a=7$ und $b=4$ give us $c=\sqrt{7^2+4^2}=\sqrt{49+16}=\sqrt{65}$
- $a=5$ and $b=\sqrt{11}$ lead to $c=\sqrt{5^2+\sqrt{11}^2}=\sqrt{25+11}=\sqrt{36}=6$
- $a=\frac14$ and $b=\frac13$ result in $c=\sqrt{\left(\frac35\right)^2+\left(\frac45\right)^2}=\sqrt{\frac{9}{25}+\frac{16}{25}}=\sqrt{\frac{25}{25}}=1$
-
Solve the radical equations.
HintsUse the quotient property of square roots each time.
For the two equations on the right you first have to seperate the coefficients from the radicals.
The square root of any square is the squared term itself: $\sqrt{a^2}=a$.
SolutionFor the following equations we use the quotient property of square roots: $\sqrt{\frac ab}=\frac{\sqrt a}{\sqrt b}$.
$~$
Let's start with the equation in the upper left-hand corner:
$\begin{array}{rclll} \frac{\sqrt{24}}{\sqrt6}&=&~\sqrt{\frac{24}{6}}&|&\text{quotient property}\\ &=&~\sqrt4\\ &=&~2&|&\text{as }2^2=4 \end{array}$
Now for the equation in the bottom left-hand corner:
$\begin{array}{rclll} \sqrt{\frac{49}{100}}&=&~\frac{\sqrt{49}}{\sqrt{100}}&|&\text{quotient property}\\ &=&~\frac7{10}&|&\text{as }7^2=49\text{ and }10^2=100 \end{array}$
$~$
To solve the equations on the right side we first have to separate the coefficients.
Let's start with the equation in the upper right-hand corner:
$\begin{array}{rclll} \frac{3\sqrt{24}}{12\sqrt6}&=&~\frac3{12}\times\frac{\sqrt{24}}{\sqrt{6}}&|&\text{seperating the coefficients}\\ &=&~\frac14\times\sqrt{\frac{24}{6}}&|&\text{quotient property}\\ &=&~\frac14\times\sqrt4\\ &=&~\frac14\times2=\frac12&|&\text{as }2^2=4 \end{array}$
The last equation is the one in the bottom right-hand corner:
$\begin{array}{rclll} \frac{-2\sqrt{48}}{5\sqrt3}&=&~\frac{-2}{5}\times\frac{\sqrt{48}}{\sqrt{3}}&|&\text{seperating the coefficients}\\ &=&~-\frac25\times\sqrt{\frac{48}{3}}&|&\text{quotient property}\\ &=&~-\frac25\times\sqrt{16}\\ &=&~-\frac25\times4=-\frac85&|&\text{as }4^2=16 \end{array}$
-
Simplify the given radical expressions.
HintsThe square root of any square is the squared term itself:
- $\sqrt4=\sqrt{2^2}=2$
- $\sqrt{16}=\sqrt{4^2}=4$
- $\sqrt{25}=\sqrt{5^2}=5$
You can simplify any fraction by dividing the numerator as well as the denominator by the same value:
$\frac{16}{8}=\frac{16\div8}{8\div8}=\frac21=2$
SolutionHere we study the quotient property of square roots,
$\sqrt{\frac ab}=\frac{\sqrt a}{\sqrt b}$,
or rather
$\frac{\sqrt a}{\sqrt b}=\sqrt{\frac ab}$.
Let's start with
$\begin{array}{rclll} \frac{\sqrt{90}}{\sqrt{10}}&=&~\sqrt{\frac{90}{10}}&|&\text{ quotient property}\\ &=&~\sqrt 9&|&\text{ simplifying the fraction}\\ &=&~3&|&\text{ because }3^2=9 \end{array}$
Here you see another example:
$\begin{array}{rclll} \sqrt{\frac{49}{9}}&=&~\frac{\sqrt{49}}{\sqrt 9}&|&\text{ quotient property}\\ &=&~\frac73&|&\text{ because }7^2=49 \text{ and }3^2=9 \end{array}$
-
Identify the simplified form of the radical expressions.
HintsTo seperate the coefficients, if necessary, use $\frac{a\times b}{c\times d}=\frac{a}{c}\times\frac{b}{d}$.
Here you see an example for the quotient property of square roots:
The square root of any square is the squared term itself: $\sqrt{a^2}=a$.
SolutionIf you have to divide radical expressions or take the square root of fractions you have to use the quotient property of square roots: $\sqrt{\frac ab}=\frac{\sqrt a}{\sqrt b}$.
$~$
Once again we practice this, together with separating the coefficients first, if necessary:
$\begin{array}{rclll} \frac{\sqrt{242}}{\sqrt2}&=&~\sqrt{\frac{242}{2}}&|&\text{quotient property}\\ &=&~\sqrt{121}\\ &=&~11&|&\text{because }11^2=121 \end{array}$
$~$
$\begin{array}{rclll} \sqrt{\frac{121}{25}}&=&~\frac{\sqrt{121}}{\sqrt{25}}&|&\text{quotient property}\\ &=&~\frac{11}{5}&|&\text{because }11^2=121\text{ and }5^2=25 \end{array}$
$~$
For the next examples we first have to separate the coefficients:
$\begin{array}{rclll} \frac{12\sqrt{80}}{3\sqrt5}&=&~\frac{12}3\times\frac{\sqrt{80}}{\sqrt{5}}&|&\text{seperating the coefficients}\\ &=&~4\times\sqrt{\frac{80}{5}}&|&\text{quotient property}\\ &=&~4\times\sqrt{16}\\ &=&~4\times4=16&|&\text{because }4^2=16 \end{array}$
$~$
$\begin{array}{rclll} \frac{5\sqrt{112}}{20\sqrt7}&=&~\frac{5}{20}\times\frac{\sqrt{112}}{\sqrt{7}}&|&\text{seperating the coefficients}\\ &=&~\frac14\times\sqrt{\frac{112}{7}}&|&\text{quotient property}\\ &=&~\frac14\times\sqrt{16}\\ &=&~\frac14\times4=1&|&\text{because }4^2=16 \end{array}$