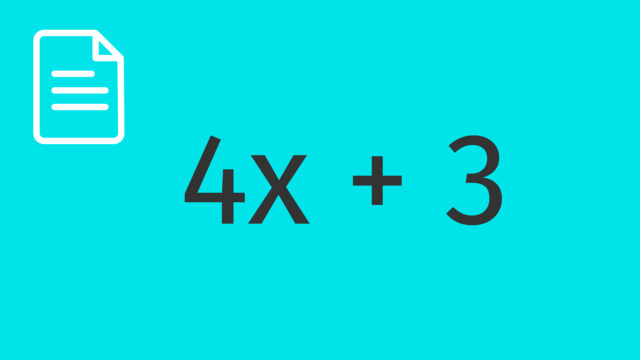Using Mathematical Terms
- Using Mathematical Terms
- Understanding Mathematical Terms
- Sum – Definition and Application
- Term– Definition and Application
- Product – Definition and Application
- Factor– Definition and Application
- Quotient– Definition and Application
- Coefficient – Definition and Application
Learning text on the topic Using Mathematical Terms
Using Mathematical Terms
Welcome to the fascinating world of mathematical terms! Anyone, who is approaching Middle School, needs to prepare to solve increasingly more and difficult mathematical problem-solving and reasoning questions. These questions often use high level mathematical terms. In this text, we'll explore crucial terms like sum, term, product, factor, quotient, and coefficient. These terms are the building blocks of mathematics, and understanding them is key to mastering many mathematical concepts.
Understanding Mathematical Terms
It is important to have a deep understanding of each of the mathematical terms to understand what mathematical operation needs to be completed. Therefore, you will find a detailed explanation of sum, term, product, factor, quotient, and coefficient below.
Sum – Definition and Application
The sum is the result of adding two or more numbers together.
In a written task such as ‘The sum of two numbers is 15. If one number is 8, what is the other number?’ it is important to understand the role the word ‘sum’ plays in this problem. When we see the word sum, it doesn’t always mean find the sum, but rather it is a clue as to what needs to be done to solve the problem. Sometimes you might need to use the inverse operation, which for sum would be subtraction.
Term– Definition and Application
In mathematics, a term is a single mathematical expression. It can be a number, a variable, or numbers and variables multiplied together.
In mathematics, especially in algebra, term refers to a single mathematical expression. It can be a number, a variable, or the product of numbers and variables. In a written task such as, "Identify the terms in the expression 3x + 4y - 5," it's important to recognize that terms are separated by plus or minus signs. Here, 3x, 4y, and -5 are distinct terms. Understanding this helps you to break down expressions into simpler parts for further operations or simplifications.
Product – Definition and Application
The product is the result of multiplying two or more numbers.
The term product refers to the result of multiplying two or more numbers or expressions. In a task like, "Find the product of 7 and 7," you must recognize that finding the product means performing multiplication. This term is crucial in understanding how to combine numbers and variables in algebraic expressions. Sometimes, the question might suggest the product is a given value, in which case you might need to use the inverse operation, division, to solve the problem.
Factor– Definition and Application
A factor is a number that divides into another number without leaving a remainder.
A factor is a number or expression that divides another number or expression evenly, with no remainder. In problems like, "List all the factors of 24," you need to identify numbers that can perfectly divide 24 (such as 1, 2, 3, 4, 6, 8, 12, 24). Understanding factors is key in simplifying fractions, finding the greatest common factors, and solving division-related problems. It’s about recognizing the building blocks that make up a number.
Factors are important, and they are applied in Prime Factorization, Least Common Multiples, and Finding the Greatest Common Factor.
Quotient– Definition and Application
The quotient is the result of dividing one number by another.
The quotient is the result of division. In a task like, "Divide 15 by 3 to find the quotient," you must recognize that the quotient is the answer to a division problem. This concept is essential in understanding how division works and in solving problems that require dividing numbers. It's important to note that the quotient helps in breaking down larger numbers into smaller, more manageable parts.
Coefficient – Definition and Application
A coefficient is a number used to multiply a variable.
In algebra, a coefficient is a number used to multiply a variable. For example, in the expression 4x + 3, 4 is the coefficient of x. When you encounter problems like, "Identify the coefficient in the term 7y," you must be able to identify that the coefficient is the numerical part of the term that is attached to the variable. Understanding coefficients is crucial in algebra, as it helps in simplifying expressions and solving equations. It’s about recognizing how numbers and variables are linked in algebraic expressions.
Mathematical Terms – Application
Test your understanding of mathematical terms with these questions.
Mathematical Terms Summary
Key Learnings from this Text:
Understanding mathematical terms like sum, term, product, factor, quotient, and the coefficient is essential.
These terms are used in various mathematical operations and expressions.
Recognizing and applying these terms correctly enhances your mathematical comprehension and problem-solving skills.
Here is a table of the terms and definitions for you to reference or make a copy of for your use.
| Term | Definition |
|---|---|
| Sum | The result of adding two or more numbers together. |
| Term | A single mathematical expression, which can be a number, variable, or both combined. |
| Product | The result of multiplying two or more numbers. |
| Factor | A number that divides another number exactly, without leaving a remainder. |
| Quotient | The result of dividing one number by another. |
| Coefficient | A number that multiplies a variable in an algebraic expression. |
Keep practicing these terms in different mathematical contexts to strengthen your understanding. Remember, these terms are not just numbers or expressions; they help structure the world of mathematics! Now you should be ready to explore Using Variables to Represent Numbers in Expressions and Equivalent Expressions
Mathematical Terms – Frequently Asked Questions
Using Mathematical Terms exercise
-
Match the terms and definitions.
Hints- Sum is used for addition
- Product is used for multiplication
- Quotient is used for division
- A factor is a whole number that can be multiplied by another whole number to produce a given product
- A coefficient is the number in front of a variable in a mathematical expression
- A term is an expression that has numbers and/or variables
SolutionHere is the completed table.
-
What is the correct mathematical term?
HintsThe product is the result of multiplying two or more numbers.
A coefficient is a number used to multiply a variable.
The sum of 4 and 6 is 10.
SolutionSum refers to the result of adding two or more numbers together. It's the total obtained when you combine quantities. For example, in the addition problem 3 + 5, the sum is 8.
-
Identify the coefficient in the term 7y.
HintsA coefficient is a number used to multiply a variable. So the coefficient here is 5.
What is y multiplied by?
SolutionA coefficient is a number used to multiply a variable, so the coefficient is 7.
-
Identify the product in the equation.
HintsThe product is the result of multiplying two factors.
The product of 2 x 3 is 6.
SolutionThe product is the result of multiplying two factors.
-
The sum of two numbers is 20. If one number is 9, what is the other number?
HintsSum means to add.
What number do we add to 9 to get 20? Or, what is 20 - 9?
How many numbers do we count up from 9 to 20?
SolutionThe correct answer is 11.
If we count up from 9 to 20, we count 11. 9 + 11 = 20, so the answer is 11.
-
Look at the bolded items. Match each term to the example.
HintsSum- the result of adding two or more numbers
The quotient is found by dividing two numbers.
You multiply two factors to find the product, or answer, of a multiplication equation.
SolutionThe sum is the result of adding 2 or more numbers.
- 8 + 6 = 14
- 3 + a
- 15 x 26 = 390
- 15 x 26 = 390
- 28 $\div$ 2 = 14
- 5x + 8 = 2