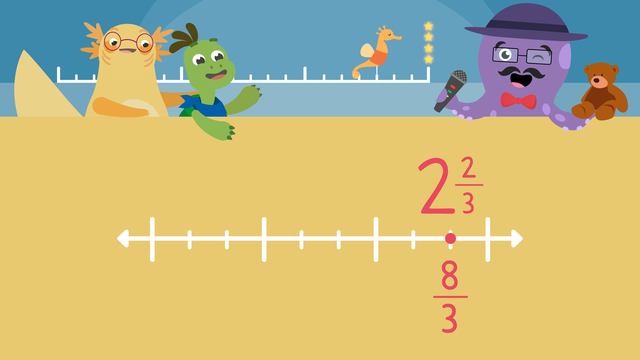
Improper Fractions and Mixed Numbers—Let's Practice!

Improper Fractions and Mixed Numbers—Let's Practice! exercise

• Convert the improper fraction to a mixed number.

  Hints

  This shows a completely shaded whole.

  In this incomplete bar, there are 3 parts shaded out of 4 in total.

  Solution

  • In the image there are 2 completely shaded wholes.
  • There are 2 parts shaded out of 7 in the incomplete bar.
  • $\frac{16}{7}$ as a mixed number is 2 $\mathbf{\frac{2}{7}}$

• Using mixed numbers.

  Hints

  First count how many wholes are shaded in. This is the whole number part of the mixed number.

  In this image there are two wholes.

  Second, how many parts of an incomplete whole are there? This is the numerator (top) part of the mixed number.

  In this image, the whole is incomplete but there are three parts shaded.

  Finally, what is each fraction bar divided into? This is the denominator, (bottom part) of the mixed number.

  In this image there are four parts altogether.

  Solution

  This image shows the correctly written mixed numbers for each fraction bar image.

• Converting an improper fraction.

  Hints

  First, count how many wholes are shaded in. This is the whole number part of the mixed number.

  Second, how many parts of an incomplete whole are there? This is the numerator (top) part of the mixed number.

  Finally, what is each fraction bar divided into? This is the denominator (bottom) part of the mixed number.

  Solution

  $\frac{11}{4}$ as a mixed number is 2 $\mathbf{\frac{3}{4}}$

• Convert to a mixed number.

  Hints

  First, count how many wholes are shaded in. This is the whole number part of the mixed number.

  Second, how many parts of an incomplete whole are there? This is the numerator (top) part of the mixed number.

  Finally, what is each fraction bar divided into? This is the denominator (bottom) part of the mixed number.

  Solution

  $\frac{18}{5}$ as a mixed number is 3 $\mathbf{\frac{3}{5}}$

• What is the fraction as a mixed number?

  Hints

  First, count how many wholes are shaded in. This is the whole number part of the mixed number.

  Second, how many parts of an incomplete whole are there? This is the numerator (top) part of the mixed number.

  Finally, what is each fraction bar divided into? This is the denominator (bottom) part of the mixed number.

  In this mixed number: The whole is 2, the numerator is 3 and the denominator is 4.

  Solution

  $\frac7 3$ = 2 $\frac1 3$

  • The fraction bars are divided into three parts, so this is the denominator.
  • There are two whole fraction bars shaded, so this is the whole number part of the mixed number.
  • There is one part shaded in the incomplete fraction bar, so this is the numerator.

• Converting mixed numbers.

  Hints

  Look at the denominator in the mixed number, this will be the denominator in the improper fraction.

  For example, in 2 $\frac2 5$ the denominator is 5.

  Multiply the denominator by the whole in the mixed number, in 2 $\frac2 5$ we would do 5 x 2 = 10.

  Then add on the numerator, which is 2. 10 + 2 = 12. This will be the numerator in the improper fraction. $\frac{12}{5}$

  Solution

  This image shows how the table should be completed.

  • 1 $\frac{2}{3}$ = $\frac{5}{3}$
  • 3 $\frac{4}{5}$ = $\frac{19}{5}$
  • 3 $\frac{2}{7}$ = $\frac{23}{7}$
  • 3 $\frac{1}{3}$ = $\frac{10}{3}$

  ----------------------------------------------------------

  For the first question, there is 1 $\frac{2}{3}$. To convert this, look at how many wholes there are, and multiply this by the denominator. Here there is one whole, so we multiply 1 x 3 = 3 or $\frac{3}{3}$. We then add on the numerator, 2. $\frac{3}{3}$ + $\frac{2}{3}$ = $\frac{5}{3}$