Fractions Greater than 1 on a Number Line

Basics on the topic Fractions Greater than 1 on a Number Line
Fractions Greater than 1 on a Number Line
You are probably familiar with the cardinal numbers already. But what about numbers or fractions greater than 1? How do you determine fractions greater than 1?.This text for third grade will teach about two ways of labeling fractions greater than 1 on a number line.
You can identify fractions on a number line greater than 1 as improper fractions or mixed numbers. Improper fractions have numerators that are greater than their denominators. Mixed numbers have a whole number and a fraction.

Fractions Greater than 1 on a Number Line – Improper Fractions
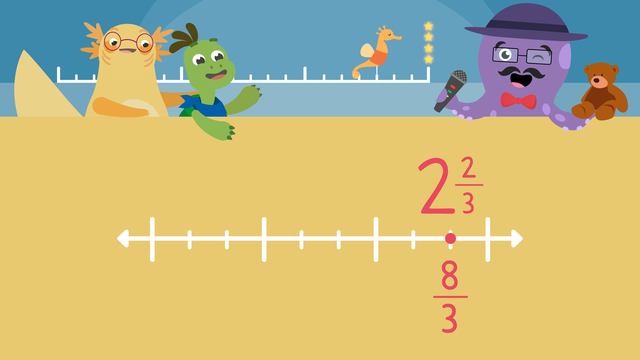
To identify the improper fraction, begin by locating the point on the number line. Next, count the number of spaces until you reach it. Write this number as the numerator, or top number.
Then, count how many parts one whole is divided into. Write three as the denominator, or bottom number.
So, eight-thirds is the location of this improper fraction on the number line.
Fractions Greater than 1 on a Number Line – Mixed Numbers
To identify the fraction greater than one as a mixed number, begin by locating the point on the number line. Next, count the amount of whole numbers before the point. Write the whole number on the left.
Then, count the number of spaces between the last whole number and the point. Write this number as the numerator, or top number.
Then, count how many parts one whole is divided into. Write that number as the denominator, or bottom number.
So this same point could also be read as the mixed number two and two thirds. Another way to state is that this fraction has two wholes plus two more thirds remaining.
Fractions Greater than 1 on a Number Line – Summary of Steps
This is how you identify fractions greater than 1 on a number line. The chart below shows the necessary steps.
| Step # | What to do |
|---|---|
| 1 | Locate the point on the number line. |
| 2 | Count the number of spaces to that point. Write this number as the numerator. |
| 3 | Count how many parts one whole is divided into. Write this number as the denominator. |
| 4 | The final number is the location of the improper fraction on the number line. |
| (5) | For mixed numbers, you can count the whole numbers first, then add on the remaining fractions. |
Fractions Greater than 1 on a Number Line – Worksheets and Activities
Have you practiced fractions on a number line greater than 1 questions for third grade yet? On this website, you can also find fractions on a number line greater than 1 worksheets, like worksheets plotting fractions greater than 1 on a number line, and fractions on a number line greater than 1 activities.
Transcript Fractions Greater than 1 on a Number Line
Axel and Tank are at the carnival. They can't wait to play Racing Seahorses! The player whose seahorse finishes first wins a prize! Ready, set, go! As they race, Axel and Tank want to identify where their seahorses are... but they dont know how! Let's help them by identifying "Fractions Greater than 1 on a Number Line." You can identify fractions greater than one on a number line as improper fractions or mixed numbers. Improper fractions have numerators that are greater than their denominators. Mixed numbers have a whole number and a fraction. To identify the improper fraction, begin by locating the point on the number line. Next, count the number of spaces until you reach it. Let's count the number of spaces one, two, three, four, five, six, seven, eight. The point is on eight. Write this number here as the numerator, or top number. Then, count how many parts one whole is divided into. Let's count together one, two, three. Write three here as the denominator, or bottom number. Eight-thirds is the location of this improper fraction on the number line. We could also read it as the mixed number two and two thirds because this fraction has two wholes plus two more thirds remaining. Now, let's help Tank locate the position of his seahorse in the race. First, we locate the seahorse as a point on the number line. Next, we count the number of spaces until we reach it: one, two, three, four, five, six, seven! Write seven as the numerator. Then, count how many parts one whole is divided into one, two, three, four, five. Write five as the denominator. Tank's seahorse is located at seven-fifths on the number line. We could also say its located at one and two fifths because this fraction has one whole plus two more fifths remaining. Let's help Axel find his seahorse! First, we locate the seahorse as a point on the number line. What do we do next? We count the number of parts to that point: one, two, three, four and write four as the numerator. What should we do next? Next, we count how many parts one whole is divided into one, two. Write two as the denominator. Where is Axel's seahorse located? Axel's seahorse is located at four-seconds on the number line. Do you notice anything else about this improper fraction? Four-seconds is also written as two on the number line. This means that four-seconds is the same as, or equivalent to, the number two. The third player's seahorse is here. What is the numerator? The numerator is thirteen because we counted thirteen spaces to get to their seahorse. What is the denominator? The denominator is seven because one whole is divided into seven spaces. Where is the third player's seahorse? Their seahorse is at thirteen-ninths on the number line. What is the equivalent mixed number? It's one and six sevenths because this fraction has one whole plus six more sevenths remaining. "Folks! We have a winner!" Before we find out who won let's remember. We can identify fractions greater than one on a number line as improper fractions or mixed numbers. Improper fractions have numerators that are larger than their denominators. Mixed numbers have whole numbers and fractions. To read the improper fraction, first locate the point. Second, count the number of spaces to that point. Write this number as the numerator. Then, count how many parts one whole is divided into. Write this number as the denominator. The final number is the location of the improper fraction on the number line. For mixed numbers, you can count the whole numbers first, then add on the remaining fractions. Congratulations, Tank you came from behind but, slow and steady always wins the race. So, you can have two prizes! .
Fractions Greater than 1 on a Number Line exercise
-
Identify fractions greater than one on a number line.
HintsThe number of parts the whole is divided into is the denominator. Here the denominator is 3.
The numerator is the number of spaces that have been counted to reach the point.
SolutionFirst locate the point, then count the number of spaces to that point.
Write this number as the numerator or the top part of the fraction.
Then count how many parts one whole is divided into, and write this as the denominator.
The final number is the location of the improper fraction on the number line.
-
Racing seahorses!
HintsTo find the numerator, find how many spaces the sea horse has jumped.
To find the denominator, look at what each whole has been divided into.
SolutionAxel's seahorse has reached $\frac{12}{5}$.
- First, find how many spaces the seahorse has jumped, and write this as the numerator, 12.
- Second, look at what the whole is divided into and write this as the denominator, 5.
-
Matching improper fractions and mixed numbers.
HintsThe numerator is the number of spaces that have been counted to reach the point.
The number of parts the whole is divided into is the denominator. Here the denominator is 3.
A mixed number means that there is a whole number plus a fraction. Here the sea horse has moved 1 whole plus another 3 parts out of 7, so it has moved 1 $\frac3 7$.
Solution- The first seahorse is at 2$\mathbf{\frac{3}{4}}$.
- The next seahorse is at 1$\mathbf{\frac{4}{6}}$.
- The third seahorse is at $\mathbf{\frac{5}{3}}$.
- The last seahorse is at $\mathbf{\frac{7}{5}}$.
-
Identify the seahorses on the number line.
HintsWhen finding the improper fraction, count the spaces along the number line to the seahorse to find the numerator.
When finding an improper fraction, look at what the whole has been divided into to find the denominator.
For a mixed number, first find how many wholes, then how many more spaces to the seahorse.
SolutionThe seahorse at 1 $\frac2 5$ is green. It has traveled one whole part, plus two more fifths.
The seahorse at 2 $\frac1 5$ is blue. It has traveled two whole parts, plus one more fifth.
The seahorse at $\frac9 5$ is pink. It has traveled nine spaces along the number line and each whole is divided into five.
The seahorse at $\frac{10}{5}$ is yellow. It has traveled ten spaces along the number line and each whole is divided into five.
-
Mixed numbers.
HintsFirst, look at how many wholes the seahorse has travelled.
Then, look at how many more spaces the seahorse has traveled.
SolutionTank's seahorse has reached 1 $\frac3 7$. It has traveled one whole plus 3 more jumps, and each whole is made of 7 parts.
-
Convert between improper fractions and mixed numbers.
HintsRemember that whatever the denominator is, this tells you how many parts the whole is divided into and how many intervals there are between each whole on the number line.
Remember that the denominator tells us how many parts the whole is divided into, so if 5 is the denominator, $\frac5 5$ would be one whole and $\frac6 5$ would be 1 $\frac1 5$.
In a mixed number such as 3 $\frac2 3$, there are $\frac3 3$ in a whole so here there would be $\frac9 3$ plus $\frac2 3$ = $\frac{11}{3}$.
Solution1.
- $\frac{12}{10}$ = 1 $\frac{2}{10}$
- $\frac{15}{4}$ = 3 $\frac3 4$
- $\frac9 5$ = 1 $\frac4 5$
- $\frac{17}{6}$ = 2 $\frac5 6$
- 3 $\frac3 9$ = $\frac{30}{9}$
- 2 $\frac4 7$ = $\frac{18}{7}$




















awesome!