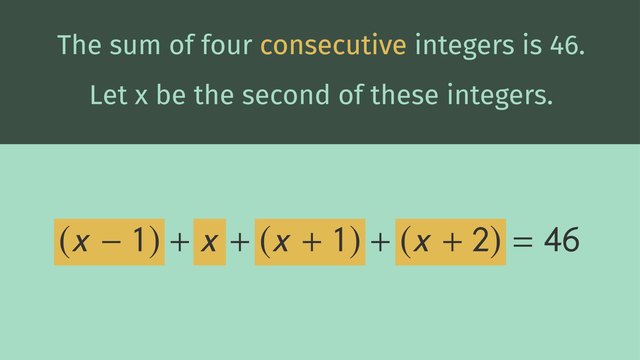Writing and Solving Linear Equations

Basics on the topic Writing and Solving Linear Equations
Summary of Writing and Solving Linear Equations
A linear equation in one variable can be solved by applying the distributive property and other properties of equality.
Solving linear equations in one variable is a necessary skill when calculating dimensions, solving pricing problems, and solving mixture problems in real life.
Learn how to solve linear equations in one variable by helping Irving, the investigator, figure out the right meeting spot in the city by using the hints and the location marks on the city map provided to calculate the angles of each triangle.
Common Core Reference
CCSS.MATH.CONTENT.8.EE.C.7.B
Let's practice: solving linear equations Exercises
With the following exercises on the topic of solving linear equations, you can test whether you have understood everything. Test your knowledge now!
Transcript Writing and Solving Linear Equations
While trying to crack a case, Irving the investigator gets an anonymous message...by fax? What’s this? It looks like a city map with a bunch of locations marked. The message says that Irving can figure out the right meeting spot by calculating each triangle's angles from the provided hints. The fax tells him that figuring out the angles of each triangle will show him the RIGHT meeting point. Let's do this by helping Irving the investigator solve linear equations in one variable. First, let's translate the hints into algebraic language. In each hint, there are three unknown angles, which means it's a triangle. The first hint says that the angles that make up the first triangle are all the same. That's an equilateral triangle. Since they're unknown values and all the same we can just use the variable 'x' to represent each angle. The sum of the interior angles of a triangle add up to be 180 degrees. Therefore, when we sum up three of the same unknown values we can write it as a linear equation, 3x equals 180. Using the opposite operation of division, we can isolate our variable and see that 'x' equals 60. So each of the angles in the triangle is 60 degrees. Since all the angles in this triangle are the same measure, and Irving can't be in three places at once, this must not tell him the right meeting place. Irving can disregard this triangle. Let's take a look at the hint for the next triangle. For this triangle, the first measure is unknown. The second angle is three times the first plus 3 and 1/3. And the third angle is twice as large as the second angle. Though this problem contains mixed numbers, we can still solve it just like before by writing a linear expression. All we need to do is deal with it piece by piece. First, sum the variable expressions that represent the measurements of the three unknown angles. Then, set the expression equal to 180, the total interior angle measure of a triangle. Now we have a linear equation. To solve for 'x', first, use the distributive property by multiplying 2 by 3x and 2 by 3 and one third. Combine the like terms and then use opposite operations to isolate 'x'. Use the opposite operation of subtraction to undo the addition by 10, and then the opposite operation of division by ten on both sides of the equation to finish isolating the 'x', giving us 17. Next, we can substitute this back into our equations for each angle. Our first angle is, of course, 17 degrees. Plugging 17 into our equation for angle 2, we get 3 times 17 plus 3 and one third, 3 times 17 is 51. Then, adding our remaining numbers gives us 54 and one third degrees. Although the expression for angle 3 looks messy, remember, we already solved the part inside the parentheses and got 54 and one third! Just multiply that result by 2 and we have our answer! 108 and two-thirds degrees. This can't be it. This whole triangle's in the water. So unless Irving needs to buy scuba gear, this isn't it, either. Hmmm... we have one more triangle left. The second angle is twice as large as the first angle, so we can represent this by calling angle 1 'x' and expressing angle 2 in terms of 'x', giving us 2x. The third angle is three times the size of the first angle, so 3x. Like the first two triangles, this information can be written as a linear equation by summing the three, unknown angles. To solve for 'x', we first combine the like terms, 'x' plus 2x and 3x, giving us 6x. Next, we should divide both sides by 6 to isolate the 'x'. Therefore, 'x' equals 30. Now, let's evaluate each of the angles, using 30 for 'x'. This means the measure of angle 1 is 30 degrees, the measure of angle 2 is 60 degrees, and the measure of angle 3 is equal to 90 degrees. 90 degrees!? That's a right triangle. The hints have shown Irving that the triangle must be a 30-60-90 triangle. That must be it! The RIGHT meeting spot! Irving gets in his car and drives to the vertex of the right angle. What’s this? It was all just a scheme to have a surprise party!? Happy Birthday Irving!
Writing and Solving Linear Equations exercise
-
Solve the linear equation using the sum of interior angles of a triangle theorem.
HintsThe interior angles of any triangle sum to $180^\circ$.
Check your solution by adding all angles together; the sum must be $180^\circ$.
- "Two times" indicates a factor of $2$.
- "Three times" indicates a factor of $3$.
SolutionEach angle is unknown but they have a relationship to one another.
The second angle is two times the first one and the third angle is three times the first one. Assigning the variable $x$ to the first angle, we can then express these relationships in the following way:
- $m\angle 1=x$
- The second angle is two times the first, or $m\angle 2=2x$
- The third angle is three times the first, or $m\angle 3=3x$
- Combining like terms gives us $6x=180^\circ$.
- Lastly, we divide by $6$ to get $x=30^\circ$.
- $m\angle 1=30^\circ$
- $m\angle 2=2\times 30^\circ=60^\circ$
- $m\angle 3=3\times 30^\circ=90^\circ$
-
Solve the equation using knowledge of linear equations and geometric properties.
HintsThe sum of all interior angles of any triangle is
$m\angle 1 + m\angle 2 + m\angle 3=180^\circ$.
The perimeter of a rectangle is given by $2l+2w$.
Here you see the distributive property: you have to multiply the factor $a$ with each summand inside the parenthesis and add the resulting products.
SolutionWe start with the equilateral triangle. All interior angles are equal $m\angle 1=m\angle 2=m\angle 3=x$ and thus $x+x+x=180^\circ$ or, combining like terms, $3x=180^\circ$. Last we divide by $3$ to get
$m\angle 1=m\angle 2=m\angle 3=60^\circ$
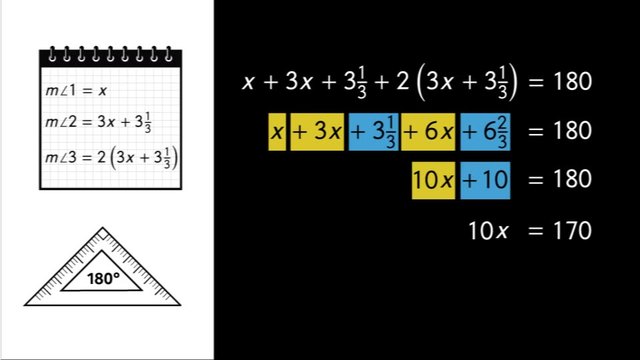
The angles of the next triangle have the following relationship:
- $m\angle 1=x$
- $m\angle 2=3x+3\frac13$
- $m\angle 3=2\left(3x+3\frac13\right)$
- We use the distributive property: $x+3x+3\frac13+6x+6\frac23=180^\circ$.
- Rearranging the terms leads to $x+3x+6x+3\frac13+6\frac23=180^\circ$.
- Now we combine the like terms to get $10x+10=180^\circ$.
- Next subtracting $10$ from both sides we get $10x=170^\circ$.
- Dividing by $10$ results in $x=17^\circ$.
- $m\angle 1=17^\circ$
- $m\angle 2=3\times 17^\circ+3\frac13^\circ=54\frac13^\circ$
- $m\angle 3=2\left(54\frac13^\circ\right)=108\frac23^\circ$
The perimeter of a rectangle is given by $2l+2w$. Thus we can establish the following equation, which we solve using opposite operations:
$\begin{array}{rclll} 2x+ 2(x+12) & = & ~44&|&\text{distributive property}\\ 2x+2x+24&=&~44&|&\text{combining like terms}\\ 4x+24&=&~44\\ \color{#669900}{-24} & & \color{#669900}{-24}\\ 4x & = & ~20\\ \color{#669900}{\div 4} & & \color{#669900}{\div 4}\\ x&=&~5 \end{array}$
The desired width is $5~\text{cm}$ and the length is $l=5~\text{cm}+12~\text{cm}=17~\text{cm}$.
-
Sort the steps needed to solve one variable linear equations in geometry.
HintsHere you see an example for combining like terms:
$3x+x+2x=6x$.
All interior angles of a triangle sum to $180^\circ$.
SolutionAssigning $x$ to the first angle, we can represent each angle by
- $m\angle 1=x=40^\circ$,
- $m\angle 2=x+10^\circ$, and
- $m\angle 3=2x+10^\circ$.
$x+x+10^\circ+2x+10^\circ=180^\circ$.
- Combining like terms leads to $4x+20^\circ=180^\circ$.
- Subtracting $20^\circ$ results in $4x=160^\circ$.
- Dividing both sides by $4$ gives $x=40^\circ$.
- $m\angle 2=40^\circ+10^\circ=50^\circ$, and
- $m\angle 3=2(40^\circ)+10^\circ=90^\circ$.
-
Solve the word problems using linear equations and geometric properties.
HintsIn a right triangle the (non right) remaining angles sum to $90^\circ$.
Pay attention that Paul's fence only has three sides: a longer one and two shorter ones.
The surface area of a cube with the side length $s$ is given by $6s^2$.
In an isosceles triangle the base angles are equal.
SolutionTyrese
In a right triangle the remaining angles sum to $90^\circ$. This together with the fact that one angle is $30^\circ$ more than three times the other angle leads to $x+30^\circ+3x=90^\circ$.
- Combining like terms ends in $4x+30^\circ=90^\circ$.
- Subtracting $30^\circ$ from both sides gives $4x=60^\circ$.
- Dividing both sides by $4$ gives $x=15^\circ$.
$~$
Paul
Let $x$ be the shorter length. So $1.5x$ is the longer length. Now we add the lengths to get $2x+1.5x=140$.
- First, we combine the like terms: $3.5x=140$.
- Next, we divide both sides by $3.5$ to get $x=40$.
$~$
Matt
All sides of a cube have the same length. The surface area is $6s^2$.
- The perimeter leads to $4s=8~in$ and dividing by $4$ results in $x=2~in$.
- Putting this in the surface area formula finally gives us $6\times (2~in)^2=24~in^2$.
Carol
Each isosceles triangle has two equal angles, the base angles which must be less than $90^\circ$.
So we get $2x+90^\circ=180^\circ$.
- We subtract $90^\circ$ to get $2x=90^\circ$.
- Next, we divide both sides by $2$, which tells us that the angles are $45^\circ$ each.
-
Find solutions to geometric problems.
HintsThe perimeter of a square is given by $4s$ and the area is given by $s^2$.
The sum of all interior angles of any triangle is 180 degrees. So, if one angle is a right angle, then the sum of the two other angles is $90^\circ$.
In a rectangle we can consider parallel lines of equal length.
The area is given by $A=lw$.
SolutionRectangle:
- Three sides are given. The desired one has the same length as the corresponding parallel side. So it's $12~in$.
- The area can be calculated by multiplying the side lengths: $A=(3~in)(12~in)=36~in^2$.
Square:
- All sides have the same length, it's $s$.
- The perimeter is given by the formula $24~in=4s$. Dividing by $4$ gives us the side length $=6~in$.
- Squaring the side length leads to the area $A=(6~in)^2=36~in^2$.
Triangle:
- The represented triangle has a right angle. Thus the sum of the other two angles is $90^\circ$.
- This leads to the equation $x+x+20=90$.
- Combining like terms and subtracting $20$ results in $2x=70$.
- Last we divide by $2$ to get $x=35^\circ$.
- The other angle is given by $x+20^\circ=55^\circ$.
-
Decide which statements are true.
HintsIn an isosceles triangle we have two equal sides and two equal angles.
At most one angle in a triangle is obtuse.
For example, a $3:2:1$ ratio leads to $3x+2x+x=180^\circ$ and thus $x=30^\circ$.
The three angles are
- $m\angle 1=30^\circ$
- $m\angle 2 = 60^\circ$
- $m\angle 3 = 90^\circ$
SolutionAn isosceles angle has two congruent angles. So either $m\angle 1=40^\circ=m\angle 2$ and thus $m\angle 3=100^\circ$, or $m\angle 1=40^\circ$ and $m\angle 20m\angle 3=70^\circ$. This means that the first statement is false, as there are two possibilities and not one given a right triangle with one angle of $40^\circ$.
The area of a square is $s^2$. If we double $s$, we get $(2s)^2=4s^2$. This is four times the area of the starting square. And so the second statement is false.
In a right triangle the remaining angles sum to $90^\circ$. If the smaller one (let it be $x$) is $18^\circ$ less than the larger one we get $x+x+18^\circ=90^\circ$. Subtracting $18^\circ$ and dividing by two after leads to $x=36^\circ$ and thus $54^\circ$ for the larger angle. The $5:3:2$ ratio leads to $5x+3x+2x=180^\circ$. Combining the like terms leads to $10x=180^\circ$. Next, we divide by $10$ to get $x=18^\circ$. So we get the following angles $2\times 18^\circ=36^\circ$, $3\times 54^\circ=36^\circ$ and $5\times 18^\circ=90^\circ$ So the third statement is true.
An equilateral triangle has three equal angles, each of $60^\circ$. Cutting such a triangle in half would divide one of these three angles into two $30^\circ$ angles. So each half would have one angle of $60^\circ$ and one of $30^\circ$. So find the remaining angle of these two triangles, we subtract $30$ and $60$ from $180$: $180-30-60=90$. And so each triangles has angles of $30^\circ$, $60^\circ$, and $90^\circ$. So the last statement is true.