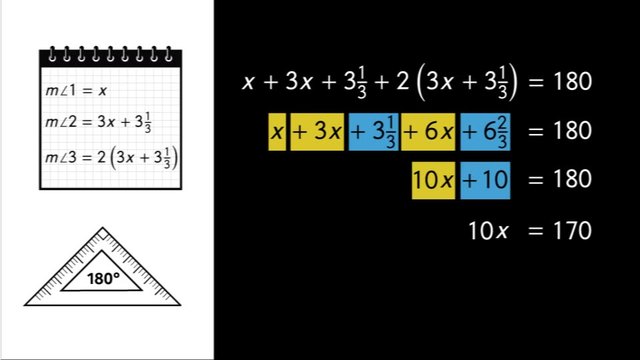
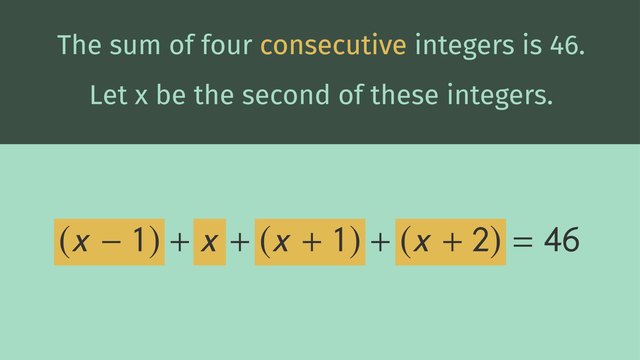Problem Solving with Multi-Step Equations


Basics on the topic Problem Solving with Multi-Step Equations
Problem-Solving with Multi-Step Equations – Introduction
The ability to solve multi-step equations is essential in algebra and lays the foundation for solving more complex problems. Learning the right strategies and understanding each step is like having the pieces to complete a puzzle.
Understanding Multi-Step Equations – Explanation
A multi-step equation is an algebraic equation that requires more than one operation, such as addition, subtraction, multiplication, or division, to solve.
It may involve variables on both sides, parentheses, and terms that need to be combined before finding a solution.
Steps to Solve Multi-Step Equations
| Step | Description | Solve the equation $2(3x + 4) = 20$ |
|---|---|---|
| Distributive Property | Apply the distributive property to remove parentheses. | $2 \times 3x + 2 \times 4 = 20 \rightarrow 6x + 8 = 20$ |
| Simplify Each Side by Combining Like Terms | Combine like terms on each side to simplify the expressions. | Since all terms are already simplified in this example, we proceed to the next step. |
| Rearrange the Equation | Rearrange the equation to isolate the variable terms on one side and constants on the other. | $6x + 8 - 8 = 20 - 8 \rightarrow 6x = 12$ |
| Perform Inverse Operations to Isolate the Variable | Use inverse operations to isolate the variable. This typically involves dividing or multiplying to eliminate coefficients. | $6x \div 6 = 12 \div 6 \rightarrow x = 2$ |
Multi-Step Equations – Example
Consider the equation $2(x + 3) = 4x$. Here's how to solve it step by step:
Step 1: Distribute $2$ across $(x + 3)$ to get $2x + 6$.
Step 2: Subtract $2x$ from both sides to get $6 = 2x$.
Step 3: Divide both sides by $2$ to isolate $x$ and find that $x = 3$.
Step 4: Check by substituting $x$ back into the original equation.
Try some on your own!
Problem-Solving with Multi-Step Equations – Summary
Key Learnings from this Text:
- A multi-step equation requires several steps to solve.
- Common operations include adding, subtracting, multiplying, and dividing.
- Distributive property, combining like terms, and moving variables to one side are crucial steps.
- Check your solution by substituting it back into the original equation.
Continue your journey by exploring systems of equations and other algebraic concepts that build on your knowledge of multi-step equations.
Problem Solving with Multi-Step Equations – Frequently Asked Questions
Transcript Problem Solving with Multi-Step Equations
The seventh graders from Whispering Pines Middle School are going on a field trip to a local theme park. During the field trip, they encounter some problem-solving with multi-step equations. In order to solve the problems given, they must be sure to read carefully to identify keywords and operations. Then, write a let statement to introduce a variable, and translate it, or turn the problem into an equation. Once an equation has been written, it can be solved to identify the solution. Let's look at the first problem the class has to solve. A group of three hundred thirty-one students are going on a field trip to a theme park. Seven of the students rode in cars to get there, but the rest rode in school buses. If each bus can fit fifty-four students, how many buses will they need to get there? The question reveals that the missing information is the number of buses, so let b equal buses. There are bus riders and car riders that combine to be our total. This is the total students, so three hundred thirty-one should be on one side of the equal sign. Seven of the students are riding in cars, so that is a constant. Lastly, if each bus fits fifty-four students, and buses is b, these terms must be multiplied since it is fifty-four students per bus. This equation can be solved, to find that b equals six, which means that the field trip will require six buses. Once at the park, one of the groups decides to ride the roller coaster called Dragon's Breath. Pause the video and read the problem carefully. The group has eleven students in it, and the roller coaster can fit four people in each row. Three are not riding the roller coaster. First, the let statement can identify the missing variable from the question, let r equal rows. Start building the equation with a total of eleven. From eleven, four riders per row can be subtracted. This equals three, which are the remaining students in the group. When the equation is solved it's concluded that the group will occupy two rows on the roller coaster. Let's take a look at the last example. Remember to identify keywords, write a let statement, and then translate the problem into a proper equation. Pause the video and try it on your own. The let statement will be, let f equal friends since the question asks us to find out how many friends got ducks. And the equation can look like this, or this, or this, depending on how you interpreted the word problem. When any of these equations are solved, it's evident that this student has shared the ducks with five friends. Let's summarize problem-solving with multi-step equations. Remember, it is important to read carefully to identify words that will help us translate it into an equation. Also, be sure to write a let statement to introduce the variable we will use. Lastly, translate it into an equation, solve it, and identify the solution. Problem-solving with equations is something that will be useful in life, not only at the theme park, but also when making purchases, preparing meals, and making many everyday decisions. And now that we have solved all these problems, let's go celebrate by riding some more theme park rides!
Problem Solving with Multi-Step Equations exercise
-
What are the steps when solving problems with multi-step equations?
Hints- Read the word problem carefully to identify any key information first.
- We can then introduce a variable (for example $a$ or $b$ or any letter).
- Write down the information using the variable.
When you have the variable translate it into an equation so you can solve it.
For example, the cost of a taxi ride = $£3$ for each mile ($m$) plus $£2$ standing charge.
We would write $C$ = $3m$ + $2$ as an equation.
Solution- Identify key words and operations.
- Introduce a variable and make a statement.
- Translate the statement into an equation
- Solve the equation.
-
Transfer the statement to an expression.
HintsTo change the statement into an expression we are shortening the information.
For example, $5$ lots of $n$ can be written as $5n$ in short.
If Maisy is $g$ years old and Molly is $4$ years older, then Molly's age is $g + 4$.
Solution- $3$ more than $a$, we can write as: $a + 3$
- $3$ lots of $a$, we can write as $3a$
- $3$ less than $a$, we can write as $a - 3$
- $3$ times $a$, then add $3$, we can write as $3a + 3$
-
Turn the problem into a formula.
HintsIdentify the key words and form a statement.
Grandma makes $51$ cup cakes.
Grandpa eats $3$ cakes and the others are put in boxes, ($b$) of $12$.
- Total cakes is $51$
- We multiply the boxes, ($b$) by $12$ (as $12$ cakes fit in each box)
- We need to add $3$ for the ones which were given to Grandpa.
Solution$51 = 12b + 3$
- Total cakes is $51$
- We multiply the boxes, ($b$) by $12$ (as $12$ cakes fit in each box)
- We need to add $3$ for the ones which were given to Grandpa.
-
Solve the multi-step equation.
Hints- Identify the key words and form a statement
- Let $t$ be Tim's age and form an equation from the statement
- Solve the equation to find $t$
- Let Tim's age be $t$
- Let Tam's age be $t - 2$
- Translate the statement into an equation
- $t + t - 2 = 28$
- Solve the equation
SolutionTim is $15$ years old
- Let Tim's age = $t$
- Let Tam's age = $t - 2$
- $t + t - 2 = 28$
- Solve for t.
$2t = 28 + 2$
$2t = 30$
$t = 30 \div 2$
$t = 15$ years old
-
Solve the multi-step equation.
HintsIdentify the keywords and form a statement.
The gardener charges $£85$ for $£15$ times the hours worked plus $£10$ call out fee.
Total Charge: $=15\times$ Hours $+ 10$
Let Hours be $h$
$85 = 15h + 10$
$15h + 10 = 85$
Solve the equation by first subtracting $10$ from both sides.
$15h = 75$
$15h = 75$
Divide by $15$ next.
Solution- Let hours be $h$, $15h + 10 = 85$.
- Subtract $10$ from both sides, $15h = 75$
- Divide both sides by $15$, $h = 75 $\div 15$
- $h = 6$ hours.
-
Solve the multi-step equation.
HintsThe perimeter is all the way around the rectangle.
We add all $4$ lengths together.
Identify the key words and form a statement.
Height is $L$.
Base is $2L + 5$.
The perimeter (all the way around the rectangle) $ = 52$
Translate the statement into an equation.
$L + L + 2L + 5 + 2L + 5 = 52$
Simplify, then solve the equation.
Solution$\bf{L = 7}$ cm
Height $ = L$
Base $ = 2L + 5$
There are $2$ heights and $2$ bases in the perimeter.
$L + L + 2L + 5 + 2L + 5 = 52$
$6L + 10 = 52$
Subtract $10$ from both sides.
$6L = 42$
Divide both sides by $6$.
$L = 42 \div 6$
$L = 7$ cm