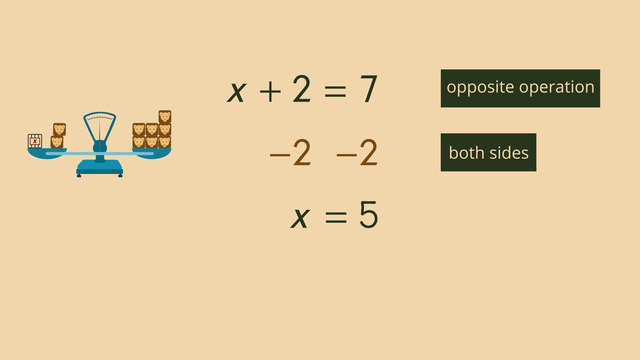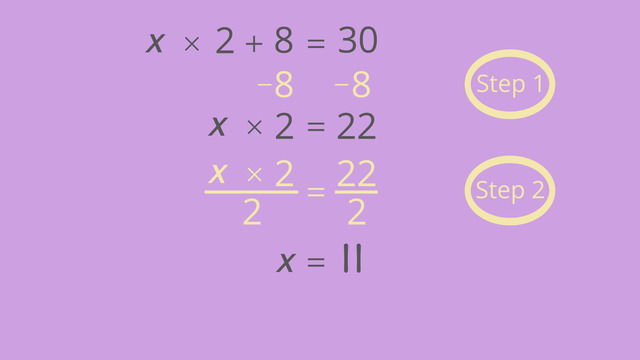Solving Multi-Step Equations with Variables on One Side

Basics on the topic Solving Multi-Step Equations with Variables on One Side
Linear equations needing more than one step to solve are called multi-step equations. When the variable is on just one side of the equal sign, you can follow these steps to solve for the value of the unknown variable.
First, if there are parentheses, use the Distributive Property to multiply the number outside the parentheses across the terms that are inside the parentheses. Second combine all the like terms. Numbers alone, also called constants, are like terms, and same variables and same variables attached to numbers, known as a coefficients, are also like terms. The third step, you can use opposite (inverse) operations in the order of reverse PEMDAS. To isolate the variable, use inverse (opposite) operations to add or subtract on both sides of the equal sign, then use inverse operations to multiply or divide to completely isolate the variable leaving, finally, the solution to the linear equation.
After following the multiple steps of multi-step equations, there is one final step – check your work. You can do this by substituting the value of the variable back into the original equation, and use the order of operations (PEMDAS, BEDMAS, BODMAS, BIDMAS, operator precedence) to simplify. If you have solved the equation correctly, the left side of the equal sign will be the same as the right side.
Explain steps to solve algebraic equations. CCSS.MATH.CONTENT.HSA.REI.A.1
Transcript Solving Multi-Step Equations with Variables on One Side
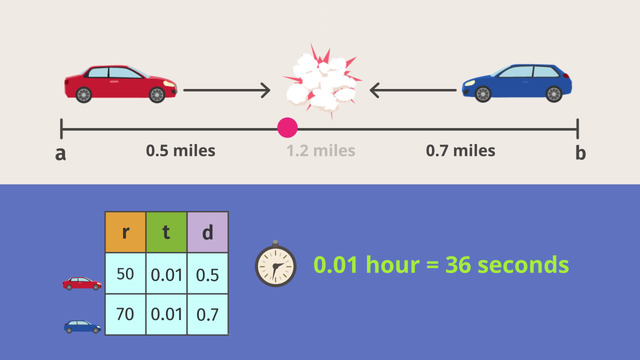
Meet Kayla and Sam. They are at the fair and are deciding on how to spend their money on the different attractions.
They want to ride all the rides. The price is 8 dollars per ticket per rollercoaster. They definitely also want to ride the ferris wheel together at least once. The price for the ferris wheel is 5 dollars per ticket.
Sam has a sweet tooth and he can't have a visit to the amusement park without cotton candy. So he will sacrifice a ride on the rollercoaster so he has some money for cotton candy. Cotton candy costs $2.
Together, Kayla and Sam have one hundred dollars. They both want to ride the rollercoasters as often as possible. Our question is: How many rollercoasters can they ride before they run out of money?
To determine the number of times each of them can ride the rollercoaster, we can use a linear equation.
Writing the Linear Equation
Let's collect our information and translate this problem into a linear equation:
- The rollercoaster is 8 dollars per ride.
- To ride the ferris wheel Kayla and Sam each have to pay 5 dollars.
- Cotton candy costs 2 dollars.
- Together they have 100 dollars to spend.
- Sam buys 1 stick of cotton candy.
- They both take a ride in the ferris wheel, which is 2 tickets.
We don't know the number of rides they can take on the rollercoaster, so let x be Kaylas number of rides. Since Sam takes one ride less in order to get some cotton candy, his number of rides can be represented by x − 1.
Let's write our equation:
- 8 Dollars for the roller coaster times x for the number of rides Kayla takes.
- Add another 8 dollars for the rollarcoaster times (x-1) for Sam's rides.
- Then, you add 5 dollars twice for the ferris wheel ride for Kayla and Sam
- Now, you add 2 dollars for the cost of Sam's cotton candy.
- They have one hundred dollars to spend so you put this on the right side of the equation.
So now you have a linear equation with the variable x on only one side. You can solve this equation for x to find out how often Kayla and Sam can ride the roller coaster.
Solving the Linear Equation
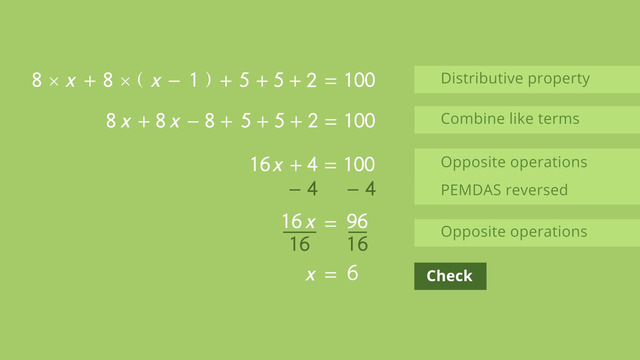
To start solving this linear equation, you need to follow the order of operations and, using the distributive property, multiply both terms inside the parentheses by eight. Now you have: 8x + 8x − 8 + 5 + 5 + 2 = 100.
On the left side of the equation, combine like terms: Combine the x terms and the integer terms together. The result of this step is 16x + 4 = 100.
Now solve the equation by using opposite operations. Remember that you use PEMDAS reversed. 4 is subtracted from both sides of the equation to obtain all integer values on the right side of the equation. The result of this step is 16x = 96.
To solve for x, use opposite operations again: both sides are divided by 16 in order to isolate the x value on one side of the equation. The solution to the equation is x = 6.
Now, let's check this solution. Substitute the value of 6 in for x. We'll simplify by using the order of operations. First look at the parentheses. Then, complete the multiplication.
Last, you can add all values on the left hand side of the equation. The value on the left side and the right side of the equation are equal. So the solution to the equation x equals 6 is the correct value for x.
So, what does this mean? Remember: We said x was for the number of rides Kayla can take on the roller coaster. So she can take 6 rides. Sam goes 1 less time or x − 1. This is 6 − 1, which is 5. Great!
Now they're finally on the roller coaster. But maybe Sam shouldn't have spent those 2 dollars on the cotton candy right before the rides.
Solving Multi-Step Equations with Variables on One Side exercise
-
Determine the best answer for each blank.
HintsRemember that Sam rides the roller coaster one time fewer than Kayla.
You can write all the money Kayla and Sam want to spend on the one side of the equation and the money they have to spend on the other side.
One roller coaster ride costs $\$8$, so four rides cost $\$8\times 4=\$32$.
SolutionWe want to know how many times Kayla and Sam can each ride the roller coaster. To figure this out, we assign the variable $x$ to the number of times Kayla can ride the roller coaster. Because Sam rides the roller coaster one time fewer than Kayla, he rides the roller coaster $x-1$ times.
We can write the expression: $8\times x+8\times (x-1)$
Pay attention to the parentheses. They are very important.
Both Kayla and Sam ride the Ferris wheel just once. This gives us the expression $5+5$.
Last but not least, Sam buys some cotton candy for $\$2$.
We must add all of these expenses: $8\times x+8\times (x-1)+5+5+2$.
Because they have $\$100$ to spend, the expression is set to equal $\$100$. This gives us the following equation:
$\$8\times x+\$8\times (x-1)+\$5+\$5+\$2=\$100$
-
Solve the equation.
HintsYou solve these equations by simplifying using the Distributive Property then Combining Like Terms and finally Isolating the Variable.
To Isolate the Variable, we use Opposite Operations:
- $+~\longleftrightarrow~-$
- $\times~\longleftrightarrow~\div$
Use Opposite Operations:
- Multiplication ($\times~\longleftrightarrow~\div$)
- Division ($\div~\longleftrightarrow~\times$)
- Addition ($+~\longleftrightarrow~-$)
- Subtraction ($-~\longleftrightarrow~+$)
SolutionOur equation is $8\times x+8\times (x-1)+5+5+2=100$.
First, we use the Distributive Property to simplify $8\times(x-1)$ to $8x-8$:
$8x+8x-8+5+5+2=100$
Next, we Combine Like Terms on the left side of the equation:
$16x+4=100$
Now we can solve the equation by using Opposite Operations:
$\begin{array}{rcrcl} 16x+4&=&100\\ \color{#669900}{-4}&&\color{#669900}{-4}&&| (\div~\leftrightarrow~\times) \text{ Opposite Operations}\\ 16x&=&96\\ \color{#669900}{\div16}&=&\color{#669900}{\div16}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&6 \end{array}$
What does this solution mean?
- Carla rides the roller coaster $6$ times
- Sam can only ride $5$ times because he bought cotton candy instead.
-
Evaluate how many times Kayla and Sam can ride the roller coaster.
Hints- Organize the given information first.
- Identify your variable.
- Set up your equation.
Use the opposite operations:
- Multiplication ($\times~\longleftrightarrow~\div$)
- Division ($\div~\longleftrightarrow~\times$)
- Addition ($+~\longleftrightarrow~-$)
- Subtraction ($-~\longleftrightarrow~+$)
You can solve each equation by using the Distributive Property, Combining Like Terms and using Opposite Operations.
SolutionFirst, we have to set up the equation for each situation.
$\mathbf{\$88}$ to spend:
$8\times x+8\times(x-2)+5+5+5+5+2+2=88$.
Now we solve the equation as follows:
$\begin{array}{rcrcl} 8\times x+8\times(x-2)+5+5+5+5+2+2 &=&88&&|\text{ Distributive Property}\\ 8x+8x-16+5+5+5+5+2+2 &=&88&&|\text{ Combining Like Terms}\\ 16x+8 &=&88\\ \color{#669900}{-8}&&\color{#669900}{-8}&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ 16x &=&80\\ \color{#669900}{\div16}&&\color{#669900}{\div16}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&5 \end{array}$
For $x=5$, this means that Carla rides the roller coaster five times while Sam rides it only three times.
$\mathbf{\$119}$ to spend:
$8\times (x-1)+8\times x+5+5+5=119$.
Now we solve the equation as follows:
$\begin{array}{rcrcl} 8\times (x-1)+8\times x+5+5+5&=&119&&|\text{ Distributive Property}\\ 8x-8+8x+5+5+5&=&119&&|\text{ Combining Like Terms}\\ 16x+7&=&119\\ \color{#669900}{-7}&&\color{#669900}{-7}&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ 16x&=&112\\ \color{#669900}{\div16}&&\color{#669900}{\div16}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&7 \end{array}$
For $x=7$, this means that Sam rides the roller coaster seven times while Kayla rides it six times.
$\mathbf{\$108}$ to spend:
$8\times x+8\times x+5+5+5+5+2+2+2+2=108$.
Now we solve the equation as follows:
$\begin{array}{rcrcl} 8\times x+8\times x+5+5+5+5+2+2+2+2&=&108&&|\text{ Combining Like Terms}\\ 16x+28&=&108\\ \color{#669900}{-28}&&\color{#669900}{-28}&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ 16x&=&80\\ \color{#669900}{\div16}&&\color{#669900}{\div16}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&5 \end{array}$
For $x=5$, this means that Kayla and Sam ride the roller coaster five times each.
$\mathbf{\$98}$ to spend:
$8\times x+8\times x+5+5+5+5+5+5+2+2=98$.
Now we solve the equation as follows:
$\begin{array}{rcrcl} 8\times x+8\times x+5+5+5+5+5+5+2+2&=&98&&|\text{ Combining Like Terms}\\ 16x+34&=&98\\ \color{#669900}{-34}&&\color{#669900}{-34}&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ 16x&=&64\\ \color{#669900}{\div16}&&\color{#669900}{\div16}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&4 \end{array}$
For $x=4$, this means that Kayla and Sam both ride the roller coaster four times.
-
Determine how many bags of candy Kayla and Sam can buy.
HintsUse the Distributive Property:
$a\times (b+c)=a\times b + a\times c$
- The opposite operation of $+$ is $-$, and vice versa.
- The opposite operation of $\times$ is $\div$, and vice versa.
SolutionLet's write a math equation to describe the situation:
Teddy bears cost $\$2$ each Balloons cost $\$1$ each
They buy two teddy bears one balloon, giving us:
- $2+2+1$
- $1.50\times x+1.50\times(x-1)$
$1.50\times x+1.50\times(x-1)+2+2+1=21.50$.
We solve this equation as follows:
$\begin{array}{rcrcl} 1.50\times x+1.50\times(x-1)+2+2+1&=&21.50&&|\text{ Distributive Property}\\ 1.50x+1.50x-1.50+2+2+1&=&21.50&&|\text{ Combining Like Terms}\\ 3x+3.5&=&21.5\\ \color{#669900}{-3.5}&&\color{#669900}{-3.5}&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ 3x&=&18\\ \color{#669900}{\div3}&&\color{#669900}{\div3}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&6 \end{array}$
$x=6$ is the number of bags of candy Sam buys. Kayla buys one bag fewer. She buys $5$ bags. Together they buy $6+5=11$ bags of candy.
-
Describe how to solve an equation.
HintsImagine an equation to be like a scale in balance:
- You can move things on one or both sides of the scale but if you remove something from one side of the scale, you have to remove the same something on the other side as well.
For example
$\begin{array}{rcr} 6x+4&=&100\\ \color{#669900}{-4}&&\color{#669900}{-4}\\ 6x&=&96 \end{array}$
is correct, but
$\begin{array}{rcr} 6x+4&=&100\\ \color{#669900}{-4}&&\\ 6x&=&100 \end{array}$
isn't.
The opposite operations are:
- $+~\longleftrightarrow~-$
- $\times~\longleftrightarrow~\div$
SolutionAn equation is like a scale in balance: We have terms on both sides of the equal sign.
We can modify the equation by using the Distributive Property or by Combining Like Terms.
Then to solve, we should Isolate the Variable by using Opposite Operations on both sides of the equation.
-
Find and solve the equation for the given situation.
HintsExample: If $x$ is the unknown value, two more would be $x+2$.
The number of cats is the same in both examples.
To isolate the variable $x$ we use opposite operations
- $+~\longleftrightarrow~-$
- $\times~\longleftrightarrow~\div$
SolutionRemember how to solve multi-step equations:
- Write the equation.
- Solve the equation.
- Let the unknown number of cats be equal to $x$.
- There are three more cats than dogs. $x-3$ is the number of dogs.
- The total cans of food: $3\times x+2\times (x-3)$.
- The pets consume 19 cans of food altogether:
We use the Distributive Property then Combine Like Terms and finally Isolate the Variable as follows:
$\begin{array}{rcrcl} 3\times x+2\times (x-3)&=&19&&|\text{ Distributive Property}\\ 3x+2x-6&=&19&&|\text{ Combine Like Terms}\\ 5x-6&=&19\\ \color{#669900}{+6}&&\color{#669900}{+6}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ 5x&=&25\\ \color{#669900}{\div5}&&\color{#669900}{\div5}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&5 \end{array}$
$x=5$ is the number of cats. Subtracting $3$ gives us the number of dogs, $2$.
$\mathbf{\$3,400}$
- Let the unknown number of cats be equal to $x$.
- There is one more dog than there are cats: $x+1$ is the number of dogs.
- The cost for the cats and the dogs is $200\times x+400\times (x+1)$.
- Together Miss Crazycat and Miss Crazydog spent $\$3400$ on their pets:
Here we use the Distributive Property then we Combine Like Terms and finally we Isolate the Variable as follows:
$\begin{array}{rcrcl} 200\times x+400\times (x+1)&=&3400&&|\text{ Distributive Property}\\ 200x+400x+400&=&3400&&|\text{ Combine Like Terms}\\ 600x+400&=&3400\\ \color{#669900}{-400}&&\color{#669900}{-400}&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ 600x&=&3000\\ \color{#669900}{\div600}&&\color{#669900}{\div600}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&=&5 \end{array}$
$x=5$ is the number of cats. Adding $1$ gives us the number of dogs, $6$.