Solving Systems of Equations by Substitution


Basics on the topic Solving Systems of Equations by Substitution
Systems of equations, also called simultaneous equations, are problems with two or more equations having the same variables. To determine the solution to the system, or the point where the equations intersect, there are several methods: graphing, substitution, and elimination.
This video investigates how to use substitution to solve systems of linear equations. To solve using substitution for a system with two equations, you must find the value of one of the variables in terms of the other and substitute it into one of the equations, allowing you to know the value for one of the variables. Next, substitute the value of that variable into the other equation and solve for the second variable. After you know the solution for both variables, plug them back into the system to verify they work.
There are a lot of steps to solving this type of problem, and you know what they say: the more the steps, the greater the chance of making a silly mistake. But keep in mind, if you get a funny answer, it could be that there is no solution, or the entire line could be the solution. If this process of solving systems of equations with substitution seems confusing, then you had better watch this video, so you can see an example worked out and have a great time while you do.
Solve systems of equations to find solutions to problems.
CCSS.MATH.CONTENT.HSA.REI.C.5
CCSS.MATH.CONTENT.HSA.CED.A.3
Transcript Solving Systems of Equations by Substitution
Mary Anderson might seem like your ordinary neighbor, except for one thing. She has a mind-bending number of cats. Don't ask us why, or how - she just does.
There's a cat show coming up and the cash rewards are huge. So Mary plans to bring as many cats as possible.
She knows her short-haired cats are better looking than the long-haired ones, so she decides to bring 7 times more short-haired cats than long-haired ones.
Now here's the thing: Mary can only spend a certain amount on cat grooming, and the local stylist isn't cheap. He charges 18 dollars for cats with short hair and 36 dollars for cats with long hair.
Her cat styling budget is $1,944. She needs to figure out how many long- and short-haired cats to bring.
Setting up a System of Equations
Let's help her by solving sytems of equations. Let the variable x denote the number of short-haired cats she brings, and let y be the number of long-hair cats. We'll write two equations to help her.
Grooming short-haired cats cost $18, so 18x represents the cost to groom all the short-haired cats she wants to bring. To groom long-haired cats costs $36, so we can write this as 36y.
Added together, these terms give the total cost of grooming cats for the show. She has a budget of $1,944.
The second equation comes from the fact that she wants to bring 7 times more short-haired cats than long-haired ones.
That means that x, the number of short-haired cats, equals 7 times the number of long-haired cats.
Solving a System of Equations
Now take a close look at this second equation: it tells us that x has exactly the same value as 7y.
This means we can substitute 7y for x in the first equation. Why would we want to do that? Well, now that we only have one variable, we can solve for y. We've got two like terms on the left, so let's combine them.
- First, 18 times 7y is 126y.
- 126y plus 36y is 162y, so we have 162y = 1944.
- Dividing both sides by 162, we see that y = 12.
- To find x, substitute 12 into our second equation: x = 7y.
- We see x equals 7 times 12, or 84.
Therefore, Mary can bring 12 long-haired cats and 84 short-haired cats. Let's check our work.
Checking the Solution
If these numbers are correct, then they must satisfy both of the equations. That means if we substitute 12 and 84 for x and y, respectively, then simplify, we should get the same values on either side of the equal signs.
So let's check. After multipying, we have 1512 + 432, giving us 1,944. That's equal to the right-hand side, so these values of x and y satisfy the equation.
In the second equation, we know 84 = 7 * 12, so we're good here, too, which means all our work checks out. Mary can definitely bring 12 long-haired cats and 84 short-haired ones.
Alltogether, that's 96 cats to groom. She brings them to the groomers for same-day service. He basically just blow dries them all at once. She picks them up and finally arrives at the show.
Poor Mary: She missed one important detail: this cat show is only for Sphynx cats - cats without hair. What's this? Mary seems to have an idea...
Solving Systems of Equations by Substitution exercise
-
Explain how to solve a system of equations by substitution.
HintsHere is an example of a system of equations:
$\begin{align*} x+y&=4\\ x&=y \end{align*}$
You can substitute $x=y$ into the first equation to get $y+y=4$. This is equivalent to $2y=4$. Dividing by $2$ leads to $y=2$.
SolutionFollow these steps for any word problem with more than one variable:
- First, establish a system of equations by assigning variables to the unknown values and write the given information into equations. Remember: You can solve systems of equations with two variables - as long as you have at least two different equations.
- Next, we are looking for a variable that is already isolated on one side. We plug in the other side of that equation for the variable in the second equation.
- Now, we only have one equation with one variable left, which we can solve by isolating the variable.
- But there is still another variable! To solve for the second variable, we plug in the calculated value for the first variable into the second equation.
- You can check your solutions by substituting them into both equations.
-
Determine the number of long-haired and short-haired cats by substitution.
HintsFollow these steps to solve this system of equations:
- First, establish the system of equations.
- Look for an equation with an isolated variable and substitute the other side of the equation for the variable in the second equation.
- Solve the equation that has only one variable left.
- Substitute the solution of the first variable into the equation with two variables and solve for the one you still don't know.
If Miss Anderson has $4$ long-haired cats, and if she has seven times more short-haired cats, she has $7\times4=28$ short haired cats.
Use the opposite operations:
- Multiplication ($\times~\longleftrightarrow~\div$)
- Division ($\div~\longleftrightarrow~\times$)
- Addition ($+~\longleftrightarrow~-$)
- Subtraction ($-~\longleftrightarrow~+$)
SolutionEstablish the System of Equations
- Let's use $x$ for the number of short-haired cats and $y$ for the number of long-haired cats.
- Because we have $\$1944$ to spend on grooming, and the cost to groom one short-haired cat is $\$18$ and the cost for long-haired cats is $\$36$, we get: $18x+36y=1944$
- We still need another equation. Miss Anderson has seven times more short-haired cats than long-haired cats, $x=7y$.
$\begin{align*} 18x+36y&=1944\\ x&=7y \end{align*}$
Substitution
Substitute $x=7y$ into the equation $18x+36y=1944$.
Doing this, we get:
$18(7y)+36y=1944$
We have just one variable left, $y$.
Solve the equation to get $y$
$\begin{array}{rclcl} 18(7y)+36y&=&1944&&|\text{ multiply}\\ 126y+36y&=&1944&&|\text{ combine like terms}\\ 162y&=&1944 \end{array}$
Finally, we divide by $162$ to get $y=12$.
Substitute to get the value for $x$
We substitute $y=12$ into the equation $x=7y=7\times 12=84$.
Now we know that Miss Anderson can take $12$ short-haired and $84$ long-haired cats to the cat stylist.
-
Decide how many dogs Miss Lovingdogs can bring to the dog stylist.
HintsYou have to multiply the cost per dog by the number of dogs. The budget is the sum of the number of poodles and the number of dachshunds.
We can write a system of equations using the given information:
- $20x+35y=475$
- $x=3y$
SolutionEstablish the system of equations
- We'll use $y$ to represent the number of poodles and $x$ for the number of dachshunds.
- Because the cost to style one dachshund is $\$20$, and the cost to style poodles is $\$35$, we get the expression $20x+35y$.
- Since Miss Lovingdogs has a budget of $\$475$, our final equation is $20x+35y=475$
- Let's have a look at the other equation: Miss Lovingdogs has three times more dachshunds than poodles, which gives us $x=3y$.
$\begin{align*} 20x+35y&=475\\ x&=3y \end{align*}$
Substitution
Substitute $x=3y$ into the first equation $20x+35y=475$:
$20(3y)+35y=475$
Now we have one equation with only one variable, $y$.
Solve the equation to get $y$
$\begin{array}{rclcl} 20(3y)+35y&=&475&&|&\text{ Multiply}\\ 60y+35y&=&475&&|&\text{ Combine Like Terms}\\ 95y&=&475\\ \color{#669900}{\div95}&&\color{#669900}{\div95}\\ y&=&5 \end{array}$
Substitute this value for y to get $x$
We substitute $y=5$ into the second equation:
$x=3y=3\times 5=15$
We know now that Miss Lovingdogs can take $5$ poodles and $15$ dachshunds to the dog stylist.
-
Write a system of equations for each situation and solve them.
HintsKeep in mind the difference between
- four times as many as $=\times 4$
- four more $=+4$.
Use the Distributive Property if necessary:
$a\times(b+c)=a\times b+a\times c$.
You can check each pair of solutions.
SolutionHere we have different examples of similar word problems. To set up each of our equations, we multiply the costs of our choices (cookies or lollipops) by the corresponding variables and add the products to equal to the given budget.
For each of the problems:
- $x=$ the unknown number of cookies
- $y=$ the unknown number of lollipops
- Cookie: $\$2$
- Lollipop: $\$0.50$
- One more lollipop than cookies.
- First equation: $2x+0.5y=30$
- Second equation: $2x=y$
They can buy $10$ cookies and $20$ lollipops.
$~$
(B)
- Cookie: $\$1.50$
- Lollipop: $\$2$
- One more lollipop than cookies.
- First equation: $1.5x+2y=30$
- Second equation: $x+1=y$
They can buy $8$ cookies and $9$ lollipops.
$~$
(C)
- Cookie: $\$2$
- Lollipop: $\$0.50$
- Same number of cookies as lollipops.
- First equation: $2x+0.5y=30$
- Second equation: $x=y$
They can buy $12$ cookies and $12$ lollipops.
$~$
(D)
- Cookie: $\$1.50$
- Lollipop: $\$2$
- Three times as many lollipops as cookies.
- First equation: $1.5x+2y=30$ (same as (B))
- Second equation: $3x=y$
They can buy $4$ cookies and $12$ lollipops.
-
Determine the two equations that are needed to correctly describe Miss Anderson's problem.
HintsIf the number of long haired cats is $3$, the number or short haired cats is $7\times 3=21$. Which equation reflects this information?
SolutionFirst, we write a system of equations using the given information.
We'll use $x$ for the number of short-haired cats and $y$ for the number of long-haired cats.
Next, we take a look at the costs:
- Miss Anderson has a budget of $\$1944$.
- The styling cost for short-haired cats is $\$18$ and $\$36$ for long-haired cats.
- The equation to describe this situation is $18x+36y=1944$.
So we have the following system of equations:
$\begin{align*} 18x+36y&=1944\\ x&=7y \end{align*}$
-
Solve the system of equations.
HintsDivide the second equation by $2$ to isolate $y$.
Substitute $2x$ for $y$ in the first equation.
To solve an equation with only one variable:
- First combine like terms
- Then use opposite operations
Use the opposite operations:
- Multiplication ($\times~\longleftrightarrow~\div$)
- Division ($\div~\longleftrightarrow~\times$)
- Addition ($+~\longleftrightarrow~-$)
- Subtraction ($-~\longleftrightarrow~+$)
SolutionTo be able to substitute one variable into the other equation, we need to have the variable isolated on one side of the equation.
Let's take a look at the second equation $4x=2y$. Dividing by $2$ gives us $2x=y$.
Great, this looks familiar. Next, we substitute $2x$ for $y$ in the first equation, $4x+6y=192$:
$4x+6(2x)=192$
Simplifying and combining like terms gives us
$16x=192$
Finally, we divide by $16$ to get $x=12$.
We substitute $12$ for $x$ into the modified equation $y=2x=2\times 12=24$.
To be sure, we check the solution:
$\begin{align*} 4x+6y&=192\\ 4\times 12+6\times 24&=192\\ 48+144&=192\\ 192&=192~\surd\\ \\ 4x&=2y\\ 4\times 12&=2\times 24\\ 48&=48~\surd \end{align*}$
Great!



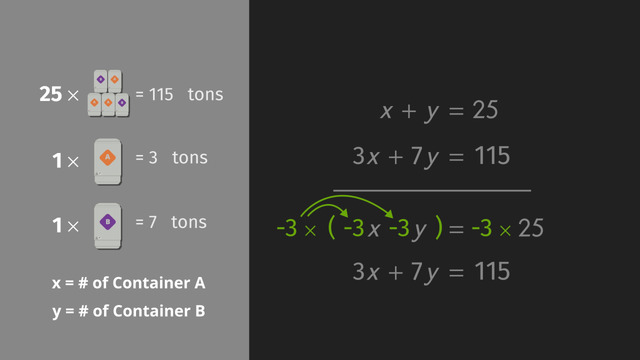











Me and my sister love CATZ
this would be our house susanne
One of my favorite Videos. :)