Writing Products as Sums and Sums as Products

Writing Products as Sums and Sums as Products exercise

• Explain how to express the product $4(32x+2)$ as a sum.

  Hints

  Here you see the distributive property.

  Take care of the notation

  • $a+b$ is a sum.
  • $a\times b$ is a product.

  Just look at the following example that looks quite similar:

  $5(4x+2)=(5)(4x)+(5)(2)=20x+10$.

  Solution

  The last year results of Ice Queen can be expressed by the term $32x+2$, where $x$ is the number of seconds.

  With rigorous training, the Ice Queen improved last year's results by a factor $4$. So we have to multiply the sum above by $4$ to get $4(32x+2)$.

  Next we use the distributive property to get $4(32x+2)=(4)(32x)+(4)(2)$.

  Last, we combine like terms: $4(32x+2)=128x+8$.

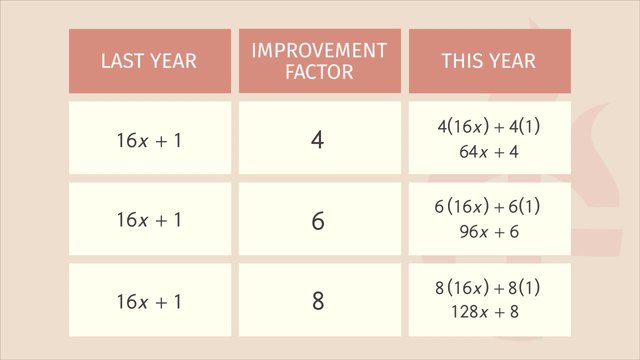
• Determine how the different factors alter the expression $16x+1$.

  Hints

  Use the distributive property, $a(b+c)=(a)(b)+(a)(c)$.

  For example, $4(16x+1)=(4)(16x)+(4)(1)$.

  Solution

  Here you see the complete table.

  Let's fill it in step by step.

  In the left column you see last year's results of En Fuego.

  In the middle column, the improvement factor is written down.

  You have to multiply last year's results with this factor each time to get the improvement results for this year:

  • $4(16x+1)=(4)(16x)+(4)(1)=64x+4$
  • $6(16x+1)=(6)(16x)+(6)(1)=96x+6$
  • $8(16x+1)=(8)(16x)+(8)(1)=128x+8$

• Explain how to express the sum $120x+16$ as a product.

  Hints

  You can also factor out $8$:

  • $\frac{120x+16}8=15x+2$
  • $120x+16=8(15x+2)$

  You can check the resulting product using the distributive property.

  Solution

  To write this sum as a product we first look for all factors of the first as well as the second summand.

  • $120x=(2)(2)(2)(3)(5)(x)$
  • $16=(2)(2)(2)(2)(2)$

  Now we can conclude that $2$, $4$, and $8$ are common factors of both summands. So we can factor out the common factor.

  Let's start with the factor $2$:

  For this we divide the whole sum by $2$: $\frac{120x+16}2=60x+8$.

  The other way round we can write the common factor in front of a set of parentheses $2(~~~)$. Inside the parentheses we write the sum $60x+8$ to get in total

  $120x+16=2(60x+8)$.

  Similarly we can factor out $4$:

  • $\frac{120x+16}4=30x+4$
  • $4(~~~)$ and last we put the sum $30x+4$ inside the parentheses.

  $120x+16=4(30x+4)$.

  Once again we factor out $8$.

  • $\frac{120x+16}8=15x+2$
  • $8(~~~)$ and last we put the sum $15x+2$ inside the parentheses.

  $120x+16=8(15x+2)$.

  Last we can state that each product is correct. Usually one factors out the greatest common factor. Here it's $8$.

• Write each sum as a product.

  Hints

  You can check each product using the distributive property.

  Keep in mind, that you can factor out any factor in common.

  For example, $24x+16=2(12x+8)=4(6x+4)=8(3x+2)$.

  Solution

  To write a sum as a product we have to find factors in common for both summands.

  The other way round we can check the product using the distributive property. So we can state that factoring and using the distributive property are opposite operations.

  Let's start with the sum $125x+15$.

  • $125x=(5)(5)(5)(x)$
  • $15=(3)(5)$

  The factor in common is given by $5$. So we divide each summand by $5$ to get $125x+15=5(25x+3)$.

  $~$

  The next sum is $132x+33$.

  • $132x=(2)(2)(3)(11)(x)$
  • $33=(3)(11)$

  The summands have the common factors $3$, $11$, and $33$. Each of those factors can be factored out. So factor out their product, $33$. Dividing each summand by $33$ we get $132x+33=33(4x+1)$.

  $~$

  The next sum is given by $120x+30$.

  • $120x=(2)(2)(2)(3)(5)(x)$
  • $30=(2)(3)(5)$

  The factors in common are $2$, $3$, $5$, $6$, $10$, $15$, and $30$. You can factor out each of those factors. Here we factor out $10$ to get $120x+30=10(12x+3)$.

  $~$

  There is just one sum left: $136x+8$.

  • $136x=(2)(2)(2)(17)(x)$
  • $8=(2)(2)(2)$

  So $2$, $4$, and $8$ are common factors. Here we factor out the greatest common factor $8$ to get the product, $136x+8=8(17x+1)$.

• State how to express the product $4(16x+1)$ as a sum.

  Hints

  If you multiply a factor by a sum, you use the distributive property, $a(b+c)=(a)(b)+(a)(c)$.

  Combining like terms means, for example, $(5)(2)(y)=10y$.

  Here the numbers $5$ as well as $2$ have been multiplied to $10$.

  The result on the right side should be a sum.

  Solution

  First we multiply the factor by each sum in the parentheses:

  • $(4)(16x)$
  • $(4)(1)$

  Next we combine like terms:

  • $(4)(6x)=64x$
  • $(4)(1)=4$

  Last, we add the resulting terms to get the sum, $4(16x+1)=64x+4$.

• Represent products as sums and vice versa.

  Hints

  There is one calculation without any mistake.

  To write a product as a sum use the distributive property.

  Just look at the example beside.

  To write a sum as a product look for the common factors of all given terms. For example,

  • $24x=(12)(2x)$
  • $12y=(12)(y)$
  • $36=(3)(12)$

  The common factor between these terms is $12$.

  Next divide each term by the common factor.

  You can check your factorization by using the distributive property.

  Solution

  Keep the following in mind:

  • To write a product as a sum use the distributive property.
  • The other way round, to write a sum as a product look for common factors of all given terms.

  Let's practice:

  $~$

  $\mathbf{124x+24}$

  • $124x=(2)(2)(31)(x)$
  • $24=(2)(2)(2)(2)(3)$

  One common factor is $4$. Next we divide each term by this factor:

  • $124x\div 4=31x$
  • $24\div 4=6$

  So we get $124x+24=4(31x+6)$.

  $~$

  $\mathbf{4(13x+5)}$

  Here we have to use the distributive property: we multiply each term in the sum by $4$ and combine the like terms:

  $4(13x+5)=(4)(13x)+(4)(5)=52x+20$.

  $~$

  $\mathbf{24x+12y-36}$

  The common factor here is $12$. Dividing each term by $12$ leads to

  • $24x\div 12=2x$
  • $12y\div 12=y$
  • $36\div 12=3$

  So we can conclude that $24x+12y-36=12(2x+y-3)$.

  $~$

  $\mathbf{24(x+2y-6)}$

  Again we use the distributive property

  $24(x+2y-6)=(24)(x)+(24)(2y)-(24)6$

  and combine the like terms

  $24(x+2y-6)=24x+48y-144$.