Combining Rational Number Like Terms

Basics on the topic Combining Rational Number Like Terms
When simplifying algebraic expressions, it’s necessary to combine all like terms together. Given a long list of objects in real life, summarizing the list is one big step in putting order to it. Just like in combining like terms, similar objects in the list can be put together for better organization. Learn how to combine like terms with rational numerical coefficients by helping Laura, an archaeologist, put back together pieces of broken artifacts that she found in a historical site in Cambodia. Common Core Reference: CCSS.MATH.CONTENT.7.EE.A.1
Transcript Combining Rational Number Like Terms
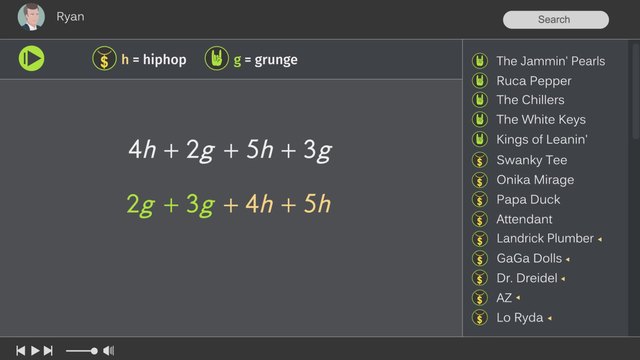
We're in Cambodia, located in Southeast Asia, to check in with our friend Laura. Laura’s an archaeologist and she's busy excavating a site that hasn't been touched in hundreds of years. While digging, Laura's discovered lots of interesting artifacts. Unfortunately they are all broken, so she has to put the pieces back together correctly. Just like putting together pieces of broken artifacts, combining rational number like terms works similarly by piecing parts of an expression together. Here's the first expression that we need to simplify: First, let's find the like terms and use the Commutative Property of Addition to reorder the expression writing the x’s together and the y’s together. Next, combine the like terms giving us 15x plus 2y. Let’s try another problem with rational numbers. Because the coefficients for this problem are fractions, we may have a few more steps but the strategy to simplify the expression is the same. First, use the Commutative Property of Addition to reorder the terms. Next, we want to combine the like terms but we can't add the coefficients of 'h' just yet. First, we'll have to find a common denominator and modify the expression. Nine works. Now, use the Multiplicative Identity Property of 1 to modify the expression so both coefficients have 9 as the denominator. To do this, we multiply three thirds by two thirds giving us six ninths. Now that both coefficients of 'h' have the same denominator we can combine the like terms and simplify the fractions.
Notice, the expression contains terms that are not multiplied with a variable.
These terms are called constants.
Constants are numbers that are multiplied by a variable raised to the zero power which is always equal to 1.
Even though the variable raised to the zero power is not written we know it's there.
In other words, a constant is simply a number by itself.
And just in time to try another problem.
To make the problem easier to solve first change the mixed number into an improper fraction.
Next, use the Distributive Property to get rid of the parentheses.
Now, reorder the terms into Standard Form using the Commutative Property.
If we want to combine like terms, we have to first find a common denominator for the constant terms.
In this case, it's 18.
Now, we can combine the like terms. The last step, as always, make sure all the fractions are written in lowest terms and that the expression is written in standard form. Looks like we're good to go! So, how's Laura doing putting together all of the broken pieces? How about that? She's put together enough pieces to host a tea party with her new pal.
Combining Rational Number Like Terms exercise
-
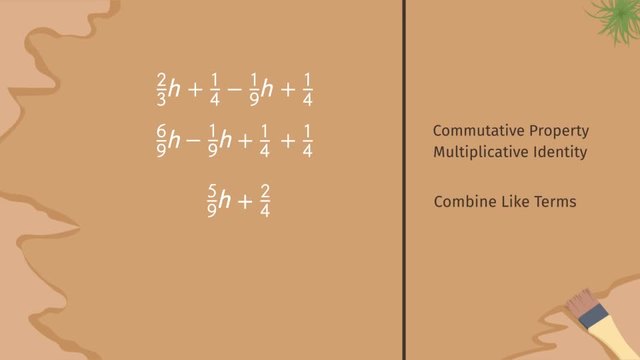
Describe how to combine like terms, and simplify the expression $\frac{2}{3}h+\frac{1}{4}-\frac{1}{9}h+\frac{1}{4}$.
HintsThe commutative property of addition states that $a+b=b+a$.
The commutative property of multiplication states that $a\times b=b\times a$.
Reducing a fraction means dividing the numerator as well as the denominator by the same number.
You can add terms such as $3h$ and $4h$ as follows: $3h+4h=(3+4)h=7h$.
SolutionTo simplify $\frac23h+\frac14-\frac19h+\frac14$, you first reorder the terms using the commutative property of addition:
$\frac23h-\frac19h+\frac14+\frac14$.
Next, combine the like terms. For this, you have to multiply $\frac23$ by $\frac33$. So both coefficients of $h$ have the same denominator:
$\frac33\cdot\frac23h-\frac19h+\frac14+\frac14=\frac69h-\frac19h+\frac14+\frac14$.
Now you are able to combine like terms:
$\frac69h-\frac19h+\frac14+\frac14=\frac59h+\frac24=\frac59h+\frac12$.
The second fraction $\frac24$ is reduced by dividing both the numerator and the denominator by $2$:
$\frac24=\frac{2\div2}{4\div 2}=\frac12$.
-
Explain the mathematical terms.
HintsThe commutative property for multiplication states that $a\times b=b\times a$.
Expanding a fraction means multiplying the numerator as well as the denominator by the same number. This doesn't change the value of the fraction, as what you are multiplying by is equal to $1$.
In this expression $3$ is the coefficient of $x^2$, $4$ is the coefficient of $x$, and $-4$ is a constant.
SolutionTo combine rational number like terms, we use some mathematical terms:
- A constant is a number that is multiplied by a variable raised to the zeroth power.
- A coefficient is a constant by which a variable is multiplied.
- We use the commutative property of addition, $a+b=b+a$, to change the order of terms.
- If we want to factor any term, like $a\times(b+c)$, we use the distributive property, which tells us that $a\times(b+c)=a\times b+a\times c$.
- The multiplicative identity property of $1$ is that multiplying by it doesn't change the value.
-
Give the steps for combing like terms.
HintsThe distributive property states that $a\times(b+c)=a\times b+a\times c$.
The commutative property of addition states that a+b=b+a.
For the standard form you reorder the terms from terms with the largest exponent to terms with the smallest exponent. For instance, $2x^2+2x-3y+4$ is in standard form.
SolutionFirst, we rewrite the mixed fraction:
$\frac{1}{3}+\frac{1}{2}\left(1\frac{1}{3}x-\frac{1}{4}\right)=\frac{1}{3}+\frac{1}{2}\left(\frac{4}{3}x-\frac{1}{4}\right)$.
Next, we use the distributive property to get rid of the parentheses:
$\frac{1}{3}+\frac{1}{2}\left(\frac{4}{3}x-\frac{1}{4}\right)=\frac{1}{3}+\frac{4}{6}x-\frac{1}{8}$.
Reordering the expression depending on the like terms leads to
$\frac{1}{3}+\frac{4}{6}x-\frac{1}{8}=\frac{1}{3}-\frac18+\frac{4}{6}x$.
So, now we are in the position to combine the like terms. For this we have to find the common denominator of the first two fractions, it's $24$, and expand the fraction as follows:
$\begin{array}{rcl} \frac{1}{3}-\frac18+\frac{4}{6}x&=&\frac{1\cdot 8}{3\cdot 8}-\frac{1\cdot 3}{8\cdot 3}+\frac{4}{6}x\\ &=&\frac{8}{24}-\frac{3}{24}+\frac{4}{6}x\\ &=&\frac{5}{24}+\frac{4}{6}x \end{array}$
Last, we can reduce the second fraction and rewrite the expression in standard form:
$\frac{5}{24}+\frac{4}{6}x=\frac{5}{24}+\frac23x=\frac23x+\frac{5}{24}$.
-
Calculate the total amount of ingredients by combining like terms.
HintsLet $e$ be the number of eggs, $f$ the number of cups of flour, and $s$ the number of cups of sugar.
For the muffins, Laura needs $2\frac13f+\frac12e$.
For the cookies, Laura needs $\frac12\left(1\frac13s+\frac34f\right)+\frac14e$.
Just add like terms and combine.
SolutionFirst, we establish both expressions describing the ingredients for the muffins as well as for the cookies:
- muffins: $2\frac13f+\frac12e$
- cookies: $\frac12\left(1\frac13s+\frac34f\right)+\frac14e$
We add both expressions together to get,
$2\frac13f+\frac12e+\frac12\left(1\frac13s+\frac34f\right)+\frac14e$.
Rewrite the mixed terms as fractions and use the distributive property:
$\frac73f+\frac12e+\frac12\left(\frac43s+\frac34f\right)+\frac14e=\frac73f+\frac12e+\frac46s+\frac38f+\frac14e$
Now we reorder the expression to get,
$\frac73f+\frac38f+\frac12e+\frac14e+\frac46s$
Almost done! We still have to find the common denominators to combine like terms:
$\begin{array}{rcl}\frac73f+\frac38f+\frac12e+\frac14e+\frac46s&=&\frac{7\cdot 8}{3\cdot 8}f+\frac{3\cdot 3}{8\cdot 3}f+\frac{1\cdot2}{2\cdot 2}e+\frac14e+\frac46s\\ &=&\frac{56}{24}f+\frac{9}{24}f+\frac{2}{4}e+\frac14e+\frac46s\\ &=&\frac{65}{24}f+\frac34e+\frac23s \end{array}$
So in total Laura needs:
- $\frac34$ eggs
- $\frac{65}{24}$ cups of flour
- $\frac23$ cups of sugar
-
State how to combine like terms with integers.
HintsThe commutative property of addition is given by $a+b=b+a$.
Just look at the following examples for like terms:
- $4x$ and $-3x$ are like terms.
- $4x$ and $4y$ aren't like terms at all.
- $3y$ and $-4y$ are like terms.
SolutionTo simplify this expression, we first find the like terms and reorder the expression using the commutative property.
So the $x$-terms as well as the $y$-terms are grouped together: $2x+13x-7y+9y$.
Now we can combine the like terms:
- $2x+13x=15x$
- $-7y+9y=2y$
-
Combine and simplify the given rational expressions as much as possible.
HintsUse the commutative property, $a+b=b+a$, as well as the distributive property, $a\times(b+c)=a\times b+a\times c$.
Here you see an example for changing mixed terms into fractions.
Solution- $\mathbf{\frac23\left(\frac12x-\frac14+x\right)}$
$\frac23\left(\frac12x-\frac14+x\right)=\frac26x-\frac2{12}+\frac23x$
We can now reduce the fractions and reorder the expression to get
$\frac13x+\frac23x-\frac16=x-\frac16$
$~$
- $\mathbf{\frac45x-\frac13y+\frac23y-y+\frac15x}$
$\frac45x+\frac15x-\frac13y+\frac23y-y$
Now we can combine the like terms to get
$\frac55x+\frac13y-y=x+\frac13y-\frac33y=x-\frac23y$
$~$
- $\mathbf{3\left(\frac25y-\frac23x+2x\right)-\frac15y}$
$\frac65y-\frac63x+6x-\frac15y$
Now we reduce the fractions if possible, reorder the expression, and combine the like terms:
$-2x+6x+\frac65y-\frac15y=4x+\frac55y=4x-y$
$~$
- $\mathbf{\frac38y+\frac25x+\frac56y-\frac4{15}x}$
$\begin{array}{rcl} \frac38y+\frac25x+\frac56y-\frac4{15}x&=&\frac25x-\frac4{15}x+\frac38y+\frac56y\\ &=&\frac6{15}x-\frac4{15}x+\frac9{24}y+\frac{20}{24}y\\ &=&\frac2{15}x+\frac{29}{24}y \end{array}$
$~$
- $\mathbf{2\frac12\left(\frac15x-\frac35y+\frac32x+y\right)}$
$\frac15x-\frac35y+\frac32x+y=\frac2{10}x+\frac{15}{10}x-\frac35y+\frac55y=\frac{17}{10}x+\frac25y$
The mixed number $2\frac12$ can be written as a fraction, $\frac52$. Last we use the distributive property:
$\frac52\left(\frac{17}{10}x+\frac25y\right)=\frac{85}{20}x+\frac{10}{10}y=\frac{17}4x+y$
$~$
- $\mathbf{3\frac13x+2\frac14y-2\frac12x-1\frac15y}$
$\frac{10}3x+\frac94y-\frac52x-\frac65y$
Now we reorder the expression to
$\frac{10}3x-\frac52x+\frac94y-\frac65y$
Next we expand each fraction to get the common denominator:
$\frac{20}{6}x-\frac{15}6x+\frac{45}{20}y-\frac{24}{20}y=\frac56x+\frac{21}{20}y$