Writing and Evaluating Expressions with Exponents

Writing and Evaluating Expressions with Exponents exercise

• Show how to write the given situation as an exonential expression.

  Hints

  Each of the three friends recommends the video to another three friends.

  Each indicates multiplication.

  $a^n$ is a shorter way to write a multiplication: $a^n=a\times a\times ...\times a$, or $a$ multiplied by itself $n$ times.

  If Leelee wants to know how many people are viewing her video in the fifth minute, she calculates $3^5=243$.

  Solution

  She plans to send it to three friends, and after a minute she wants each of those friends to send it to another three friends each, and so on. Let's see what Leelee´s results should be:

  • During the first minute, three people will view the video.
  • During the second minute, $3\times 3$ people will view the video, which simplifies to $9$ people total.
  • During the third minute, $3\times 3\times 3$ people will view the video, which simplifies to $27$ people total.
  • During the fourth minute, $3\times 3\times 3\times 3$ people will view the video, which simplifies to $81$ people total.
  • We can generalize this by saying that during the $x^{th}$ minute, $3^x$ people will view the video.

• Express the following problem as a mathematical expression.

  Hints

  We have that $5^3=5\cdot 5\cdot 5\cdot 5$, while $3^5=3\cdot 3\cdot 3\cdot 3\cdot 3$.

  Five friends who should send it to five friends each gives us $5\times 5=5^2=25$ people viewing the video.

  You finally have to multiply $25$ by $5$ once again: $25\times 5=125$.

  Check this result with each of the given alternatives.

  Solution

  First Leelee sends the videos to five friends. Then all of those friends send the video to five friends each. This leads to $5\times 5=5^2=25$.

  Then, all of these $25$ people send the video to five friends each. So we finally get $5\times 5\times 5=5^3=125$ people viewing the video in the end.

• Explain how to transform the word problems.

  Hints

  Here you see how a video is shared after $1$, $2$, $3$, and $4$ minutes, when it is shared with three people in the first minute, and those three people share it with three more people in the second minute, and so on.

  Keep the notation for exponents in mind:

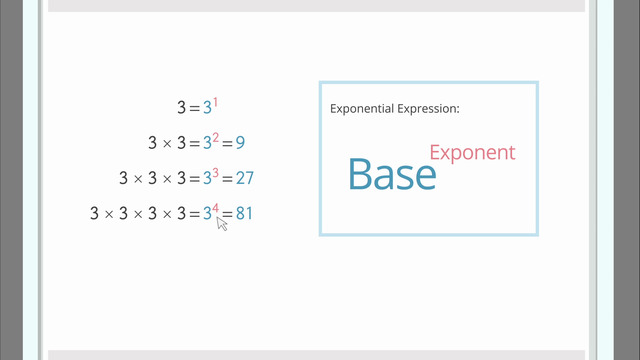
  • $3=3^1$
  • $3\times 3=3^2$
  • $3\times 3\times 3=3^3$
  • $3\times 3\times 3\times3=3^4$

  So after $3$ minutes the video is shared with $3^3=27$ people.

  Solution

  In each of the given examples you have to find the base as well as the exponent of a power:

  Ladybugs

  The video is shared with $4$ people. All of those share it with $4$ people each after one minute and so on. So after 5 minutes, we get $4\times 4\times 4...\times 4=4^5=1024$.

  Butterflies

  Leelee shares the video with $10$ people. All of those share it again with $10$ people each after one minute. And so on. Thus, after 4 minutes, we get $10\times 10\times 10...\times 10=10^4=10000$.

  Grasshoppers

  This time Leelee sends the video to $7$ people and asks them to send it out to $7$ people each after one minute. Then the video will be sent to $10$ people and so on. So after 6 minutes, we have $7\times 7\times 7...\times 7=7^6=117649$.

• Find the right exponential expression.

  Hints

  The base of $a^n$ is $a$.

  The exponent of $a^n$ is $n$.

  For example, $4\times 4\times 4=4^3$.

  Solution

  New Years Messages

  She sends the messages to $6$ friends. After one hour each of those friends sends a message to another $6$ friends. So it's $6\times 6$. After another hour all of those $6\times 6$ friends send a message to $6$ friends each ... So we get $6^4$ messages sent after three hours.

  Bacterium

  A bacterium doubles after one period to $2=2^1$ bacteria. After another period there are $2^2$ bacteria. So we can see that $2^n$ there are bacteria after $n$ periods.

  Giraffe Legs

  First determine the number of giraffes: $4$ giraffes in $4$ zoo exhibits leads to $4\times 4$ giraffes. Each of the giraffes has $4$ legs. So, in total we can count $4\times 4\times 4=4^3$ legs.

  Mateo's Flyer

  To promote his new taco bar, Mateo distributes flyers: first to $5$ people. All of those people distribute flyers to $5$ people each. After $4$ distributions $5^4$ flyers are given away.

• Label the base as well as the exponent.

  Hints

  The exponent is the number of times you multiply the base by itself.

  In general a power is given by $a^n$, where $a$ stands is the base of the power.

  Keep the meaning of the corresponding positions in mind.

  In the example beside $7$ is the base while $5$ is the exponent.

  Solution

  In general a power is given by $a^n$, where $a$ is called the base and $n$ is called the exponent.

  You can read it as $a$ raised to the power of $n$.

• Determine the corresponding expression.

  Hints

  The volume of any rectangular prism is given by

  width $\times$ length $\times $ height.

  You can simplify as follows:

  $(3x)\times(2x)\times(4x)=(3)(2)(4)(x^3)=24x^3$.

  Just multiply the coefficients.

  The volume of a cube with the length $4$ is given by $64$.

  Solution

  The volume of a cube can be determined by raising the side to the power of $3$. This leads to $a^3$.

  The volume of a rectangular prism is given by width $\times$ length $\times $ height. Thus, we get $(3h)(4h)(h)=(3)(4)h^3=12h^3$.

  The area of a square is given by the length squared: $s^2$.