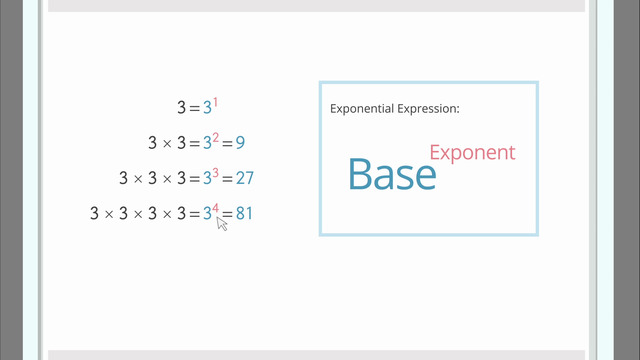
Writing and Evaluating Expressions with Multiplication and Division

Writing and Evaluating Expressions with Multiplication and Division exercise

• Walk through the steps of picking a variable, setting up an expression, and completing a table.

  Hints

  Keep keywords for multiplication in mind:

  • times
  • each
  • per
  • twice
  • doubled
  • tripled

  So twice indicates multiplication.

  Let's have a look at an example: Paul has double as much money as Anne. Anne has $4$ dollars. So, Paul has $8$ dollars.

  Solution

  Let's start with the unknown height of the Jones' tree. Usually we assign a variable to an unknown value. Often the letter $x$ is used as a variable. You can also use any letter; let's use the variable $h$ for the unknown height.

  Next, we transform the word problem into an algebraic expression. The twice indicates multiplication. We have to multiply $h$ by $2$. This leads to $2h$ as the height of the Smiths' tree.

  Last we can complete the table by multiplying each given height by $2$.

• Complete the table using the correct expression.

  Hints

  Let's have a look at the following example: the total number of candles is $40$. So the number of candles per window is $8$.

  The following are some keywords for division:

  • quotient
  • split
  • per
  • every
  • divide
  • ratio
  • each

  evenly divide indicates division.

  Solution

  First, we figure out the operation we need to use: it's division.

  The unknown number of candles, $c$, should be divided evenly between the $5$ windows. So you have to divide $c$ by $5$: $\frac c5$.

  With this algebraic expression you can determine the number of candles per window, given the number of candles:

  • $c=5$ $\rightarrow$ $\frac55=1$
  • $c=10$ $\rightarrow$ $\frac{10}5=2$
  • $c=15$ $\rightarrow$ $\frac{15}5=3$
  • $c=20$ $\rightarrow$ $\frac{20}5=4$

• Complete the table to find the expression.

  Hints

  You get a voucher of $25$ cents per bottle returned.

  per indicates multiplication.

  $25$ cents is a quarter of a euro; $25$ cents $=$ $0.25$ euros.

  If you want to know the number of returned bottles, divide the total sum by $0.25$.

  Let's have a look at an example.

  If you get $50$ euro back, then you've returned

  $\frac{50\text{ euros}}{0.25\text{ euros}}=200$

  bottles.

  Solution

  Let $b$ be the number of returned bottles. So $0.25b$ determines the value of the voucher.

  The other way round: for a given voucher, $v$,

  $\frac{v}{0.25}=4v$

  gives you the corresponding number of bottles.

  You can see all the solutions beside.

  Let's have a look at a view values:

  • $b=10$ leads to $(10)(0.25)=2.50$
  • $b=325$ leads to $(325)(0.25)=81.25$

  The other way round: $v=100$ leads to

  $\frac{100}{0.25}=(4)(100)=400$.

  This is the desired number in the last row.

• Determine the wanted values.

  Hints

  The answer depends on the desired information:

  What is given? The number of lawns or the amount of money earned?

  Use division to solve the task given a certain amount of earned money.

  For example, if Leo mows $12$ lawns, then he earns $180$ dollars. If he would like to earn $105$ dollars, then he should mow $7$ lawns.

  Solution

  Beside you see the whole solution.

  Let's start with the algebraic expression.

  Leo gets $15$ dollars for each lawn. Let $l$ be the variable for the number of lawns he mows. So we have to multiply: $15~l$.

  With this information you get amount of money Leo earns, depending on the number of lawns he mows:

  • $l=3$ leads to $(15)(3)=45$ dollars.
  • $l=6$ leads to $(15)(6)=90$ dollars.

  The other way round, you have to divide to get the number of lawns, given a certain amount of money earned. For example, $195$ dollars:

  $\begin{array}{rcl} 15\times l & = & ~195\\ \color{#669900}{\div 15} & &\color{#669900}{\div 15}\\ l & = & ~13 \end{array}$

  So Leo has to mow $13$ lawns to earn $195$ dollars.

• Evaluate the expression $4l+200$ for the given $l$ values.

  Hints

  If you have to evaluate a given algebraic expression, just plug the value in for the variable.

  Here you see an example with the algebraic expression $2h$ and $h=20$.

  You see $h$ is replaced by $20$.

  Solution

  If you'd like to evaluate this algebraic expression for different values for $l$, just replace $l$ by the values:

  • $l=2$ leads to $(4)(2)+200=8+200=208$
  • $l=5$ leads to $(4)(5)+200=20+200=220$
  • $l=10$ leads to $(4)(10)+200=40+200=240$
  • $l=15$ leads to $(4)(15)+200=60+200=260$

• Express the word problem as an expression and evaluate.

  Hints

  Decide which operation to use first.

  For this, keep keywords for multiplication or division in mind.

  You can check each result. For example, if Paul buys tacos for $250$ dollars, at $5$ dollars each, he has to divide, $\frac{250}5=50$, to get the number of tacos.

  The other way round, Paul multiplies to see how much money he will spend in total: $50\times 5=250$ dollars.

  Solution

  If Zoe earns $m$ per year, you can calculate the monthly income by dividing by $12$: $\frac m{12}$.

  For a given income of $36,000$ dollars per year, we get $\frac{36000}{12}=3000$ dollars.

  The total amount of concert tickets sold is $m$. If each ticket costs $75$ dollars, how can we determine the number of sold tickets? It is $\frac m{75}$.

  Let $m= 93,750$. To get the number of sold tickets, we have to divide $m$ by $75$: $\frac {93,750}{75}=1250$. This is the desired number of sold tickets.