What is Factoring?

What is Factoring? exercise

• Recall the steps for factoring an expression.

  Hints

  Pay attention to the paranthesis.

  You can check the factorization. Let's have a look at the following example: $6+8x=2(3+4x)$.

  You can use the distributive property to check your answer: $2(3+4x)=(2)(3)+(2)(4x)=6+8x$. ✓

  To get the greatest common factor, just multiply all common factors.

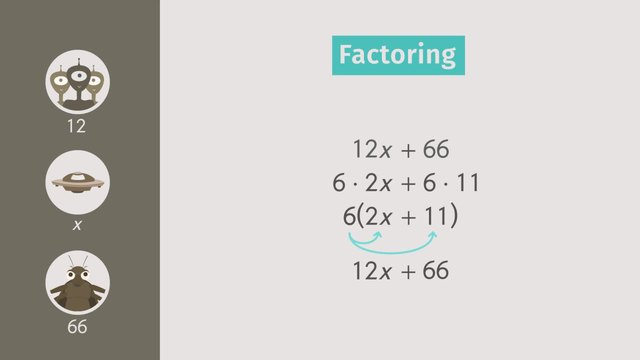
  Solution

  The is the expression for the total of aliens is $12x+66$.

  To decide which sports they could do, he must divide the aliens into groups of equal size.

  First, Ralph has to look for the greatest common factor of $12x$ and $66$:

  • $12x=(2)(2)(3)(x)$
  • $66=(2)(3)(11)$

  Just look at both terms. Which factors do they have in common? They have $2$ as well as $3$. So the product $(2)(3)=6$ is the greatest common factor.

  So we can factor $6$ out of the expression to get $6(2x+11)$.

  You can check this factorization using the distributive property:

  $6(2x+11)=(6)(2x)+(6)(11)=12x+66$.

  That's it.

• Factor the expressions.

  Hints

  Keep the commutative property in mind. The order of multiplication can be changed:

  $a\times b=b\times a$.

  The input is clear depending on the parentheses and the factor $x$.

  Just look at this example.

  The greatest common factor of $12x$ and $8$ is $4$.

  You can check your factorization using the distributive property.

  Solution

  Here you see the solution. The expression $6x+15$ should be factored out.

  First, we factor both terms:

  • $6x=2\times 3\times x$ and
  • $15=3\times 5$

  Each term has just the factor $3$ in common. Thus it's the greatest common factor:

  $6x+15=3\times 2x+3\times 5$.

  Now we can factor out $3$ to get $3(2x+5)$.

  You can check this factorization using the distributive property.

  $3(2x+5)=(3)(2x)+(3)(5)=6x+15$ ✓

• Identify the expressions that are factored completely.

  Hints

  Check each factorization by using the distributive property.

  Just look at the example beside.

  Make sure to factor out the greatest common factor.

  Sure $8x-12y=2(4x-6y)$ and $2$ is a common factor of $8x$ and $12y$. But it isn't the greatest common factor.

  Solution

  Poor Ralph. He's a little bit stressed. He has to factor out a lot of expressions and he should be absolutely concentrated... but he keeps getting distracted.

  Let's help him out by helping him to find his mistakes.

  First, let's look at $\mathbf{24x+16}$. We have that

  • $24x=2\times 2\times 2\times 3\times x$
  • $16=2\times 2\times 2\times 2$

  The factors in common are three times $2$. Thus, the greatest common factor is $2\times 2\times 2=8$.

  $24x+16=8\times 3x+8\times 2=8(3x+2)$.

  Just have a look at another example: $\mathbf{8x^2+24x}$. We have that

  • $8x^2=2\times 2\times 2\times x\times x$
  • $24x=2\times 2\times 2\times 3\times x$

  Here we have a greatest common factor including $x$, namely $2\times 2\times 2\times x=8x$.

  $8x^2+24x=(8x)(x)+(8x)(3)=8x(x+3)$.

  To get a better feeling in your factoring skills, just check the calculations using the distributive property.

• Construct and factor the corresponding expressions.

  Hints

  Assign the variable $x$ to the unknown values.

  Let's have a look at an example. If Lisa buys $4$ muffins with an unknown price $x$ and coffee for $4$ dollars, we get the expression $4x+4$.

  The greatest common factor of $4x$ and $4$ is $4$ itself:

  $4x+4=(4)(x)+(4)(1)=4(x+1)$.

  Solution

  Here we first have to establish the algebraic expression. After that, we factor out the greatest common factor.

  $~$

  Paul

  He buys three pairs of shoes for an unknown price, $x$. This leads to $3x$. The price of the headphones must be added. The resulting expression is $3x+48$. The greatest common factor is $3$:

  $3x+48=(3)(x)+(3)(16)=3(x+16)$.

  $~$

  Monica

  She spends a lot of time doing her homework, six hours per assignment. The corresponding expression is $6x$. Next we add the total time for sports and get $6x+12$. Here we have the greatest common factor $6$, which we can factor out:

  $6x+12=(6)(x)+(6)(2)=6(x+2)$.

  $~$

  Matteo

  Each taco costs $4$ dollars. Because the number of sold tacos is unknown, we assign the variable $x$ to it. The fix delivery cost is added to get $4x+200$. The greatest common factor is $4$:

  $4x+200=(4)(x)+(4)(50)=4(x+50)$.

• Identify the GCF between the pairs of numbers.

  Hints

  You find the greatest common factor by factoring both numbers.

  Here is the factorization of $24$.

  Factor both numbers and then check for common factors:

  • $24=\mathbf{2}\times \mathbf{2}\times \mathbf{2}\times 3$
  • $16=\mathbf{2}\times \mathbf{2}\times \mathbf{2}\times 2$

  The factors in common are highlighted.

  The greatest common factor is the product of the highlighted factors:

  $2\times 2\times 2=8$.

  Solution

  To find the greatest common factor, you factor the given terms and check for common factors among the terms. If the terms have more than one factor in common, the greatest common factor is the product of those factors.

  Let's have a look at a few examples:

  $12$ and $8$:

  • $12=2\times 2\times 3$
  • $8=2\times 2\times 2$

  Both numbers have two times the factor $2$ in common. Thus the greatest common factor is $2\times 2=4$.

  $12$ and $9$:

  • $12=2\times 2\times 3$
  • $9=3\times 3$

  The only factor in common is $3$. So, it's the greatest common factor.

  $30$ and $24$:

  • $30=2\times 3\times 5$
  • $24=2\times 2\times 2\times 3$

  Both numbers have the factor $2$ and $3$ in common. Thus the greatest common factor is $2\times 3=6$.

  $25$ and $10$:

  • $25=5\times 5$
  • $10=2\times 5$

  The only factor in common is $5$ and so, it's the greatest common factor.

• Decide how to factor the expressions.

  Hints

  Determine the greatest common factor for the term of each expression.

  Let's have a look at an example: $15x^2-10x+5x^3=(5x)(3x)-(5x)(2)+(5x)(x^2)$.

  The greatest common factor is $5x$.

  If $5x$ is the GCF of $15x^2-10x+5x^3$, then we can factor it out to get $(5x)(3x)-(5x)(2)+(5x)(x^2)=5x(3x-2+x^2)$.

  Solution

  For each example, let's first look for the greatest common factor for all terms in the expression. Then we factor out the greatest common factor:

  $12x+6y+36=(6)(2x)+(6)(y)+(6)(6)=6(2x+y+6)$

  $12x^2+6x-36x^3=(6x)(2x)+(6x)(1)-(6x)(6x^2)=6x(2x+1-6x^2)$

  $24y-12x^2+18=(6)(3y)-(6)(2x^2)+(6)(3)=6(3y-2x^2+3)$