Keywords for Division


Basics on the topic Keywords for Division
Introduction to the Video Keywords or Division
Instant recognition of specific keywords is a sure technique to easily proceed with the division process. Knowing the specific parts, like the dividend, divisor, quotient, and remainder, will avoid misplacement of the given and ensure a correct computation. Other words that imply division, like ratio and average, can be additional clues when easily identified in a problem requiring division as part of the solution. See how shepherd McWIlliams taught his sheep dog McWoof to learn these keywords and to help him take care of his herd.
Common Core Reference
CCSS.MATH.CONTENT.6.EE.A.2
Keywords for Division Exercise
Start the following exercises regarding the topic Keywords for Division and test your knowledge now!
Transcript Keywords for Division
Meet McWoof, sheep herding dog extraordinaire. Although he's an expert now, he wasn't always able to divide herds of sheep into smaller groups.
The keywords for division
To get where he is today, McWoof had to learn all the keywords for division. Since McWoof was a puppy, Shepherd McWilliams taught him different signals for the parts of division:
- the dividend
- the divisor
- the quotient and the
- remainder
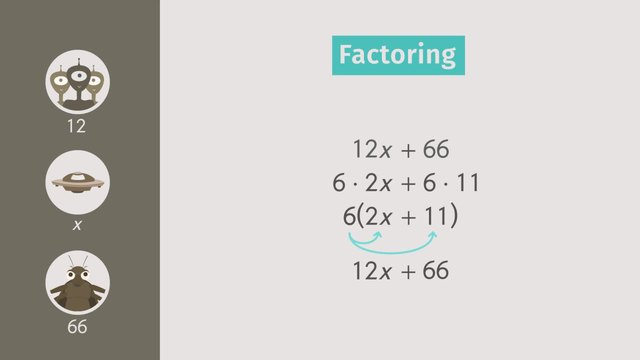
To stop overgrazing, Shepherd McWilliams’ sheep have to graze on different fields. So, we need these 18 sheep to be divided into equal groups of 6 sheep each. In this situation, 18 is the dividend, or the number you are going to divide. 6 is the divisor, or the number of sheep in one group. From 18, we can make three equal groups of 6 sheep, so the quotient is 3. If there were any sheep left over after dividing, that would be the remainder. We could write this equation like this, this, or this.
No matter how you write it, the answer, or the quotient, is the same. When he’s finished, McWoof gives the shepherd a signal that the job is done and he can check to see if the groups are of equivalent sizes. He was taught that it's important to make sure all the groups are the same size, with no remainders. It looks like McWoof tried to divide the 18 sheep into equal groups with 5 sheep in each group. But after making 3 groups, each with 5 sheep, there are 3 sheep remaining; so the remainder is 3.
The ratio
In addition to splitting the herd into equal groups, McWoof was also taught to keep track of the ratio of white sheep to black sheep in the herd. In this case, we have a ratio of 15 white sheep to 3 black sheep. Typically, we reduce the ratios to the simplest form, just like with fractions! This means that for every five white sheep, there is one black sheep. Ratios can be written in a couple of different ways.
McWilliams also taught McWoof to help determine the average amount of wool that comes from his different kinds of sheep. To find the average of something, you take a total sum and divide that by the number of elements you added together. For example, if the black sheep’s wool production looks like this, then the total sum of the pounds of wool, 9, is your dividend, and the number of things you summed, in this case, the number of black sheep, 3, is your divisor. Now we just divide 9 by 3 to find the average. So each time one of the black sheep is sheared, Shepherd McWilliams can expect an average of 3 lbs. of wool. Shepherd McWilliams is so proud of his herding hound, that he’d like to give him one of his favorite treats. Oh, I guess counting sheep has unintended consequences.
Keywords for Division exercise
-
Describe different ways to represent division.
HintsCheck the result by inverting division.
$?\cdot 6=18$
Keep in mind:
dividend $\div$ divisor $=$ quotient.
You have different ways to write down a division, but it doesn't make sense to get different results.
SolutionThe dog has to divide the flock of $18$ sheep into groups of $6$.
This is a division task: $18\div 6$.
- $18$ is the dividend, the number you are going to divide.
- $6$ is the divisor, the number of sheep in each group.
- The result, the number of groups of sheep, is the quotient. Here it is $3$.
- divisor)$\overline{\text{dividend}}$ and for the example above $6)\overline{18}$
- dividend $\div$ divisor - this leads to $18\div 6$
- $\frac{\text{dividend}}{\text{divisor}}$ - or for the example above $\frac{18}6$
-
Explain ratios.
HintsKeep in mind that we are looking for the ratio of white sheep to black sheep. Not the other way round.
You can reduce the fraction as follows:
$\frac{15}3=\frac51=5$.
But a ratio needs two arguments.
SolutionYou can also describe ratios using division notation.
If you look at the example of $18$ sheep with...
- ...$15$ white sheep and
- ...$3$ black sheep
So $15:3$ is the ratio of white sheep $:$ black sheep.
You can simplify the expression to $5:1$. This means that for each group of $5$ white sheep, there is just $1$ black sheep in the group.
You can also write a ratio...
- ...as a fraction $\frac51$, or
- ...as $5$ to $1$.
-
Describe how to determine the average.
HintsLet's have a look at another average calculation, for George and Ringo:
- First, we add the given pounds to get $4+6=10$.
- Next, we divide this sum by the number of sheep, i.e. $2$.
The sum of all calculated averages above is $11$.
Two of the averages are the same.
SolutionTo determine the average of different values you proceed as follows:
- Add all the given values.
- Divide the resulting sum by the number of values.
- $4+2=6$
- $6\div 2=3$
Next we consider John, Paul, and Ringo:
- $2+4+6=12$
- $12\div3=4$
Lastly, let's have a look at all the sheep:
- $4+2+4+6=16$
- $16\div4=4$
-
Highlight the key words concerning division problems.
HintsRemember, dividend $\div$ divisor $=$ quotient.
Here you see an example with a remainder:
$25\div 3=7$ remainder $4$.
Let's check it: $7\times 3=21$. So you get the following:
$7\times 3+4=21+4=25$.
SolutionRemember, dividend $\div$ divisor $=$ quotient:
Dividing stands for...
- ...splitting into several parts
- ...sharing anything
- ...cutting into equal pieces
Paul shares his cherry sweets leads to $25\div 5=5$:
- The number of sweets, $25$, is the dividend.
- The number of persons, $5$, is the divisor.
- The resulting number of sweets for each person, $5$, is the quotient.
- $12$ is the dividend.
- $3$ is the divisor.
- $12\div 3=4$ is the quotient.
The average age of Claire, Sue, and Jenny can be calculated as follows:
- $17+21+34=72$. This is the dividend.
- Divide the dividend by $3$, the number of people. This is the divisor.
- The average age is the quotient $72\div 3=24$.
This leads to $100\div 6=16$ remainder $4$ dollars per person.
-
Represent dividend, divisor, and quotient.
HintsFor addition, you have: (summand) $+$ (summand) $=$ (sum).
For subtraction, you have: (minuend) $-$ (subtrahend) $=$ (difference).
For multiplication, you have: (factor) $\times$ (factor) $=$ (product).
The divisor is that which you divide the dividend.
The quotient is the result of a division.
SolutionThe dog has to divide the herd into groups of $6$. $18$ sheep belong to the herd. So you get the following division task: $18\div 6$.
- $18$ is the dividend, the number you are going to divide.
- $6$ is the divisor, the number of groups you are going to make.
- The result, the number of groups of $6$ sheep made, is the quotient. Here it is $3$.
-
Apply division, ratio, and average problems.
HintsSimplify ratios or fractions if it's possible.
Let's have a look at the following example:
$\frac{20}{8}=\frac 52$.
To get the average of several values proceed as follows:
- Add the values.
- Divide the resulting sum by the number of values.
For the desired ratio, determine the number of female students first.
SolutionLet's start with the ratio male students to female students:
- There are $30$ students in total, $18$ of them male.
- Thus $30-18=12$ are female students.
You can interpret this result as follows: for each $3$ male students there are $2$ female students.
To practice division the school class will be divided into four groups. $30$ isn't divisible by $4$. Thus we must get a remainder. We have to divide $30$ by $4$ as follows:
$30\div 4=7$ remainder $2$.
Perhaps those $2$ students can be the referees for the following quiz. We have to determine the average:
- Add all given values: $120+80+70=270$.
- Divide this sum by the number of values $270\div 3=90$.