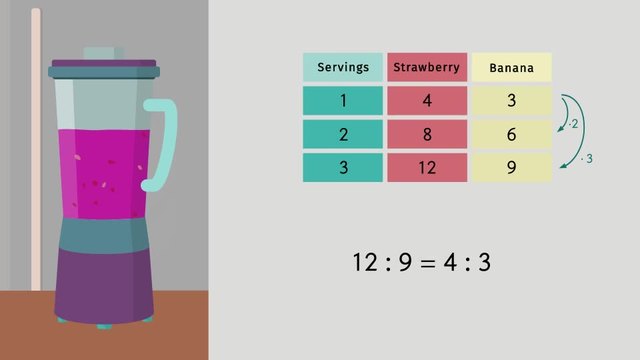
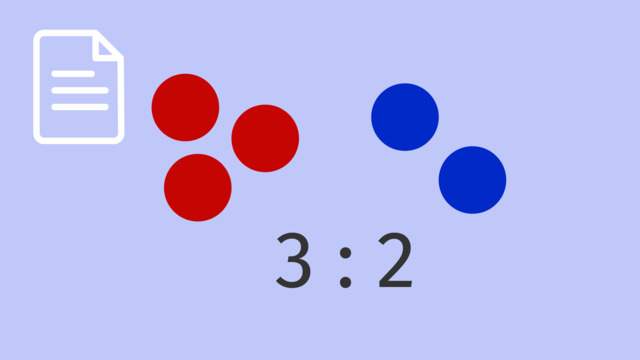
What is a Ratio? (Using Ratio Language)
Learning text on the topic What is a Ratio? (Using Ratio Language)
Understanding Ratios
Ratios are a fundamental part of mathematics and are used in many different areas, including cooking, map reading, and even in your favorite video games. If you're curious about how ratios are everywhere in our lives, you're in the right place! Let's explore what ratios are, the terminology used with them, and how we can understand them better. Get ready to dive into the world of ratios!
What is a Ratio?
A ratio is a way to compare two quantities by showing how many times one value contains the other. It tells us about the relative size of two quantities. Think of it like a recipe - if a cake recipe calls for 2 cups of flour and 1 cup of sugar, we can say the ratio of flour to sugar is 2:1.
Key Terms in Ratios
Here are some important terms you'll come across when learning about ratios:
| Term | Definition |
|---|---|
| Antecedent | The first number in a ratio |
| Consequent | The second number in a ratio |
| Equivalent Ratios | Different ratios that express the same relationship |
| Proportion | An equation stating that two ratios are equal |
Examples of Ratios
If a bag has 6 red marbles and 4 blue marbles, the ratio of red to blue marbles is 6:4.
In a classroom with 10 girls and 15 boys, the ratio of girls to boys is 10:15.
Ratio Language – Different Expressions
Ratios can be expressed in different ways:
- As a fraction: $\frac{1}{2}$
- Using a colon: 1:2
- With the word 'to': 1 to 2
All these expressions mean the same thing - for every 1 of the first quantity, there are 2 of the second quantity.
Ratio– Application
Answer these questions to test your understanding of ratios and the terms used in ratios.
What is a Ratio?– Summary
Key Learnings from this Text:
A ratio is a comparison of two quantities.
Ratios can be written in different forms but mean the same thing.
Understanding the terms like antecedent, consequent, equivalent ratios, and proportion is crucial.
Ratios are used in everyday life in various ways.
Keep practicing with ratios in different scenarios to strengthen your understanding. Make sure you are using the ratio terminology that you have learned today. If you are ready to do some ratio-related math, apply it to calculating lengths. And remember, ratios are not just numbers; they are a way to understand the world around us!
##What is a Ratio?– Frequently Asked Questions
