Unit Rates with Fractions
Basics on the topic Unit Rates with Fractions
Unit Rates with Fractions – Introduction
Unit rates are a fundamental concept in mathematics that help us understand the efficiency or cost-effectiveness of various situations. When combined with fractions, this concept becomes an essential tool for making informed decisions in daily life. Whether comparing prices or calculating speed, the ability to compute unit rates that involve fractions is a practical skill to have.
Understanding Unit Rates – Definition
A unit rate is a ratio that compares a quantity to one unit of another measure. It often answers the question, "How much of one item is there per one unit of another?" A unit rate with fractions involves ratios where one or both of the quantities are fractions.
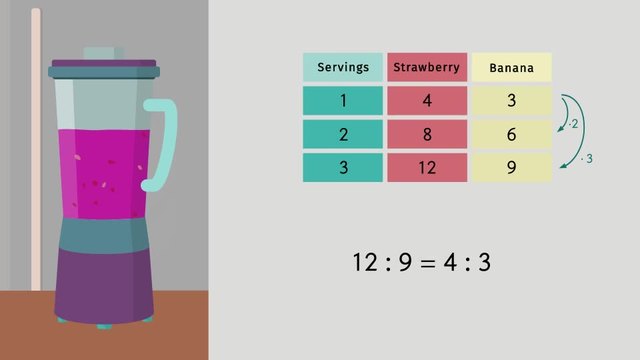
For instance, if a car travels $\frac{3}{4}$ of a mile in $\frac{1}{4}$ of an hour, the unit rate would be the distance per hour, which can be calculated by dividing the fraction representing the distance by the time fraction.
Unit Rate with Fractions – Example
Calculate the speed of a car that travels $\frac{3}{4}$ of a mile in $\frac{1}{4}$ of an hour.
| Step | Description | Mathematical Expression |
|---|---|---|
| Identify the quantities | Distance traveled is $\frac{3}{4}$ mile, and time taken is $\frac{1}{4}$ hour. | - |
| Find the unit rate | Speed = Distance/Time | - |
| Manipulate the fractions | Divide the distance by the time to find the speed. | $\left(\frac{3}{4}\right) \div \left(\frac{1}{4}\right)$ |
| Solve | Multiply by the reciprocal of the time fraction. | $\left(\frac{3}{4}\right) \times \left(\frac{4}{1}\right) = 3$ |
The car's speed is 3 miles per hour.
Let's find the unit price of bananas if $\frac{3}{5}$ of a pound costs $\$$1.20.
| Step | Description | Mathematical Expression |
|---|---|---|
| Identify the quantities | Quantity of bananas is $\frac{3}{5}$ pound, and cost is $\$$1.20. | - |
| Find the unit price | Price = Cost / Quantity | - |
| Convert to the unit rate | Divide the cost by the quantity to find the unit price. | $\$ 1.20\div \dfrac{3}{5}$ |
| Solve | Multiply by the reciprocal of the quantity fraction. | $\$1.20\times \dfrac{5}{3} = \$2.00$ |
The unit price for bananas is $\$$2.00 per pound.
Unit Rate with Fractions – Summary
Key Learnings from this Text:
Understanding unit rates involving fractions is important for real-world problem-solving.
To calculate a unit rate with fractions, divide the given quantity by the unit measurement.
Comparing unit rates with fractions helps in making better choices in shopping and time management.
Practice is essential to mastering the calculation of unit rates with fractions.
Explore other content on our platform, including interactive practice problems and videos, to support your educational journey in understanding unit rates and fractions.
Unit Rate with Fractions – Frequently Asked Questions
Transcript Unit Rates with Fractions
Kai and Luis are interviewing for a pizza delivery job at Mario’s Pizza Palace. The person that can deliver at the fastest rate will be given the position, so let's compare the, Unit Rates with Fractions. To compare the delivery speeds, you need to find the unit rate this shows the quantity of a unit per one quantity of another unit. To find the unit rate, you set up a ratio and then divide the numerator by the denominator. It looks like the first orders are in, so Kai will get ready for the first delivery. Kai covers one-half of a mile in one-fourth of an hour, delivering pizzas to hungry customers. To find the unit rate for Kai’s delivery speed, you need to first set up a ratio, and then divide the miles by the hours. It's called a complex fraction when there is a fraction in the numerator or the denominator, or both. A complex fraction can simply be rewritten horizontally, to show that this is a division problem. Let's calculate Kai’s unit rate by dividing one-half by one-fourth, which will tell you what their speed is in miles per hour. Remember, when dividing fractions we multiply the first fraction by the reciprocal of the second fraction. Now multiply the numerators and denominators straight across, which equals four halves. Four halves simplifies to two. Kai delivered pizzas at a rate of two miles per hour. Now it's Luis's turn to deliver! Luis delivers the ordered pizzas flawlessly and covers one-third of a mile in one-sixth of an hour. Again, to find the unit rate, you divide the miles by the hours. You rewrite it like this and then change it to a multiplication problem. One-third multiplied by six is six-thirds which will simplify to two. Kai and Luis both had the same delivery speed of two miles per hour, resulting in a tie! Time for a rematch! Kai is handed the next set of orders, determined to beat their earlier delivery speed. Kai covers one mile in one-fourth of an hour. How do we find the unit rate? We divide one by one-fourth, and rewrite it as one multiplied by four. What is the unit rate? Four miles per hour, which is a new record for Kai! Luis’ delivery route has a whopping four miles on it, and he completes the order in one-fifth of an hour. Pause here and to calculate Luis' delivery speed to see if he, by some miracle, beat Kai’s record. Four divided by one-fifth is rewritten as four multiplied by five, which is twenty. Luis made his deliveries in an astonishing twenty miles per hour! Before we see who gets the job, let's summarize! Find the unit rate by changing the division of fractions to multiplying by the reciprocal. Finding the unit rate is useful when comparing different rates, such as miles and hours. Luis sure knows the meaning of working smarter, not harder.