What are Supplementary and Complementary Angles?
- Supplementary and Complementary Angles – Introduction
- Complementary Angles
- Identifying Complementary Angles
- Finding a Complementary Angle

Basics on the topic What are Supplementary and Complementary Angles?
Supplementary and Complementary Angles – Introduction
In geometry, understanding angle relationships is essential, especially when we talk about supplementary and complementary angles. These concepts are key tools for solving puzzles about missing angles without even using a protractor. Mastering these angles helps you tackle various geometric problems more efficiently and see the shapes in the world with a new perspective. Whether you're working on a school project, designing something creative, or just curious about how things fit together, knowing about these angles is incredibly useful. It’s all about applying these ideas to figure out angles in real-life scenarios, boosting your skills in geometry and beyond.
| Definition | Image | Examples | |
|---|---|---|---|
| Complementary Angles | Two angles whose sum is 90 degrees. |  |
Two angles measuring 30 and 60 degrees. |
| Supplementary Angles | Two angles whose sum is 180 degrees. |  |
Two angles measuring 110 and 70 degrees. |
Having a basic understanding of angles and measuring angles will be helpful when it comes to understanding angle pairs.
Complementary Angles
Complementary angles are two angles whose measures have a sum of exactly 90 degrees. When combined, they form a right angle.
Identifying Complementary Angles
To determine if two angles are complementary, you can calculate the sum to find out if it is equal to $90^\circ$.
Are the angles $40^\circ$ and $45^\circ$ complementary?
- Add the angles together $40+45$.
- Answer the question, is this sum exactly $90^\circ$? If you answered yes, they are complementary! If the answer is no, as in $40+45=85$, then the answer is no, these angles are not complementary.
Are the angles $53^\circ$ and $37^\circ$ complementary?
- Add the angles together $53+37$.
- These angles have a sum of 90 degrees since $53+37=90^\circ$. These two angles form a right angle.
Finding a Complementary Angle
If one of the two angles are known, using subtraction can help to find the angle that is complementary to the given angle.
What is the measure of the missing complementary angle?
- Since a right angle is equal to 90 degrees, this is our total.
- The known value, in this case, $41^\circ$ can be subtracted from the total to find the missing complementary angle. $90-41=49$.
- $41^\circ$ and $49^\circ$ are complementary angles because they have a sum of $90^\circ$.
Finding missing angles is a skill that will help solve problems involving more complex angle relationships.
Supplementary Angles
Supplementary angles are two angles whose measures sum to 180 degrees, together forming a straight line.
Think of a straight ruler as an analogy. If two angles were placed at one end of the ruler, with their vertexes meeting at the same point and their sides extending along the ruler's length, these angles would be supplementary if they covered the entire 180-degree space opposite each other.
Identifying Supplementary Angles
To determine if two angles are supplementary, you can calculate the sum to find out if it equals $180^\circ$.
Are the angles $120^\circ$ and $60^\circ$ supplementary?
- Add the angles together $120+60$.
- Answer the question, is this sum exactly $180^\circ$? If you answered yes, they are supplementary!
Are the angles $130^\circ$ and $40^\circ$ supplementary?
- Add the angles together $130+40$.
- These angles have a sum of 170 degrees since $130+40=170^\circ$. These two angles do not form a straight line.
Finding a Supplementary Angle
If one of two angles is known, using subtraction can help to find the angle that is supplementary to the given angle.
What is the measure of the missing supplementary angle?
- Since a straight line is equal to 180 degrees, this is our total.
- The known value, in this case, $38^\circ$, can be subtracted from the total to find the missing supplementary angle. $180-38=142$.
- $38^\circ$ and $142^\circ$ are supplementary angles because they have a sum of $180^\circ$.
Supplementary and Complementary Angles – Application
A skateboard ramp forms an angle of $140^\circ$ with the ground. What is the measure of the angle between the ramp and a vertical line from the ground?
- Since a straight line measures $180^\circ$, and the ramp forms a $140^\circ$ angle with the ground, we're looking for the supplementary angle.
- Subtract the ramp's angle from $180^\circ$ to find the missing angle: $180 - 140 = 40$.
- The angle between the ramp and a vertical line from the ground is $40^\circ$.
Supplementary and Complementary Angles – Summary
Key Learnings from this Text:
- Complementary angles sum to 90 degrees and form a right angle.
- Supplementary angles sum to 180 degrees and create a straight line.
- Understanding these angle pairs is essential for solving various geometric problems without the use of a protractor.
Supplementary and Complementary Angles – Frequently Asked Questions
Transcript What are Supplementary and Complementary Angles?
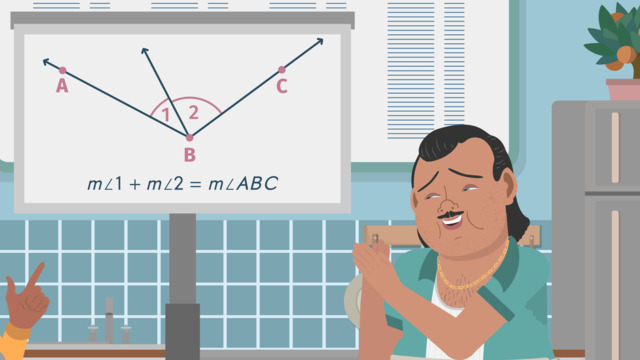
Pete is passionate about pizza pie! His thin crust, fresh mozzarella, and secret spicy tomato sauce drive his customers wild but Pete needs a better slicing and pricing strategy. So he calls in Caroline the Consultant. She'll know how to get Pete straightened out. Caroline's an expert in precise geometric work, and she'll explain to Pete what supplementary and complementary angles are. That'll help Pete slice and price to perfection. Pete explains his current system to Caroline, where people choose their own slices and are charged 5 cents per degree of the angles. Usually people choose two slices, and then Pete measures the angle of each slice. For example, this slice measures 60 degrees, and this slice measures 70 degrees. After adding them together to get 130 degrees, he charges 5 cents per degree. Caroline jumps in and shows Pete a shortcut. He can put the two slices together, so that the two angles are ADJACENT. Adjacent angles are angles that share a common point, and a common ray. In everyday terms, that means they are next to each other. Then he just has to measure the resulting single angle, which gives us the same result: 130 degrees. A hundred thirty degrees, times 5 cents, means these slices will cost six fifty. Adjacent angles will make Pete's work a lot easier! Let's examine a little more closely how adjacent angles work. Adjacent angles share a VERTEX and one side, but they DON'T overlap. Angle 1 and angle 2 are adjacent. Their sum is the same as the measure of the entire angle ABC. Pete is super grateful for Caroline's advice, but Caroline has an idea that will allow Pete to simplify his calculations even more, so he can focus fully on his passion: Pizza! She thinks Pete should slice his pizza so that the two slices always add up to either 180 degrees or 90 degrees. But why did she choose those numbers and not something like 70 or 170 degrees? Let's look at what happens if we cut a pizza in half. We can call this a STRAIGHT angle, and it's measure is 180 degrees! Now we can slice this half-pizza any way we want, and the two angles will add to 180 degrees. Any two angles which add to 180 degrees are called supplementary angles Here, angle 1 and angle 2 are supplementary. How much do two supplementary slices cost? 180 degrees, times 5 cents, gives us 9 dollars. Now let's see what happens when we cut a half a pizza in half again. Then we get quarters, and each quarter is 90 degrees. That's why she chose 90! Now we can slice the quarters however we want and the resulting angles will add to 90 degrees. Two angles which add to 90 degrees are called complementary angles. Here, angle 3 and angle 4 are complementary. And how much do two complementary slices cost? 90 degrees times 5 cents gives us four fifty. If Pete slices his pizza into supplementary and complementary pairs he won't have to measure any more angles to get the prices! Pete quickly slices his pies. But be careful Pete, don't mix them all up! Oh for Pete's sake! All right, well let's measure and match the supplementary and complementary pairs correctly. This slice measures 117 degrees and this one measures 63 degrees. Are they supplementary, complementary or neither? One seventeen and 63 is 180 degrees! They are supplementary angles! To check, we place the two slices ADJACENTand see that they form a STRAIGHT angle of 180 degrees. Ok, how about these two? This one measures 100 degrees, while this one measures 54 degrees. Adding them together gives us 154 degrees, which means they are neither supplementary nor complementary. And when we place them ADJACENT to each other, we can see that even more clearly. And what about these two slices? This one's 20 degrees and this one's 70 degrees. Together, they add up to 90 degrees! That means they are complementary angles! Placed adjacent, they form a RIGHT angle. That scrumptious pair will cost four fifty. Pete keeps his supplementary and complementary pairs together, waiting for a lucky customer to purchase them. While the pizza is bubbling in the oven, let's review what Pete learned. Supplementary angles add to 180 degrees. When adjacent to each other, they form a STRAIGHT angle. Complementary angles sum to 90 degrees. When adjacent, they form a RIGHT angle. Ok, the pizza is ready! Let's have a toast to delicious pizza pairs! Ah, Caroline and Pete COMPLEMENT each other nicely! But Caroline is a little miffed! Remember Pete, NEVER slice through the pepperonis!
What are Supplementary and Complementary Angles? exercise
-
What are complementary and supplementary angles?
HintsThere are different ways to help you remember the difference between complementary and supplementary angles.
Remember that complementary angles add up to 90 degrees, just like the corners of a square or rectangle. Think of "C" for "corner" or "right angle."
Supplementary angles add up to 180 degrees, forming a straight line when adjacent. Associate "S" with "straight line."
SolutionWhen two angles share a side and a vertex, they are known as adjacent angles. When two adjacent angles have a sum of $90^\circ$ they are called complementary angles. Angles that have a measurement of $90$ degrees are also known as right angles.
When adjacent angles are on a straight line, they have a measurement of $\bf{180^\circ}$. These angle pairs are known as supplementary angles.
-
Understand the difference between Complementary and Supplementary Angles.
HintsComplementary angles are two angles whose measures add up to 90 degrees, often forming a right angle when adjacent.
Supplementary angles are two angles whose measures add up to 180 degrees, often forming a straight line when adjacent.
SolutionComplementary Angles (Sum of $90^\circ$)
- $82^\circ$ and $8^\circ$
- $73^\circ$ and $17^\circ$
- $45^\circ$ and $45^\circ$
- $50^\circ$ and $40^\circ$
- $15^\circ$ and $75^\circ$
- $57^\circ$ and $123^\circ$
- $150^\circ$ and $30^\circ$
- $101^\circ$ and $79^\circ$
- $99^\circ$ and $81^\circ$
- $129^\circ$ and $51^\circ$
-
Apply your knowledge of complementary angles.
HintsComplementary angles are two angles that have a sum of $90$ degrees.
The square in the corner of an angle indicates that it is a right angle with a measurement of 90 degrees.
SolutionTo find the solution you can subtract the $62$ from $90$ because these angles are complementary and have a sum of 90 degrees.
$90-62=28$
$x=28^\circ$
-
Apply your knowledge of supplementary angles.
HintsSupplementary angles span the length of a straight line. Remember that two angles that span a line add up to 180 degrees.
Look at this example to better visualize supplementary angles.
SolutionThese two angles have a sum of $180$ degrees and are called supplementary angles. To find the missing angle, $121$ can be subtracted from $180$.
$180-121=59$
$y=59^\circ$
-
What are the definitions of different types of angles?
HintsAdjacent means 'next to.' In geometry, adjacent angles are like two pizza slices touching at a corner, sharing one side. They start from the same point and lie beside each other.
Straight lines have an angle measurement of 180 degrees.
What type of angles add to 180 degrees?
The sum of two supplementary angles are always greater than the sum of two complementary angles.
When two angles have a sum of 90 degrees, they also form what's known as a right angle.
SolutionStraight Angle - Angles with a measurement of 180 degrees.
Adjacent Angles - Angles sharing a common side and vertex.
Complementary Angles - Two angles that add to 90 degrees.
Supplementary Angles - Two angles that add to 180 degrees.
-
Apply your understanding of complementary and supplementary angles.
HintsThe symbol $\angle$ means angle.
Instead of saying "angle x", it can be written $\angle x$.
Complementary angles are two angles whose measures add up to $90^\circ$. They often form a right angle when adjacent. For example, if one angle measures $30^\circ$, its complementary angle must measure $60^\circ$, because $30^\circ + 60^\circ = 90^\circ$.
Supplementary angles are two angles whose measures add up to $180^\circ$. They typically form a straight line when adjacent. For instance, if one angle measures $110^\circ$, its supplementary angle must measure $70^\circ$, as $110^\circ + 70^\circ = 180^\circ$.
There are a total of 3 correct answers for this problem.
SolutionTrue statements:
- $\angle a = 49^\circ$
- $\angle b = 49^\circ$
- $\angle a = \angle b$

















Pete's Pizza Paradise
lol
cool