Additive Angles
Basics on the topic Additive Angles
Adding and Subtracting Angles
Did you know that you can add and subtract angles? Adding and subtracting angles happens, when an angle gets smaller or larger. If an angle gets larger, we speak of additive angles. Additive angles are made from adjacent angles. Additive means we can use addition or subtraction to solve for unknown angle measurements.
Adjacent Angles – Definition
What are adjacent angles?
Adjacent angles are two angles that are next to each other but do not overlap. They share the same vertex and one side. Adjacent angles have smaller angles and also a larger one created when they are combined.
Adjacent Angles Examples
The illustration below shows an example of how to add angles. The formula is rather simple. You add the adjacent angles to get a new whole.
When we know the measurements of the parts of an adjacent angle, we can add the parts together to find the measurement of the total angle created.
| part | + part | = whole |
|---|---|---|
| 20° | + 25° | = 45° |
If we add the two adjacent angles, we get a new whole of 45°.
Let’s look at an example of how to subtract angles. The illustration below shows an example of how to subtract angles. In order to subtract angles, you reverse the formula of how to add angles.
When we know the measurement of the whole angle created by adjacent angles, we subtract the measurement of the known part from the total to find the missing measurement.
| whole | - part | = part |
|---|---|---|
| 140° | - 30° | = 110° |
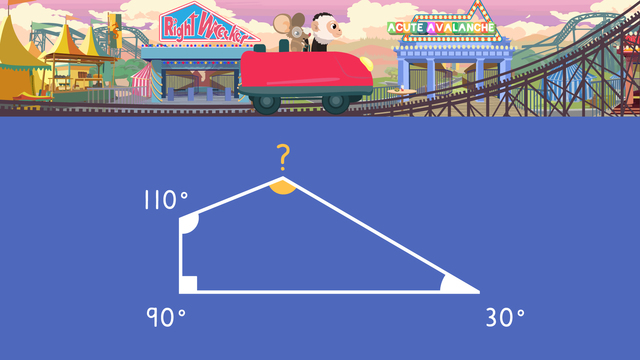
If we subtract 30° from our whole of 140°, we can conclude that our missing measurement is 110°!
Adding and Subtracting Angles – Further Practice
Following the video, there is additional additive angles practice for 4th with interactive exercises and worksheet. The additive angles worksheet will have more examples of adjacent angles that have missing angle measurements.
Transcript Additive Angles
Wow! Check out Nico and Nia's new hang gliders! Each one of them thinks they can make it go higher so they decide to have a FRIENDLY competition! As their gliders lift off the ground, we can see that their flight patterns have created several angles. These angles are ADDITIVE, which means we can use addition and subtraction to solve for the unknown angle measurements. "Additive Angles" Nico and Nia's flights have created ADJACENT ANGLES. Let's look at the angles and see the line and the vertex they share. Adjacent angles share the SAME VERTEX and ONE SIDE. Adjacent angles have smaller angles and also a LARGER one that is created when they are COMBINED. Let’s measure the adjacent angles made by Nico and Nia's flights. Nia’s flight made the first angle. We know that it measures twenty degrees... ...And Nico flew twenty-five degrees HIGHER. How can we solve to find the TOTAL angle of Nico’s flight? We know the parts, so we can ADD to find the whole angle. What is the sum of twenty and twenty-five? Zero plus five is five, and two plus two make four. Forty-five! Nico’s flight was at a forty-five-degree angle! NICO WON! "No, no, no!" "It's the BEST OUT OF THREE That's how we decide the winner!" For the NEXT round, they decide to take off from a hill to give themselves more height. What observations can you make about the angles made by where they took off and the directions they went in? We can see the widest angle is made from the tree to the bottom of the hill, and Nia and Nico have now created a total of THREE angles inside. Nico flew at a sixty-six-degree angle off the ground. Nia's take-off was seventeen degrees higher than Nico's, (...) AND her flight path was twenty-six degrees from the tree. What do we need to do to find the angle measurement made by the tree to the ground? We need to ADD the three angles to find the sum. What is the total of sixty-six, plus seventeen, plus twenty-six? In the ones, six plus seven is thirteen. What is six more? Nineteen. In the tens, add six plus one plus two to make nine, (...) and now add one more. The total angle is one hundred nine degrees. Since Nia flew higher in round two, it's time to get SERIOUS for the tiebreaker! BLAST OFF! Nico and Nia took off in opposite directions and have created new adjacent angles with the ground. What do you observe about these angles? Here we have given the total of the widest angle, and the measurement of Nia's angle. What do you think we need to do to find the MISSING angle, which is the DIFFERENCE between Nico and Nia’s flight? In this problem, we will need to SUBTRACT the KNOWN angle from the WHOLE to find the missing angle. This means whole minus part equals part. We set up the problem, one hundred forty MINUS thirty. Start subtracting with the ones. There are zero ones. Four minus three equals one. We still have one in the hundreds because we didn’t have any to take away. The unknown angle measures one hundred ten degrees. Remember, Adjacent angles are ADDITIVE, so we can use known angle measurements to solve for the unknown parts. In a group of adjacent angles that are joined together by one vertex, we can add the smaller angles to find the sum of the degrees of the widest angle. Or sometimes, we know the combined angle measurement and we need to find a missing part. We solve these by taking the angle degrees we do know and subtracting that number from the whole. [Nia's about to fall] "WOAH!WOAH!WOAH!" "NICO!"HELP!" "NIA!! HANG ON!" "I'M ON MY WAY!" “Thank you, Nico!” “DO OVER?”
Additive Angles exercise
-
Testing the gliders.
HintsRead the text to find the angles of Nico and Nia and where they can be marked on the image.
Find the largest angle by adding the two smaller angles together.
SolutionNia's glider made an angle of 40°, this is shown in green. Nico's glider made and angle of a further 38°, this is shown in pink. When we add this on to Nia's angle: 40° + 38° = 78°. This is shown in orange.
-
What is the angle of Nico's flight?
HintsSince Nico flew 20° higher than Nia, we need to add 20° onto Nia's angle to find the angle of Nico's flight.
What is 20 + 35?
Solution- To find the angle that Nico's glider flew from the ground, we need to add 20° to Nia's angle, since we know that Nico flew 20° higher than Nia.
- Nia flew 35° and Nico flew 20° higher.
- 35° + 20° = 55°.
- So Nico's glider flew 55° from the ground.
-
Find the missing angle.
HintsTo find a missing angle when we know the total of 2 adjacent angles, take the known angle from the total.
What is the calculation that needs to be completed?
Solution- Nico and Nia both make angles with their gliders, that total 75°.
- Since Nia's angle is 43°, to find Nico's angle we must subtract 43° from 75°.
- This will give us the missing angle.
- When we take 43° from 75° we get 32°, so this is the angle made by Nico.
-
Missing angles.
HintsWhere two adjacent angles have been given, the total of these can be found by adding them together.
If one adjacent angle has been given, subtract this from the total to find the missing angle.
Look carefully where the question mark is to find the correct missing angle.
Remember that we can recognize a right angle as 90° because it is marked by a small square like this,
SolutionThe solution image shows how the missing angle can be found for each question.
-
Which image shows adjacent angles?
HintsAdjacent angles share one straight line and a vertex.
Adjacent angles are next to each other. Remember, we are just looking at the angles marked in pink and green.
Solution- The angles shown in a) are adjacent because they share a vertex and one straight line. This is the CORRECT answer.
- The angles shown in b) do not share a vertex or straight line.
- The angles shown in c) share a vertex but do not share a straight line.
- The angles shown in d) share a vertex but do not share a straight line.
-
Angles in a circle.
HintsLook at the right angles marked in the circle, can this help you to find the missing angles?
Remember that there are 90° in a right angle and 180° on a straight line.
Solution- To find angle a: We can see that there is a little square that means right angle, this means that the angle is 90°.
- To find angle b: The top right quarter of the circle is shown by a right angle, this is then split into two adjacent angles. One of these is given as 37°. To find the missing angle we need to subtract 37° from 90° to get 53°.
- To find angle c: There are two right angle symbols on the top half of the circle, so we know that the line going horizontally across the circle is a straight line and angles on a straight line must add up to 180°. As one of these adjacent angles is given here as 132° we then subtract 132° from 180° to get 48°.