Finding Angles Using Equations


Basics on the topic Finding Angles Using Equations
After this lesson you will be able to solve for unknown angles using facts about parallel lines, transversals, and congruent angles.
The lesson begins by teaching you that parallel lines cut by a transversal have many congruent angles. It leads to identifying corresponding, alternate interior and alternate exterior angles. It concludes with solving for missing angle measurements.
Learn about transversals and angles by helping the raccoons work in a new city without wrecking their shopping carts!
This video includes key concepts, notation, and vocabulary such as: transversal (a line which intersects two other lines in a plane); corresponding angles (angles that are in the same position around each vertex); alternate interior angles (two angles on opposite sides of transversal and interior to the parallel lines); and alternate exterior angles (two angles on opposite sides of transversal and exterior to the parallel lines).
Before watching this video, you should already be familiar with complementary, supplementary, vertical and adjacent angles.
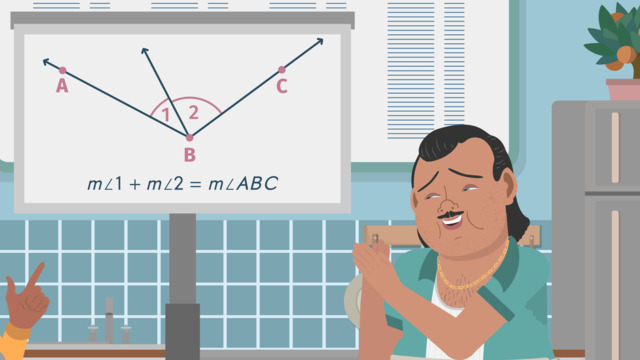
After watching this video, you will be prepared to learn that the sum of interior angles of a triangle is equal to 180 degrees.
Common Core Standard(s) in focus: 8.G.A.5 A video intended for math students in the 8th grade Recommended for students who are 13-14 years
Transcript Finding Angles Using Equations
These 3 raccoons are riding their shopping cart around town in search of another meal. It's been slim pickins lately, so they take a break at their favorite stump to discuss a possible plan. Should they start working in a new city? Maybe but remember what happened last time they visited unfamiliar territory. Some of the angles were too sharp and they wrecked their shopping carts. If they want to avoid the same fate, they'll need to do careful calculations involving transversals. The raccoons zero-in on the Grocery District- this part of town is stacked with supermarket trash! And the layout is perfect: look at all the parallel roads! These parallel lines are cut by transversals which means there are many congruent angle pairs. Congruent angles are angles that are equal in measure. Do you remember which angle pairs are congruent when two parallel lines are cut by a transversal. Corresponding angles are one such pair. Corresponding angles are angles that are in the same position around each vertex. Can you see any pairs of corresponding angles? Angles 1 and 5 are a pair of corresponding angles. So are 2 and 6, 3 and 7 and 4 and 8. Alternate interior angles are also congruent. Can you find the alternate interior angles here? 3 and 5 and 4 and 6 are pairs of alternate interior angles, since they are on alternate sides of the transversal and interior to the parallel lines. Alternate exterior angles are on alternate sides of the transversal and exterior to the parallel lines and are also congruent. Which angle pairs are alternate exterior? 1 and 7 and 2 and 8 are alternate exterior angles. As long as the lines are parallel, all the angle pairs we identified are congruent. We can help the raccoons plan their route by finding the measurements of some of these angles. The raccoons measured angle 1 to be 25 degrees. What is the relationship between angle 1 and angle 5? 1 and 5 are corresponding angles, so they are congruent. That means angle 5 is also 25 degrees. How does angle 5 relate to angle 3? 5 and 3 are alternate interior angles, so they are congruent. That means angle 3 is also 25 degrees. How does angle 1 relate to angle 7? 1 and 7 are alternate exterior angles, and they are also congruent. That means angle 7 is 25 degrees. We found out the measure of all these angles after measuring just one, since parallel lines cut by a transversal have many pairs of congruent angles. Now let's take a look at a busier part of town. Here there are lots of supermarkets, and plenty of intersections! There are two parallel lines and there are also two transversals! We only know the measures of angle 1, which is 55 degrees and angle 2, which is 45 degrees, but we want to find the measures of angles 3, 4, and 5. Since together they form a straight angle, the sum of these three angles must equal 180 degrees. But first let's focus on one transversal at a time, which will allow us to use some of the relationships we saw before. First, the transversal on the left. How does angle 1 relate to angle 3? They are corresponding, which means they are congruent, so angle 3 is 55 degrees. Now let's focus on this transversal. How does angle 2 relate to angle 4? They are alternate interior, which means they are congruent, so angle 4 is 45 degrees. Plugging the values 55 for angle 3 and 45 for angle 4 into our equation from before gives us measure of angle 5 equal to 80 degrees. The raccoons have all the angles to make their attack! While they accelerate towards their prize, let's review the tools they used to hatch their daring plan. If parallel lines are cut by a transversal, we have several congruent angle pairs. Corresponding angles are in the same position around each vertex. Alternate interior angles are on opposite sides of the transversal, and interior to the parallel lines. Alternate exterior angles are on opposite sides of the transversal, and exterior to the parallel lines. When solving for missing angle measurements, if there is more than one transversal, focus on one at a time. Keep your raccoon eyes ready for two or more angles that are on a straight line. They sum to 180 degrees, and you can often write and solve an equation to find an unknown angle. They've reached their destination, but it looks like the raccoons forgot about the hills! They'll need to take their geometry to three dimensions next time.