Volume of a Cone
Basics on the topic Volume of a Cone
Volume of a Cone
Just like the familiar cylinder, cones are everywhere in our daily lives, from ice cream cones to traffic cones. Learning the volume of a cone is not just an academic exercise but a practical skill that can be applied in various real-world scenarios.
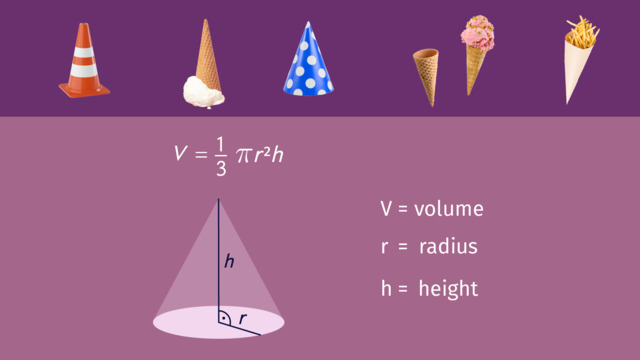
The volume of a cone is the amount of space inside a cone. This volume is calculated using the formula $V=\frac{1}{3} \pi r^{2}h$, where $V$ stands for volume, $r$ is the radius of the cone's base, and $h$ is its height.
Understanding the Volume of a Cone
The key to understanding the volume of a cone is to realize that it is a third of the volume of a cylinder with the same base and height. This concept is crucial in grasping why the formula includes the $\frac{1}{3}$ factor.
Volume of a Cone – Cubic Units
Determining the Volume of Simple 3D Shapes involves using specific formulas, and it's equally important to label the final results with the appropriate units.
Choosing the Correct Volume Units
Volume in cones, like all three-dimensional objects, is expressed in cubic units. These units depend on the measurement of radius and height. For instance, if these measurements are in centimeters, the volume will be in cubic centimeters (cm³).
Volume of a Cone – Step-by-Step Instructions
Here’s how to calculate the volume of a cone, step by step:
| Step Number | Directions | Example |
|---|---|---|
| 1 | Identify the radius and height of the cone. | Radius $r = 3$ cm, Height $h = 12$ cm |
| 2 | Substitute the values into the formula $V=\frac{1}{3} \pi r^{2}h$. | $V=\frac{1}{3} \pi \times 3^2 \times 12$ |
| 3 | Calculate the volume, attending to rounding rules. | $V=\frac{1}{3} \pi \times 9 \times 12 = 36\pi$ cm³ approx. $113.1$ cm³ |
| 4 | Write the final answer with the correct units. | Volume of the cone is $113.1$ cm³ |
Let’s work through an example of calculating the volume of a cone.
Imagine a party hat shaped like a cone with a radius of $4$ in. and a height of $10$ in.
In addition to cones, you can determine the Volume of Prisms by employing different formulas while following a parallel approach.
Calculating Volume 'In Terms of Pi'
Calculating volume 'in terms of $\pi$' means leaving $\pi$ as is, without converting it to a decimal. This method is more precise and often used for exactness in mathematical and scientific calculations.
Let’s practice calculating the volume of a cone and leaving the answer in terms of $\pi$.
Volume of a Cone – Real-World Problems
Cones are everywhere in our daily life, not just in math books. Think of a party hat or a funnel in a kitchen. Knowing how to calculate their volume can be really handy in real-world situations.
Let's explore real-world problems involving cones.
Volume of a Cone – Exercises
Now that you have learned the formula and process for calculating the volume of a cone, try these exercises!
Volume of a Cone – Summary
Key Points from this Text:
- The formula for calculating the volume of a cone is $V = \frac{1}{3} \pi r^2 h$.
- To find the volume, identify the radius and height of the cone.
- Substitute the values into the formula and use a calculator to compute, rounding to the nearest tenth or leaving in terms of $\pi$.
- Real-world examples of cones include ice cream cones and traffic cones, making this concept widely applicable.
Volume of a Cone – Frequently Asked Questions
Transcript Volume of a Cone
These objects all have one thing in common; they're cones! But how much space is inside? We can find out by finding the 'volume of a cone'. A cone is a three-dimensional shape made from a circular base and a curved surface that ends in a point. To calculate this, we will use the formula volume equals one-third, times pi, times the radius squared, times the height. The radius is the distance from the center of the circular base to the outside. The height is the vertical distance between the center of the base to the tip of the cone. Time to look at an actual cone and find its volume. Find the volume of the cone, and round your answer to the nearest tenth of a centimeter. First, write the formula you are using to find the volume. V equals one-third, pi, r squared, h. Next, let's identify the radius and height of the cone. The radius, or r equals four centimeters. The height, or h is equal to nine centimeters. These values can be substituted into the formula. First multiply the numbers we know, leaving pi for the end. One-third times four squared, times nine, is equal to forty-eight. Remember, pi is approximately three point one four and so on, and we will use a calculator to help us find the volume. The product of forty-eight and pi is approximately one hundred fifty and eight tenths. We always represent volume with cubic units. The volume of the cone is approximately one hundred fifty and eight-tenths centimeters cubed. Let's try another example! Find the volume of the cone, and round your answer to the nearest tenth of an inch. Starting with our formula, the radius for this cone is three inches and the height is five, which we substitute in the formula. Leaving pi for the last step, the product of one-third, three-squared, and five is fifteen. Fifteen multiplied by pi is approximately forty-seven and one-tenth. And don't forget to include the units in your final answer! Let's try one more, but this time find the volume of the cone in terms of pi. This means that we will not be calculating pi on our calculator, but rather leaving pi as a symbol in our final answer. Start by writing down the formula, and then ask yourself, what are the radius and height? The radius is six centimeters, and the height is twelve. Pause the video here and rewrite the formula for volume including the known values. Your formula now should look like this! To find the final solution in terms of pi, we will calculate all but the pi in our calculators. The volume equals one hundred forty-four, pi, centimeters cubed, which is an exact measurement since it was not rounded. Let's wrap this all up! This is the formula used to find the volume of a cone. The r is the radius, and h is the height of the cone. Using the formula, substitute in any known values, solve, and be sure to always include your cubic units. Stay calm, don't get cone-fused and you can find the volume of these 3D shapes with ease!
Volume of a Cone exercise
-
Understand what the volume of a cone measures.
HintsA cone is a three-dimensional shape, such as this party hat! Some other common cones are:
- ice cream cones
- traffic cones
- funnels
Like any three-dimensional shape, the volume measures the interior 3D space.
SolutionThe volume of a cone measures the amount of space inside the cone.
-
Using a formula to find the volume of a cone.
HintsThe radius is the distance from the center of a circle to the outside.
The height of a cone measures the distance from the center of the circle to the tip of the cone. This line of measurement must be perpendicular to the base.
Substituting a value for a variable means replacing the variable with a specific number in an equation or expression, which allows you to solve or simplify it.
For example, if we know that $x=4$, and we need to evaluate $x - 6$, we would then substitute to write $\bf{4} - 6$.
SolutionThe radius of the cone is $\bf{2\:cm}$.
The height of the cone is $\bf{4\:cm}$.
The formula used to find the volume of the cone is $\bf{V=\frac{1}{3} \pi r^2h}$.
Once the values for the radius and height are identified, substitute them for the appropriate variables, and write the equation: $\bf{V=\frac{1}{3} \pi (2^2)(4)}$.
-
Volume of a Cone.
HintsTo use a formula, substitute in the values for the corresponding variable.
For example, the cone seen here has the following measurements:
$r=3\:cm$
$h=2\:cm$
The formula to find the volume is: $V=\frac{1}{3}\pi\: r^2h$
When the values are substituted, the equation is: $V=\frac{1}{3}\pi \:(3^2)2$.
SolutionSubstitute the values of the radius and height into the formula, $V=\frac{1}{3}\pi \:r^2h$, for $r$ and $h$
-
Find the volume of a cone.
HintsThe formula for volume of a cone is $V=\frac{1}{3}\pi r^2h$.
In terms of $\pi$ means to not calculate $\pi$ and leave it as the symbol in the answer instead.
To calculate the volume of this cone (in terms of $\pi$), first substitute in the known values.
$V=\frac{1}{3} \pi \:r^2h$
$V=\frac{1}{3} \pi \:(6^2)(15)$
Then calculate the product of all the numbers except pi.
$\frac{1}{3}(6^2)(15)=180$
Lastly, the pi symbol can be written next to the product, to show the exact value for the volume.
$V=180\pi\:m^3$
Solution$r=2$
$h=6$
$V=\frac{1}{3}\pi r^2 h$
$V=\frac{1}{3} \pi (2^2) (6)$
$\frac{1}{3}(2^2)(6)=8$
$V=8\pi\:in^3$
-
Identify the variables in the formula for the volume of a cone.
HintsRemember, this problem is about the volume of a cone. Which measurements are important to finding this value?
Look at the cone. What measurements do the $h$ and $r$ represent?
SolutionThe variables and symbols in the Volume of a Cone formula are:
V = volume
r = radius
h = height
$\pi$ = pi
-
Calculate the volume of a cone using the formula.
HintsFormula for the volume of a cone: $V=\frac{1}{3}\pi r ^2 h$
If you do not have a calculator handy, you can use the approximate value of pi, $3.14...$.
Remember, the $r$ is the radius of the cone, and the $h$ is the height of the cone.
Make sure to take your time and show your work to find the volume.
- Identify the radius and height.
- Substitute these values in the formula $V=\frac{1}{3}\pi r^2 h$.
- Calculate the volume and round to the nearest tenth.
SolutionThe volume for each cone:
V $\approx$ $804.2\:cm^3$
V $\approx$ $212.1\:cm^3$
V $\approx$ $83.8\:cm^3$
Steps to solve the first one:
$V=\frac{1}{3}\pi r ^2 h$
$r=8$
$h=12$
$V=\frac{1}{3}\pi (8^2) (12)$
$V=256 \pi$
$V \approx 804.2\:cm^3$