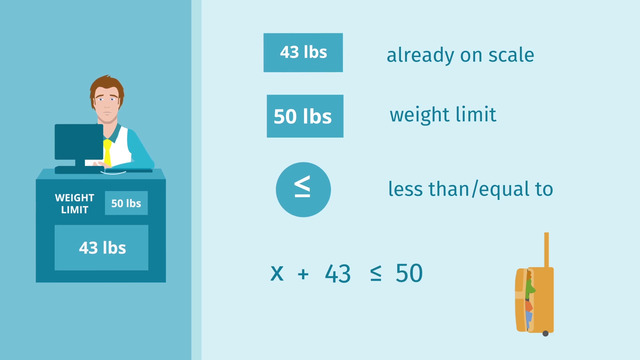
Solving Two-Step Inequalities

Solving Two-Step Inequalities exercise

• Determine how Miss. Crazycat can estimate the number of cats.

  Hints

  Let's assume that Miss Crazycat owns $15$ cats. $13$ cats eat fish.

  Pay attention to the rules of PEMDAS:

  • Evaluating parentheses first:

  $(3+4)\times 2=7\times 2=14$

  • You get the same result by using the Distributive Property:

  $(3+4)\times 2=3\times 2+4\times 2=14$

  • $5x=60$ is an equality
  • $5x<60$, $5x\le60$, $5x>60$ and $5x\ge 60$ are inequalities

  Solution

  Let's try to help Miss Crazycat solve her problem, by estimating how many cats she has living in her home.

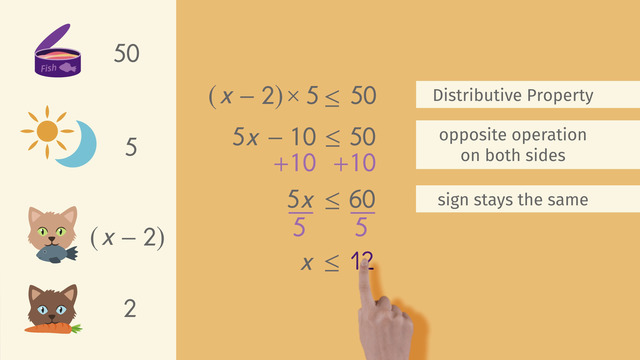
  First of all, we assign the unknown number of cats to the variable $x$. Because two cats are vegetarian, there are $x-2$ cats that eat fish. We know that fewer than $50$ cans of fish will be eaten in $5$ days. Writing this mathematically, we get the inequality $(x-2)\times 5\le 50$.

  Using the Distributive Property, we multiply the contents of the parentheses by $5$. Doing this leaves us with $5x-10\le 50$.

  We perform the opposite operation of $-10$ on both sides, which gives us $5x\le 60$.

  Last but not least, we divide by $5$. We have to pay attention to the sign of $5$. It's positive, so the sign $\le$ stays the same: $x\le 12$.

  So, now Miss Crazycat knows that the number of cats in her apartment is less than or equal to $12$.

• Estimate the number of cats in Miss. Crazycat's apartment.

  Hints

  The inequality we need to solve is $(x-2)\times 5\le 50$.

  This leads to $x\le 12$.

  Miss Crazycat knows that two of her cats are vegetarians.

  Miss Crazycat's cats eat $50$ cans of fish in $5$ days. Let's assume that neither Miss Crazycat nor the two vegetarian cats eat any cans of fish. So, there has to be at least one cat that eats fish.

  A negative number of cats is not possible. Poor $-1$ cat!

  Solution

  Let's look at the given information:

  • We assign the unknown number of cats to the variable $x$.
  • Miss Crazycat has two vegetarian cats, so $x-2$ represents the number of cats that eat fish.
  • Her cats eat $50$ cans of fish in $5$ days.

  Together with this information, we can write the following inequality: $(x-2)\times 5\le 50$. Solving for $x$ gives us $x\le 12$.

  Now we have two vegetarian cats and at least one fish-eating cat, so Miss Crazycat has at least $3$ cats.

  We can conclude that Miss Crazycat has between $3$ and $12$ cats. We can express this mathematically as $3\le x\le 12$.

  Unfortunately, Miss Crazycat still doesn't know if she has fewer than $10$ cats...

• Write an inequality to describe a number line.

  Hints

  First, transform each inequality to a simpler version.

  $\begin{array}{lcl} x+5&\le&15\\ ~~~\color{#669900}{-5}&&\color{#669900}{-5}\\ x&\le& 10 \end{array}$

  You can draw the graph of $x\le10$ by drawing a closed circle at $10$ and an arrow pointing to the left.

  You can draw the graph of $x \ge 10$ by drawing a closed circle at $10$ and an arrow pointing to the left.

  Solution

  Drawing graphs of inequalities is a good way to help you understand their solution sets.

  • For the inequality $x+2\le8$, subtracting $2$ from both sides gives us $x\le 6$. You can draw the graph of $x+2\le8$ by drawing a closed circle at $6$ and an arrow pointing to the left.
  • For the inequality $x-2\ge4$, adding $2$ to both sides gives us $x\ge 6$. You can draw the graph of $x\ge6$ by drawing a closed circle at $6$ and an arrow pointing to the right.
  • For the inequality $x+4\ge 11$, subtracting $4$ from both sides gives us $x\ge 7$. You can draw the graph of $x\ge7$ by drawing a closed circle at $7$ and an arrow pointing to the right.
  • For the inequality $x+6\le 13$, subtracting $6$ from both sides gives us $x\le 7$. You can draw the graph of $x\le7$ by drawing a closed circle at $7$ and an arrow pointing to the left.

• Describe how Miss. Crazydog can estimate the number of dogs living in her home.

  Hints

  It's a good idea to check your solution. The total number of cans of normal dog food eaten by Miss Crazydog's dogs should be $56$.

  Use Opposite Operations:

  • Multiplication ($\times~\longleftrightarrow~\div$)
  • Division ($\div~\longleftrightarrow~\times$)
  • Addition ($+~\longleftrightarrow~-$)
  • Subtraction ($-~\longleftrightarrow~+$)

  Solution

  It's not raining cats and dogs, but nevertheless Miss Crazycat and her friend Miss Crazydog have the same problem. They've lost count of how many cats or dogs they have living in their homes.

  Miss Crazydog knows that all but one of her dogs eat $2$ cans of dog food everyday, and one of her dogs is a vegetarian, so this dog does not eat canned dog food. Her dogs eat $56$ cans in total.

  $x$ is the number of dogs, and $x-1$ the number of non-vegetarian dogs.

  This gives us the equation $(x-1)\times 2\times7=(x-1)\times 14=56$.

  First, we use the Distributive Property to get

  $14x-14=56$

  Now we add $14$ on both sides of the equation sign, leaving us with:

  $14x=70$

  Finally, by dividing by $14$ on both sides of the equation, we get the solution:

  $x=5$

  Miss Crazydog has $5$ dogs in total, $1$ vegetarian dog, and $4$ meat eaters.

  Let's check and see if this solution is correct: $4$ dogs eating $2$ cans each of seven days leads to $4\times 2\times 7=56$. That's correct!

• Describe the meaning of the inequality sign $\le$.

  Hints

  $\le$ means less than OR equal to.

  $<$ means less than.

  There's a difference between less than and at most as well as between more than and at least.

  Solution

  The number of Miss Crazycat's cats can be expressed using the inequality $x\le 12$.

  What does this mean? The number of cats is, at most, $12$.

  • Less than $12$ is written as $x<12$.
  • More than $12$ is written as $x>12$.
  • At least $12$ is written as $x\ge 12$.
  • Equal to $12$ is written as $x=12$.

• Solve the following inequalities.

  Hints

  Use Opposite Operations:

  • Multiplication ($\times~\longleftrightarrow~\div$)
  • Division ($\div~\longleftrightarrow~\times$)
  • Addition ($+~\longleftrightarrow~-$)
  • Subtraction ($-~\longleftrightarrow~+$)

  You can solve an inequality in a manner similar to solving equalities. But keep in mind that multiplying or dividing by a negative number flips the sign. For example:

  $2<x$ but $-2>-x$

  You can solve each inequality in two steps.

  The sign of only one inequality flips.

  Solution

  How do you solve a two-step inequality? The process is similar to solving two-step equations, but in order to isolate the variable in an inequality, we have to pay special attention when we multiply or divide by negative numbers. In these special cases, we have to flip the inequality sign.

  Take a look at this example:

  $2<3$ is obviously true. If we multiply by $-2$ we get $-4$ on the left an $-6$ on the right side. So the inequality $-4>-6$ is valid. Notice how we flipped the sign?

  $\begin{array}{rclcl} 2x+4&<& ~10\\ \color{#669900}{-4}&&\color{#669900}{-4}&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ 2x&< &~~~6\\ \color{#669900}{\div2}&&\color{#669900}{\div2}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&<& ~~~3 \end{array}$

  It works the same for the following equations:

  $\begin{align*} 3(x+1)&< 15\\ x+1&< 5\\ x&< 4 \end{align*}$

  ... and ...

  $\begin{align*} 3x+1&< 19\\ 3x&< 18\\ x&< 6 \end{align*}$

  Let's take a closer look at an inequality where the sign flips:

  $\begin{array}{rcrcl} -2x+8&<& 14~~\\ \color{#669900}{-8}&&\color{#669900}{-8}~~&&| (+~\leftrightarrow~-) \text{ Opposite Operations}\\ -2x&<& 6~~\\ \color{#669900}{\div(-2)}&&\color{#669900}{\div(-2)}&&| (\times~\leftrightarrow~\div) \text{ Opposite Operations}\\ x&>& -3~~&&|\text{ Flip the Sign!} \end{array}$