Relating Scale Drawings to Ratios and Rates

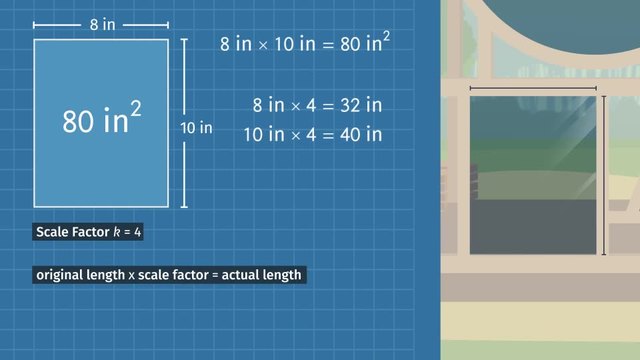
Malique is a young artist who is fascinated with the diversity of animal life, both past and present. He has come to the museum to make sketches of some interesting creatures. This woolly mammoth catches his eye, but fitting such a giant beast in his small sketchbook will be a challenge! Malique will need to create a scale drawing, then use his knowledge of ratios to make sure it’s accurate. Malique draws a sketch of the mammoth. Is his sketch larger or smaller than the actual mammoth? Smaller of course! His drawing needs to be smaller than the original to fit in the sketchbook. But is his drawing an accurate REDUCTION of the original? Because a reduction is a proportionally scaled-down image of the original, we’ll need to take some measurements, and see if the drawing is proportional to the original. If it is, then we can say it’s a SCALE drawing, and a true reduction! The original mammoth is gigantic! Its height is 250 centimeters. That's basically the height of two 8-year-olds. The mammoth's length is 400 centimeters. Almost the length of the average car. What a fantastic creature! Malique’s drawing has a height of 5 centimeters and a length of 8 centimeters. A mammoth can fit in his sketchbook after all! Let's organize the information using a table to see if it is a true scale drawing. First, we'll record the original height and length. Followed by the drawing height and length. This gives us our organized information all written in centimeters. An image is a scale drawing if its corresponding measurements are proportional to those of the original. In order to find out if they are proportional, we need to see if the ratios of corresponding measurements are equal. That means we should create ratios of height to height, and length to length and write the ratios of drawing to original. This will help us find the constant of proportionality. So, let’s substitute our known values for the heights into the ratios. That’s 5 to 250. And the ratio of the drawing length to the original length is 8 to 400. It’s hard to tell if they’re the same, so let’s reduce each ratio. 5 over 250 reduces to 1 over 50. 8 over 400 reduces to 1 over 50 as well! The ratios are equal, which means Malique has made an accurate scale drawing. 1 over 50 is the constant of proportionality 'k', which represents the relationship between the scale-drawing and the original. When the constant of proportionality is between 0 and 1, the image is a reduction of the original. Good job, Malique, on creating a scale drawing reduction. What are you drawing next? Bugs? Really, Malique? Well I guess these beetles are such intricate little creatures. How will Malique show the world their complexity? Do you think he’ll want to sketch a reduction or an enlargement? An enlargement would be best to highlight their features! An enlargement is a proportionally scaled-up image of the original. The original beetle is 6 centimeters long by 2 centimeters wide. And Malique’s drawing is 12 centimeters long by 6 centimeters wide. Let's organize this information using a table. Similar to before, we'll record the original length and width. Followed by the drawing length and width. Again this gives us our organized information all written in centimeters, so that we can easily identify the ratios. If the ratios of corresponding measurements are the same, the drawing will be a true enlargement. Remember, we should first set-up ratios of corresponding measurements, such as length to length or width to width. And keep the ratio order of drawing to original. The ratio of the lengths is 12 to 6, and the ratios of widths is 6 to 2. We'll need to reduce the ratios to see if they are equal. 12 over 6 reduces to 2 and 6 over 2 reduces to 3? Those are NOT the same! The ratios are not proportional. In fact, if you look closely at the drawing, you can see that it’s a bit distorted. Malique's drawing is too wide! To draw a true enlargement, the drawing width should be 4 cm. So, instead of a drawing width of 6 centimeters from our earlier calculations let’s correct it to be 4. That means the ratio of the widths simplifies to be two as well. Two is our constant of proportionality. Since the ratios are equal they are proportional! Therefore, we now have a true scale drawing. It is an enlargement because the constant of proportionality, 'k', is greater than 1. Let’s review what we've learned. To determine if we’ve got a scale drawing, set-up two ratios of corresponding measurements. Use the ratio drawing to original to calculate the constant of proportionality. If the ratios are the same then the drawing and original are proportional. This proportionality means we DO have a scale drawing. It's a reduction if the constant of proportionality is between 0 and 1. And it's an enlargement if the constant of proportionality is greater than than one. Great sketches, Malique. You really—WAIT. What is THAT? Maybe, uh, just an enlargement of one of the exhibits? Ah c’mon, man. That's not cool!