Prime and Composite Numbers — Let's Practice!
- Prime and Composite Numbers – Introduction
- What Are Prime and Composite Numbers?
- Importance of Telling Apart Prime and Composite Numbers
- Simplifying Fractions
- Solving Mathematical Problems

Basics on the topic Prime and Composite Numbers — Let's Practice!
Prime and Composite Numbers – Introduction
Get ready because today we're going to practice identifying prime and composite numbers! Knowing the difference between prime and composite numbers helps us understand the building blocks of all numbers. Ready? Let's go!
What Are Prime and Composite Numbers?
Prime numbers are numbers that have exactly two distinct positive divisors: one and itself. Composite numbers have more than two positive divisors.
Let's start with some examples to help us understand prime and composite numbers better!
Importance of Telling Apart Prime and Composite Numbers
Understanding the difference between prime and composite numbers is a fundamental skill in mathematics. Here are some reasons why it is important:
Simplifying Fractions
Knowing whether numbers are prime or composite helps in simplifying fractions. Prime numbers have fewer factors, which makes it easier to determine the the greatest common factor and simplify fractions effectively.
Solving Mathematical Problems
Many mathematical problems, especially those involving factors and multiples, require an understanding of prime and composite numbers. Recognizing these numbers aids in solving problems more efficiently and accurately.
Prime and Composite Numbers – Step by Step
We'll look at some numbers and list all their factor pairs to determine if they are prime or composite.
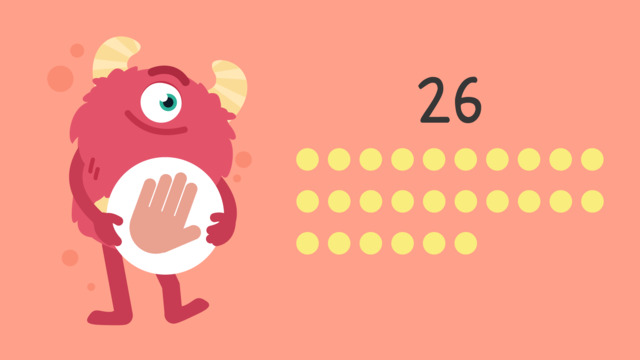
- Example 1: Is 26 Prime or Composite?
Solution: - Factor pairs of 26: 1 and 26, 2 and 13 - Since 26 has more than two factor pairs, it is composite.
Did you also get that 26 is composite? Great job!
- Example 2: Is 31 Prime or Composite?
Solution: - Factor pairs of 31: 1 and 31 - Since 31 has only two factor pairs, it is prime.
Did you also get that 31 is a prime number? Awesome!
- Example 3: Is 77 Prime or Composite?
Solution: - Factor pairs of 77: 1 and 77, 7 and 11 - Since 77 has more than two factor pairs, it is composite.
Did you also get that 77 is a composite number? Excellent!
- Example 4: Is 97 Prime or Composite?
Solution: - Factor pairs of 97: 1 and 97 - Since 97 has only two factor pairs, it is prime.
Did you also get that 97 is prime? Fantastic!
Prime and Composite Numbers – Practice
Now, let's practice identifying prime and composite numbers with some more examples.
Spot the Prime and Composite Numbers – Application
Prime and Composite Numbers – Summary
Key Learnings from this Text:
- Prime numbers have exactly two distinct positive divisors: one and itself.
- Composite numbers have more than two positive divisors.
- Listing all factor pairs of a number helps determine if it is prime or composite.
Keep practicing identifying prime and composite numbers to become a math expert! Check out more fun math activities and exercises on our website.
Prime and Composite Numbers – Frequently Asked Questions
Transcript Prime and Composite Numbers — Let's Practice!
Razzi says get these items ready because today we're going to practice Prime and Composite Numbers It's time to begin! List all the factor pairs of the following numbers to determine if the number is prime or composite. Is twenty-six, PRIME, or COMPOSITE? Pause the video to work on the problem and press play when you are ready to see the solution! The factor pairs of twenty-six are one and twenty-six... and two and thirteen. Did you also get that twenty-six is COMPOSITE? Let's tackle the next problem! Is thirty-one, PRIME, or COMPOSITE? Pause the video to work on the problem and press play when you are ready to see the solution! The factor pairs of thirty-one are one and thirty-one. There are no more factor pairs. Did you also get that thirty-one is a PRIME number? Let's tackle the next problem! Is the number seventy-seven, PRIME, or COMPOSITE? Pause the video to work on the problem and press play when you are ready to see the solution! The factor pairs of seventy-seven are one and seventy-seven... and seven and eleven. Did you also get that seventy-seven is a COMPOSITE number? Let's tackle the final problem! Is ninety-seven, PRIME or COMPOSITE? Pause the video to work on the problem and press play when you are ready to see the solution! The factors of ninety-seven are one and ninety-seven. There are no more factor pairs. Did you also get that ninety-seven is PRIME? Razzi had so much fun practicing with you today! See you next time!
Prime and Composite Numbers — Let's Practice! exercise
-
What are Prime and Composite numbers?
HintsUse the examples below to help you answer the questions.
9 is a composite number. Its factors are 1, 3, and 9: 1 x 9 3 x 3
3 is a prime number. Its factors are 1 and 3: 1 x 3
Make a list with all the factors of 12 and 7.
The prime number will have fewer factors than the composite number.
Solution- A Prime number has only two factors - 1 and itself.
- A Composite number has more than two factors.
- 12 is a composite number.
- 12 is composite because it has more than 2 factors: 1, 2, 3, 4, 6, and 12.
- 7 is prime because it has only 2 factors: 1 and 7 (itself).
-
Say whether 45 is prime or composite by listing all factors.
HintsLet's do one together.
3 is a factor of 45. How many times does 3 go into 45?
3 goes into 45 15 times. So, 15 is a factor of 45!
Remember, a number is prime when it has only 2 factors: 1 and itself.
So, how many factors did you find for 45?
SolutionThe factor pairs of 45 are: 1 x 45, 3 x 15, and 5 x 9.
45 is composite.
-
Answer the questions and fill in the missing factors.
HintsLet's complete the factor pair 3 x ? = 99.
First, find out how many times 3 goes into 99.
3 goes into 99 33 times. So, 33 is the missing factor in the pair!
Can you find the other factors?
A prime number has only two factors: 1 and itself.
A composite number has more than two factors.
Apply these rules to the factors you found for 83, 99, and 63.
Solution83: There are 2 factors, 1 and 83.
99: The factor pairs of 99 are: 1 x 99, 3 x 33, and 9 x 11.
63: The factor pairs of 63 are: 1 x 63, 3 x 21, and 7 x 9.
The prime number in the image is: 83.
The composite numbers in the image are: 99 and 63.
-
Help Razzi find all the factors for the numbers below.
HintsStart by finding all the factor pairs of 65. Can you fill in the missing factors?
1 x ?
5 x ?
Let's list the factor pairs of 52. Can you find the missing factors?
1 x 52
? x 26
4 x ?
Solution65 The factors of 65 are: 1, 5, 13, 65
67 The factors are: 1 and 67
52 The factors are: 1, 2, 4, 13, 26, 52
The prime number is 67. The composite numbers are 52 and 65.
-
Answer the questions about the numbers 17 and 16.
HintsLet's find the missing factor in the pair 2 x ? = 16.
How many times does 2 go into 16? Count up by twos until you reach 16.
2 goes into 16 8 times. So, 8 is the missing factor!
Remember:
You can check if a number is prime or composite by how many factors it has.
If it has more factors than 1 and itself, it's composite!
SolutionThe factor pairs of 17 are: 1 x 17.
17 is prime.
The factor pairs of 16 are:
1 x 16
2 x 8
4 x 4
16 is composite.
-
What are the prime numbers between 30 and 40?
HintsCheck the factors of each number to see which only have factors of 1 and the number itself.
Let's find the factors of 32 together.
The factor pairs of 32 are: 1 x 32, 2 x 16, 4 x 8. There are six factors.
So, 32 cannot be prime because it has more factors than just 1 and itself.
Remember, if a number has more than 2 factors it must be composite!
The even numbers between 30 and 40 cannot be prime because they have 2 as a factor!
SolutionThe prime numbers between 30 and 40 are: 31 and 37.










