Inequality Symbols: <, >, ≤, ≥

Basics on the topic Inequality Symbols: <, >, ≤, ≥
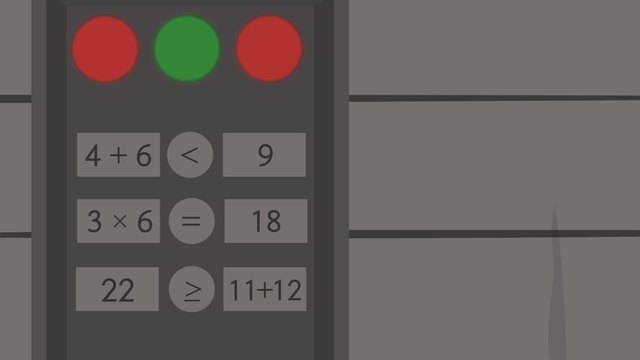
Inequality symbols are a shorthand notation used to compare different quantities. There are four inequality symbols “greater than”, “less than”, “greater than or equal to”, and “less than or equal to”. So, for instance, the sentence “5 is greater than 2” can be written as 5>2. A good way to remember which number is greater is to think of each symbol like a mouth; the mouth will always eat the larger of the two numbers being compared. Learn about inequality symbols by helping Christopher the vampire pack the maximum number of supplies he needed for his trip to California. Common Core Reference: CCSS.MATH.CONTENT.6.EE.B.8
Transcript Inequality Symbols: <, >, ≤, ≥
Christopher the Vampire is a foodie and he needs a fresh, new story for his blog: The Vegetarian Vampire. He’s working on a new piece, so he wants to go to a place where his favorite fruit grows: the blood orange. He read on Vampedia that blood oranges grow in California, which is perfect because he’s always wanted to visit the underground gardens there. To help him pack, he uses his knowledge of inequality symbols. And he has all his supplies laid out in his bed? Capes, check. Hair gel, check. Blood orange juice, check. But how much of this stuff is he allowed to carry with him on the plane? Let's take a look at the number line.
Use of inequalities
Christopher the Vampire’s trip will last fewer than 15 days. For inequailties with 'less than', we use this sign <. Furthermore, for this trip, Chris can't take more than 1000ml of blood orange juice on the plane. For inequalities like 'less than or equal to' we use this symbol: ≤. Our foodie vampire also needs to pack more than 1 bottle of hair gel, since he ran out during his last vacation. Let's draw this on the number line. For inequalities with 'more than', we use the 'greater than' symbol. He also needs to pack at least 16 capes, one for each day and two, just in case. For inequalities with 'at least', we use the 'greater than or equal to' symbol.
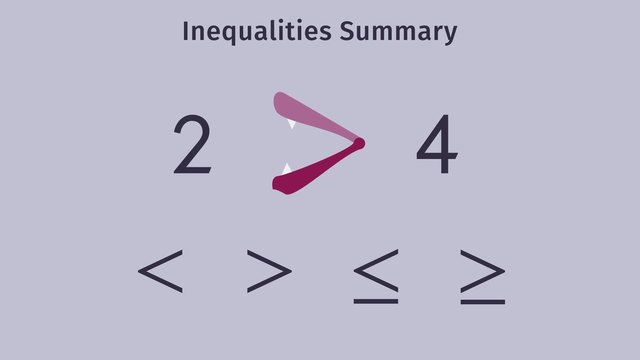
Inequalities summary - Imagine a mouth
Let's take another look at the different inequality symbols. A good way to remember which number is greater, is to think of each sign like a mouth. The mouth will always eat the larger of the two numbers being compared. For example let's compare 2 and 4. Since 2 is less than 4, the mouth will eat the 4. If the mouth opens to the right, it's read: 'a' is less than 'b'. However, if the mouth opens to the left, it's read: 'a' is greater than 'b'. As we saw earlier, the greater than and less than symbols can also be combined with the equal sign. When we say 'as many as' or 'no more than', we mean 'less than or equal to' which means that a could be less than b or equal to b. But, when we say 'at least', we mean 'greater than or equal to'. Here a could be greater than b or equal to b. Let’s see how Christopher the Vampire is enjoying his vacation. OH NO! No more blood oranges?!? This might make his vacation a bit tougher...
Inequality Symbols: <, >, ≤, ≥ exercise
-
Explain the inequality symbols.
HintsHere you see a number line for $>65$.
$\ge~$ is the same as $~>~$ including the $~=~$ relation.
Here you see a number line for $\le 55$.
Pay attention to the circle.
SolutionTo distinguish the inequality symbols:
- $<~$ for the less than relation. You see the regarding number line beside. The fact, that Christopher spends less than 15 days on his trip, is represented by an empty circle.
- $\le$ for the less than or equal relation. The difference between this symbol and the $<$-symbol is the $=$ sign. This can be seen by the filled circle.
- $>$ for the greater than relation. Similar to the $<$ relation the corresponding circle is empty.
- $\ge$ for the greater than or equal relation. It also includes the $=$ sign which can be shown by a filled circle.
-
Identify the symbol that correctly describes the relationship.
HintsTake a look at this example:
$4$ is more than $2$.
You can write it as $4>2$.
Or you could write it as $2<4$.
At least indicates greater than or equal.
Remember the relation signs:
- $<$ less than
- $\le$ less than or equal
- $>$ greater than
- $\ge$ greater than or equal
SolutionChristopher already knows that his trip takes fewer than 15 days. Fewer than indicates the $<$- or less than-symbol: $<15$. For the representation on a number line you use an empty circle surrounding 15.
The amount of blood orange he can take with is limited to the top by $1000~ml$, including this amount. This indicates the $\le$- or less than or equal-symbol: $\le 1000$. Here you use a filled circle.
Christopher knows how much hair gel he needs. So he concludes to pack more than one bottle of hair gel. This indicates the $>$- or greater than-symbol: $>1$. Again you use an empty circle.
Last but least he packs some capes: at least one for each day and one in spare, 16 in total. At least indicates the $\ge$- or greater than or equal-symbol: $\ge 16$. Here you use a filled circle on a number line.
But, what's that? Arriving in the blood orange garden Christopher discovers a sign: Sorry! No blood oranges.
-
Determine the corresponding mathematical inequality to match the number line.
HintsThis number line represents the inequality $x\le 7$.
- The arrow to the left indicates $<$ or $\le$.
- The filled circle stands for less than or equal.
This number line stands for $x>-20$.
The $\ge$-symbol can be excluded because the empty circle.
SolutionFirst, we consider number lines in general.
- An arrow to the left indicates a $<$ or $\le$ relation.
- An arrow to the right indicates a $>$ or $\ge$ relation.
- An empty circle means $<$ or $>$ depending on the direction of the arrow.
- A filled circle means $\le$ or $\ge$.
- $x\le 8$
- $x<8$
- $x>4$
- $x\ge 4$
-
Examine the inequality according to different word problems.
HintsAt least indicates greater than or equal.
More than indicates the $>$-symbol.
Distinguish between less than ($<$) and less than or equal ($\le$).
SolutionYou can memorize different keywords which indicate the regarding inequality-symbol:
- More than indicates greater than $>$.
- At least indicates greater than or equal $\ge$.
Birthday party You like to invite less than 10 friends. So we get $x<10$.
Earphones More than indicates $>$-symbol. This gives us $x>25$.
-
Decide which inequality symbol to use.
HintsPay attention
- $5<7$ but
- $-5>-7$
Take care of the use of $>$ or $\ge$:
- $7\ge 7$ but $7\not > 7$
- $7>4$ as well as $7\ge 4$
SolutionYou can imagine the greater than symbol as a mouth.
The bigger number eats the larger one.
- For example $4>2$. You can also use the $\ge$ sign.
- Similarly $2<4$ as well as $2\le 4$. The order is changed.
- $-2>-4$ as well as $-2\ge -4$.
- The other way round we can conclude $-4<-2$ or $-4\le -2$.
-
Determine the corresponding inequality.
HintsPay attention to the circle:
- Empty circles indicate $>$ or $<$.
- Filled circles indicate $\ge$ or $\le$.
A circle indicates the number you have to use in the inequality.
The circle indicates at the one hand the number $65$ and on the other that $65$ belongs to the inequality.
The arrow to the right indicates $>$ or $\ge$.
Together we can conclude the following inequality for this number line:
$x\ge 65$.
SolutionYou use number lines to represent inequalities.
First, you draw a circle exactly at the position of the regarding number.
Depending on the inequality symbol, the circle is filled or empty:
- Empty: $>$ or $<$
- Filled: $\ge$ or $\le$
Here you see four different number lines, top down:
- $x>-6$
- $x\le -2$
- $x<4$
- $x\ge 2$