Clever Calculations with Money
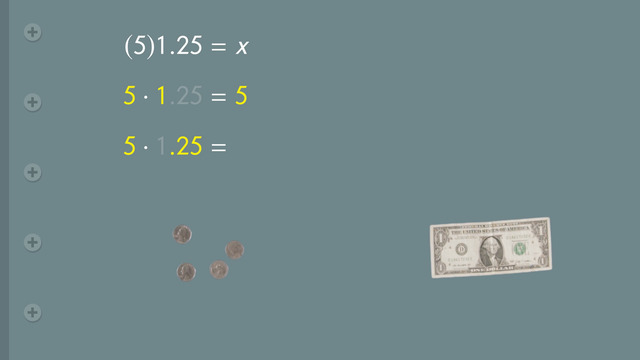

Basics on the topic Clever Calculations with Money
After this lesson, you will be able to solve real-world currency-related problems that require fast calculations.
The lesson begins by teaching you to note if certain amounts are linked to specific money values. It leads you to learn how to group the given amounts by place or money value. It concludes with a firm reminder to use the grouping method that makes the most sense to you.
Learn about clever money calculations by watching Denise outsmart Blake in computing their restaurant bill.
This video introduces new concepts, notation, and vocabulary such as the grouping of place and money values, like cents and dollars, quarters and dimes, even tens and hundreds, as a proper application of the mathematical properties of the basic operations required to solve the problem.
Before watching this video, you should already be familiar with currency values and terms, the four basic operations and the mathematical properties.
After watching this video, you will be prepared to better solve other real world problems that involve units of measurement, aside from currency, like time, distance and volume.
Common Core Standard(s) in focus: 4.MD.A.2 A video intended for math students in the 4th grade Recommended for students who are 9 - 10 years old
Transcript Clever Calculations with Money
Two friends; Blake and Denise go out to eat at the Disaster Zone. But it's no ordinary restaurant...Customers at the Disaster Zone can experience natural disasters while they eat, just like in nature! The two friends have finished eating and are now just waiting for their bill. Oh, their bill's all wet and some of the numbers are smeared. This is perfect! Blake's been dying to try out his new calculator app, but Denise believes she can calculate the missing information quicker than Blake and his app. In order to help Denise with her calculations, she needs to know how to do clever calculations with money. Let's take a look at their bill. Together, Blake and Denise ordered 5 drinks. Each drink costs $1.25. What is the total cost for the drinks? We can write this as a math expression by setting up an equation to look like this. If you see a question like this on your homework, you might be tempted to reach for your calculator, just like Blake. But, if we think of this multiplication problem in terms of money, we can imagine what the answer will be. Five times one is five. That leaves us with 5 times 0.25. What do you know that has the value 0.25? If you said a quarter, you're exactly right! This problem just got a whole lot easier! If you have 4 quarters, you have a dollar, and five quarters gives you $1.25. We just add this to 5 and we have our answer! $6.25. After multiplying the menu prices by how many of each menu item was ordered, the two friends decide to tally the total. Uh-oh! EARTHQUAKE! Good luck hitting those buttons, Blake! Getting back to the bill, they ordered $6.25 in drinks, a total of $13.85 in appetizers, and $26.10 for the main courses, and don't forget their coupon for $1! If we write a mathematical expression for their bill, we get 6.25 plus 13.85 plus 26.10 minus 1. First, let's look at the cents. We can group the 2 and 8, giving us one dollar. Since our coupon is worth $1, we can ignore this part. That leaves us with 5 cents plus 5 cents plus 10 cents. Five plus five is 10...10 plus 10 is 20 cents. Let's write this down. Next, we have to look at the dollars. Now we just have to add 6, 13, and 26. 6 and 13 is 19. Now we have 19 plus 26. Taking away 1 from 26 and giving it to 19 gives us 20 plus 25 which is 45, and adding our 0.20 from earlier leaves us with a total of 45.20. Group the numbers in the way that makes the most sense to you! Since Blake and Denise want to split the bill evenly, we have to divide by 2. Let's break this up again into dollars and cents. Just looking at the dollars, let's break up 45 into 40 and 5. 40 divided by 2 is 20 and 5 divided by 2 is 2.50, so 45 divided by 2 is 22.50. 20 cents divided by 2 is 10, so adding that to our previous answer, we get 22.60 each. Remember, group the numbers in the way that makes the most sense to you! Getting back to the Disaster Zone, Denise is finished with her calculations, but what about Blake? I guess you can't always rely on technology!
Clever Calculations with Money exercise
-
Explain how to use mental math using quarters.
HintsIf one drinks costs $ \$ 1.25$ dollars, five drinks costs
$1.25+1.25+1.25+1.25+1.25$.
You can do this more quickly by using multiplication.
One quarter is worth $0.25$ dollars.
SolutionWhat a disaster: Blake and Denise can't recognize the total for their five drinks, which cost $ \$ 1.25 $ each.
First, the keyword each indicates multiplication. So they have to multiply $5\times 1.25$.
For this they first multiply $5\times 1=5$. That's quite easy.
They still have to multiply $5\times 0.25$. Blake remembers that four quarters are in one dollar. So, there is still one left. This leads to
$5\times 0.25=1.25$.
Finally, they add the results $5+1.25=6.25$ to get the total for all their drinks: $6.25$ dollars.
-
Explain grouping mental math strategies for addition and subtraction.
HintsHere you see another example:
$22+19=21+1+19=21+20=41$.
A similar trick also works for subtraction:
$16-8=16+2-2-8=18-10=8$.
SolutionMental math is sometimes made easier with the help of a few tricks.
Simplifying addition with compensating
You adjust one number to have zero in the ones place by adding, and then subtract the same amount from the second number:
- $19+26=19+1-1+26=20+25=45$.
- $99+172=99+1-1+172=100+171=271$.
- $15-9=15+1-1-9=16-10=6$.
-
Explain how to group division problems.
HintsDivide step by step. Just look at the example $13.20 \div 2$:
- $10\div 2= 5$
- $3\div 2=0.75$
- $0.20\div 2=0.10$
Sometimes dividing leads to a remainder:
$10\div 3= 3$ with the remainder $1$
because
$3\times 3+1=10$
SolutionSometimes it's easier to divide step by step, and to add the individual results after:
$\mathbf{45.20\div 2}$
- $40\div2=20$
- $5\div 2=2.50$
- $0.20\div 2=0.10$
The next example shows how to handle a remainder:
$\mathbf{37.50\div 3}$
- $30\div 3=10$
- $7\div 3=2$ with a remainder of $1$
- Add the remainder to the decimal and divide the sum by $3$: $1.50\div 3=0.50$
$\mathbf{22.80\div 4}$
- $20\div 4=5$
- $2\div 4=0.50$
- $0.80\div 4=0.20$
-
Solve the following problems involving money.
HintsOne quarter is $25$ cents, a dime is $10$ cents, and a nickel is $5$ cents.
First decide if you have to add, subtract, multiply, or divide.
SolutionCameron
$0.25$ for each of the $8$ hats leads to multiplication: $8\times 0.25$. As $8$ is double $4$, it's easier to multiply a quarter by $4$ to get $1$ dollar, and to double the result to get $2$ dollars.
$~$
Sandy
Just add the values: $6+2\times 0.25+5\times 0.10+8\times 0.05=6+0.50+0.50+0.40=6+1+0.40=7.40$ dollars.
$~$
Anne
$5$ tacos at $3.40$ dollars each costs $5\times 3.40$:
- $5\times 3=15$
- $5\times 0.40=2$
$~$
Sakamma
Here we have to divide: We know the total $33.50$ for $5$ burgers.
- $30\div 5=6$
- $3\div 5=0.60$
- $0.50\div 5=0.10$
-
Determine the amount of money needed to pay each bill.
HintsCount the number of the notes as well as the coins.
Multiply the value of any bill or coin by the number of coins or bills of that type. Then add the resulting values.
SolutionLet's check the receipts from left to the right:
The receipt for $45.20$ dollars
Two $20$ dollar bills and one $5$ dollar bill, plus two $10$ cent coins.
The receipt with the amount $25.20$ dollars
Two $10$ dollar bills and one $5$ dollar bill, plus one $50$ cent piece and three $10$ cent coins.
The receipt with the amount $38.50$ dollars
One $20$ dollar bill, one $10$ dollar bill, one $5$ dollar bill, and three $1$ dollar bills, as well as one $50$ cent coin.
The receipt with the amount $27.60$ dollars
Two $10$ dollar bills, one $5$ dollar bill, and two $1$ dollar bill, plus one $50$ cent piece and one $10$ cent coin.
-
Identify correct mental math techniques.
HintsJust look at the following example $57\times 11$:
- The last digit is $7$.
- Add $5+7=12$.
- The middle digit is $2$.
- The first digit is $5+1=6$.
Check the statements with a few examples.
SolutionLet's check the tricks:
Multiplying a whole number by $10$
This leads to the same number with a $0$ tacked to the end:
- $12\times 10=120$
- $123\times 10 =1230$
- ...
- $1.2\times 10=12.0=12$
- $1.23\times 10=12.3$
Multiplying by $9$
$x\times 9=x\times (10-1)=10x-x$
using the distributive property. Let's have a look at an example:
$27\times 9=270-27=270-20-7=250-7=243$
Another example for simplifying multiplying by $5$. As $5=10\div 2$ we can do the following:
$x\times 5=x\div 2\times 10=x\times 10\div2$
Again, we try an example: $120\times 5=60\times 10=600$
You can always decide which calculation seems to be more comfortable for you.
$~$
Multiplying by $11$
$49\times 11$:
- The last digit is $9$.
- Add $4+9=13$.
- The middle digit is $3$.
- The first digit is $4+1=5$.
Here you see the explanation:
$49\times 11=49\times 10+49\times 1=490+49=539$
$~$
Squaring any number ending in $5$
You still have to use FOIL multiplication for two binomials:
$\begin{array}{rcl} 65^2&=&(60+5)^2\\ &=&60^2+2\times 60\times 5+25\\ &=&6^2\times 100+6\times 100+25\\ &=&6\times100(6+1)+25\\ &=&6\times 7\times 100+25\\ &=&4200+25=4225 \end{array}$