Linear and Nonlinear Functions

Basics on the topic Linear and Nonlinear Functions
A linear function is a function with standard form y = mx + b, where m is the slope and b is the y-intercept, and whose graph looks like a straight line.
There are other functions whose graph is not a straight line. These functions are known as nonlinear functions and they come in many different forms.
One such nonlinear function is a quadratic function, which is written in the standard form, y = ax2 + bx + c.
The graph of a quadratic function is a parabola. The direction in which the parabola opens depends on the coefficient a: If a is positive, the parabola opens upward. If a is negative, the parabola thus opens downward.
Another nonlinear function is the cubic function, which is written in the standard form, y = ax3 + bx2 + cx + d.
The graph of a cubic function look like a sideways S, where the direction S is rotated depends on a: If a is positive, S is rotated counter-clockwise. If a is negative, S is rotated clockwise.
Once you know the standard form of a function, it becomes much easier to graph and understand!
Analyze functions using different representations.
CCSS.MATH.CONTENT.HSF.IF.C.7
Transcript Linear and Nonlinear Functions
Welcome to the 65th annual Pole Position Championship! Where we have AIR TON from team Senna vs. Elaine from team Prost. Elaine seems really relaxed ahead of the final race of the season while AIR TON is carefully examining the track.
Linear and non-linear Functions
The race starts with a linear track where the racers can build up speed. Then, they have to transition to the quadratic portion of the track where they whip around Parabolica’s curve. The final leg of the race is the hardest. The cubic portion of the track requires the racers to navigate the tricky s-curve before they race to the checkered flag.
Linear Functions
To understand the track better, let's look at linear and non-linear functions. AIR TON’s team thinks it’d be a good idea if she has a different strategy for each portion of the race, so they analyze each part separately. The linear portion of the track is shown here.
As AIR TON knows, when linear functions are graphed, they always look like a straight line and are in the form f(x) = mx + b. Remember, ‘m’ is the slope and ‘b’ is the y-intercept. If ‘m’ is positive, the graph of the line increases from left to right. If ‘m’ is negative, the graph of the line decreases from left to right. So just remember, each one of these lines is also a linear function. The positive slopes generally look like this and the negative slopes generally look like this. This particular track has the equation f(x) = 4x + 22.
Quadratic Functions
The next part of the track AIR TON needs to analyze is Parabolica’s curve. Parabolica, that sounds like ‘parabola’. Quadratic functions, like the one shown here, either open down or open up. The functions are in the form f(x) = ax² + bx + c. If ‘a’ is positive, the graph opens upwards and downwards if ‘a’ is negative. Notice how the graph of quadratic functions have one ‘bend’ in the graph. This particular part of the track has the equation g(x) = -x² - 6x - 3. Notice how 'a' is negative and the graph opens downwards.
Cubic Functions
The last part of the track is Cubic Curve. Cubic functions have the form f(x) = ax³ + bx² + cx + d. If ‘a’ is positive, the graph of the line increases from left to right. If ‘a’ is negative, the graph of the line decreases from left to right. The graph of cubic functions have two ‘bends’ in the graph. So quadratic functions have one bend and cubic functions have two bends. The final curve has the expression h(x) = -1.5x³ + 3x² + 2x - 1
Let’s rejoin the racers at the starting line. Aaaaaand they’re off. well, one of them anyway.
Linear and Nonlinear Functions exercise
-
Identify the parameters of the given linear function.
HintsIn general, the slope intercept form of a linear function is given by $f(x)=mx+b$.
The term where $x$ as a factor is the slope.
The term where $x$ is not a factor is the $y$-intercept.
SolutionWe are analyzing the linear function $f(x)=4x+22$.
The graph of a linear function is a line. For our function $f(x)$ specifically, we can see that:
- $m=4$ is the slope.
- The graph of $f(x)$ is increasing; in general, a line is increasing when it has a positive slope, and decreasing when it has a negative slope.
- $b=22$ is the $y$-intercept.
-
Define a quadratic function.
HintsThe degree, or the largest exponent value of a term in an expression, of a quadratic function is $2$.
An example for a quadratic function is
$g(x)=-x^2-6x-3$.
The corresponding parabola is opened downwards, as the coefficient of $x^2$ is $-1$, which is negative.
SolutionThe second part of the race is a parabolic curve.
A parabola is the graph of a quadratic function. It looks like a rainbow, or a smile.
The standard form of a quadratic function is given by $f(x)=ax^2+bx+c$.
The degree, or the largest exponent value of a term in an expression, of a quadratic function is $2$.
The parameters $a$, $b$, and $c$ have different implications for the corresponding parabola. For example,
- If $a$ is positive, then the parabola is opened upwards.
- If $a$ is negative, then the parabola is opened downwards.
With this information, Air Ton can certainly prepare for the big race.
-
Decide which functions are linear functions.
HintsA linear function in slope intercept form is given by $f(x)=mx+b$.
You can also express a linear function in point slope form:
$f(x)-y_1=m(x-x_1)$,
where $(x_1,y_1)$ is any point on the line.
The degree, or the largest exponent value of a term in an expression, of a linear function is $1$.
An example of a linear function is $f(x)=2x+22$.
An example of a quadratic function is $f(x)=-x^2-6x-3$.
SolutionTo decide which function is a linear one, you have to look at the degree, or the largest exponent value of a term in an expression; it must be $1$:
- For $f_1(x)=\frac14+5x$, the highest exponent is $1$ and so this is a linear function with $m=5$ and $b=\frac14$.
- For $f_2(x)=\frac14+5x^2$, we can see that $2$ as the highest exponent. This is a quadratic function.
- For $f_3(x)=3(x-2)+5=3x-6+5=3x-1$, we have that $m=3$ and $y=-1$. This is a linear function as well.
- For $f_4(x)=\frac14x^2-5$, the highest exponent $2$ characterizes this function as a quadratic one.
- For $f_5(x)=\frac14x-5x^3$, this is a cubic function, with the highest exponent being $3$.
- For $f_6(x)=4x-5$, the highest exponent is $1$, with $m=4$ and $b=-5$. So this function is a linear one.
-
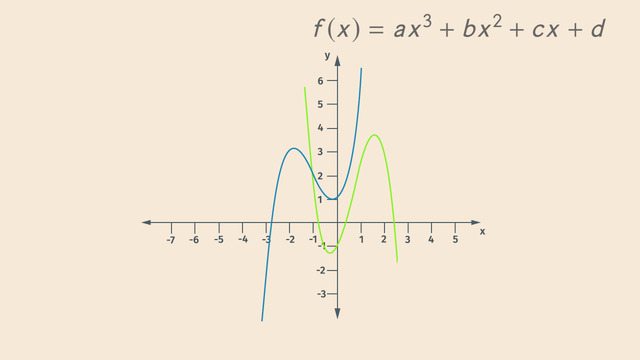
Determine the right function for the graph.
HintsAny cubic function is given by $f(x)=ax^3+bx^2+cx+d$.
The $y$-intercept of $f(x)=ax^3+bx^2+cx+d$ is $d$.
The $x$-intercepts are given by $x_1=-2$, $x_2=-1$, and $x_3=1$.
Check if the given functions have those $x$-intercepts.
SolutionTo establish the corresponding cubic function, first we remember the standard form of a cubic function:
$f(x)=ax^3+bx^2+cx+d$.
We can first see that $d=-1$ is the $y$-intercept of our cubic function.
We can also see that the $x$-intercepts are $x_1=-2$, $x_2=-1$, and $x_3=1$.
With $x_3=1$ we can conclude that $a(1)^3+b(1)^2+c(1)-1=0$, or equivalently that $a+b+c=1$.
$x_2=-1$ gives us similarly that $a(-1)^3+b(-1)^2+c(-1)-1=0$, or $-a+b-c=1$.
Adding these equations together, we get $2b=2$. Dividing by $2$ leads to $b=1$. Putting this value for $b$ in the equations above leads to
- $a+1+c=1$ or $a+c=0$.
- $-a+1-c=1$, which is equivalent to the equation above, $a+c=0$.
$a(-2)^3+(-2)^2+c(-2)-1=0$.
We can transform this equation into $-8a+4-2c-1=0$ and subtract $3$ to get $-8a-2c=-3$.
Lastly we substitute $a=-c$ from $a+c=0$ in the equation to get
$-8(-c)-2c=-3$.
Combining the like terms leads to $6c=-3$. Lastly we divide by $6$ to get $c=-\frac12$, and thus $a=\frac12$.
Together the resulting function is given by
$f(x)=\frac12x^3+x^2-\frac12x-1$.
-
Find the graphs of different types of functions.
HintsThe graph of a linear function, $f(x)=mx+b$, is a line.
The graph of a quadratic function, $f(x)=ax^2+bx+c$, is a parabola.
The graph of a cubic function has two bends, while the graph of a parabola has only one bend.
SolutionYou can see the solution in the picture to the right.
From the start of the race to the first orange marker point, we have a line. This is the graph of a linear function.
From the first orange marker point to the second, we have a parabolic curve, or a parabola. This is the graph of a quadratic function.
The last part of the race, from the second orange marker point to the finish is a cubic curve.
-
Find the graphs of the given functions.
HintsThe graph of a linear function, $f(x)=mx+b$, is a line.
You can see $b$, the $y$-intercept, immediately.
The graph of a quadratic function, $f(x)=ax^2+bx+c$, is a parabola.
A parabola is opened upwards (downwards) for $a>0$ ($a<0$).
Check several points lying on the graph.
SolutionThe graphs most left and right are parabolas. The corresponding functions are quadratic.
i.e. of the form $f(x)=ax^2+bx+c$.
The left one is opened downwards, so we have $a<0$, while the right one is opened upwards, and thus $a>0$. For both parabolas we see the $y$-intercept.
So the left parabola belongs to $f(x)=-x^2-4x-3$ and the right one to $h(x)=x^2-4x+1$.
The both inner graphs are lines and thus correspond to linear functions.
i.e. of the form $f(x)=mx+b$.
The left one is decreasing, so $m<0$, and has the $y$-intercept is $b=1$. So the corresponding function is $m(x)=-\frac12x+1$.
The right line is increasing, meaning that $a>0$, with $b=2$. The corresponding function is given by $n(x)=2x+2$.