Representing Proportional Relationships with Equations

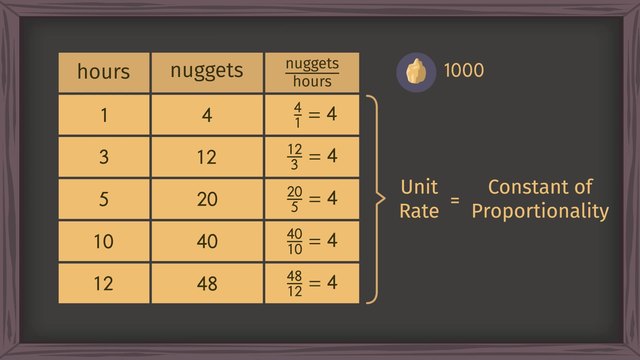
Peter Pan has flown into Wendy’s room! And has invited her to take off to Neverland with him! But Wendy is confused; how can she possibly fly like Peter Pan? Peter explains that there is a proportional relationship between happy thoughts and altitude. And if we represent this proportional relationship with an equation, we can help Wendy take to the skies! Peter explains that he has to think 2 happy thoughts in order to ascend 15 feet. It takes 4 happy thoughts for him to ascend 30 feet, and so on. Oh no! Peter realizes that a fog has set in, and they will need to think enough happy thoughts to fly above it and off to Neverland. They will need to fly above 400 feet to beat the fog, but 400 feet is not in Peter's table! If Peter's table represents a proportional relationship, then he can set up an equation to help him figure out how many happy thoughts he needs to get above the fog. Let's write the ratio of altitude to Peter’s happy thoughts in a new column in our table. We know this table represents a proportional relationship if the ratio of feet to thoughts is always the same. Simplifying each ratio we see that 30 over 4 reduces to 15 over 2 and that all the ratios reduce to this fraction, meaning that the table represents a proportional relationship. To set up an equation from the table, let’s first assign some variables. Let’s make happy thoughts 'h', and altitude 'a'. We can now see that 'a' over 'h' always equals 15 over 2 or 7 point 5. We know that 7 point 5 is our unit rate, or number of feet Peter flies per one happy thought. The unit rate is also known as the constant of proportionality. As, while the variables 'h' and 'a' can change, the constant of proportionality stays, well, constant! If we multiply both sides by 'h', we can see that for every happy thought Peter thinks his altitude goes up by a factor of 7 point 5. This is our equation for the proportional relationship in standard form. So using our equation, how many happy thoughts does he need to fly above the fog? Remember, the fog in the city extends up to 400 feet. So, plug in 400 for 'a.' To isolate the variable 'h', divide both sides by 7.5.

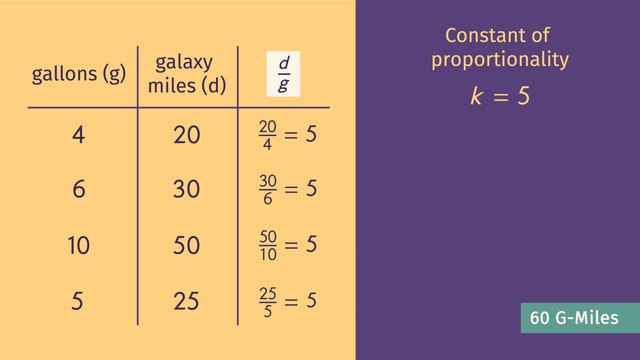
This gives us 'h' equals 53 and one-third happy thoughts. So Peter decides to think 54 happy thoughts to fly just over the fog. Wendy starts thinking happy thoughts and begins to fly, but how many happy thoughts does she need to get above the fog? Peter shows Wendy a graph of her altitude versus the number of her happy thoughts! Is Wendy’s altitude proportional to her happy thoughts? Yes! Because the graph is a straight line, going through the origin. 0 happy thoughts gets her an altitude of 0 and a straight line has a constant slope, which is our constant of proportionality. If we can find the constant of proportionality from the graph, we can make an equation! The constant of proportionality is given by the unit rate. The unit rate is the altitude Wendy achieves from one happy thought. At one happy thought we find that Wendy flys 12 feet! So Wendy's unit rate is 12 feet per one happy thought. The unit rate, or constant of proportionality, tells us that the ratio of altitude 'a' to thought 'h' will always equal 12. Multiplying both sides by 'h' we have our equation for Wendy in standard form! Just like Peter, Wendy needs to fly above 400 feet to get over the fog. We can plug in 400 for 'a' to figure out how many happy thoughts she will need. To isolate the variable 'h', divide both sides by 12 to get that Wendy needs 33-and-a-third happy thoughts to get above the fog. She decides to think of 34 happy thoughts to be in the clear. Peter and Wendy are super happy kids, so they quickly zoom above the fog, into the moonlight on their way to Neverland! But poor Nana the dog wants in on the magic! All right Nana, let's review what we've learned. Given a table representing a proportional relationship any ratio in the table can be used to find the constant of proportionality. This gives us an equation. Given a graph, we can find the constant of proportionality by finding the 'y'-coordinate on the graph corresponding to 'x' equals 1. As before, our constant of proportionality equals 'y' over 'x', or 'a' over 'h'. This gives us an equation representing our proportional relationship. Representing proportional relationships with equations enables us to quickly find any ratios we need to fly at any altitude! Nana musters up one very happy thought, and ascends 5 feet! Ah, now we know the happy thought that inspired her flight! Who needs Neverland when there are treats right here!