Powers of Products and Quotients
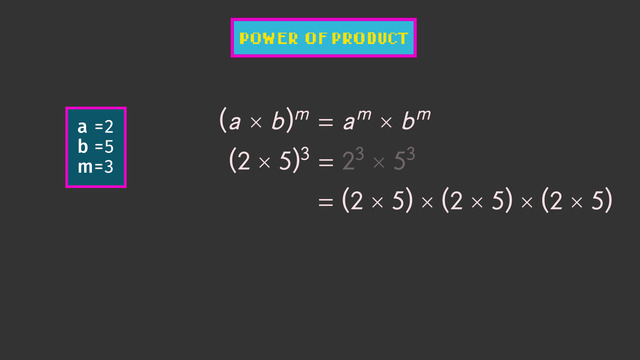

Basics on the topic Powers of Products and Quotients
The powers of products rule says that raising a product a∙b to the power m, is the same as raising a to the power m and b to the power m and then considering the product am ∙ bm:
(a ∙ b)m = am ∙ bm.
The powers of quotients rule says that raising a quotient a/b to the power m is the same as raising a to the power m and b to the power m and then considering the quotient am ÷ bm:
(a ÷ b)m = am ÷ bm.
Indeed we can see in the following example that the powers of products rules works: (3 ∙ 5)² = 3² ∙ 5² (15)² = 9 ∙ 25 225 = 225
And in the following example that the powers of quotients rule also works: (4 ÷ 2)³ = 4³ ÷ 2³ 2³ = 64 ÷ 8 8 = 8
The powers of products and the powers of quotients rules are powerful tools which make solving algebraic and arithmetic problems easier and faster, allowing us to calculate important quantities in our daily lives, like speed or distance of moving objects.
Work with radicals and integer exponents. CCSS.MATH.CONTENT.8.EE.A.1
Transcript Powers of Products and Quotients
Charlotte is playing a new game, Pow Pow Powers, but the kids call it 'Triple P'. She is stuck on a level at the moment in this level, she has to complete the rules for raising products and quotients to a power. Surely, her knowledge of Powers of Products and Quotients will help her dance her way to the top of the leaderboard.
Power of Products and Quotients Rules
Charlotte has to complete the rules for raising a product, a quotient, and a power to a power in order to achieve gaming immortality. She starts with the power of a product. The variables are coming faster now
"'a'! Times! 'b'! all to the 'm' power! Equals! 'a'! To the 'm' power! Times! 'b' to the 'm' power!"
Next up is raising a power to a power! The variables just keep coming!
"'a'! To the 'm' power! all to the 'p' power! Equals! 'a'! To the 'm'! Times 'p'! Power!"
Last one! Raising a quotient to a power! Can Charlotte keep up?!
"'a'! Divided by! All to the 'm' power! Equals! 'a'! To the 'm'! Divided by! 'b'! to the 'm'! Power!"
Let's look at an example of the rules Charlotte needed to know for the game with real numbers.
Raising Products to a Power
The first rule was for raising products to a power. [a(b)]ᵐ = aᵐ(bᵐ) Let's plug in 2 for 'a', 5 for 'b' and 3 for 'm'. Is [2(5)]³ the same as (2³)(5³)? Let's think of [2(5)]³ as (2)(5) (2)(5) (2)(5).
According to the Commutative Property of Multiplication, you can change the order of the factors to be (2)(2)(2)(5)(5)(5). To simplify this, you can write (2³)(5³). This means that [2(5)]³ is the same as (2³)(5³).
Raising a Power to a Power
But, how do you raise a power to a power? This rule states that (aᵐ)ᵖ = aᵐ⁽ᵖ⁾. Let's let 'a' be 2 again. 'm' is still 3. 'p' will be 4. Is (2³)⁴ the same as 2¹²? Let's imagine multiplying 2³ by itself four times. Since the bases are the same, we can add the exponents together. There are four exponents with the value 3, so we can write the expression as 2⁽³⁾⁽⁴⁾. To simplify this, we can multiply the exponents, giving us 2¹².
Raising Quotients to a Power
For her last feat, Charlotte raise quotients to a power. This rule states that (a/b)ᵐ = aᵐ / bᵐ. What does this look like when we use numbers? For this, 'a' will be 2 again, 'b' will be 5 and 'm' will be 3. Which brings us to our question: what is (2/5)³ equal to?
According to the Commutative Property of Multiplication, you can rewrite this expression as (2/5)(2/5)(2/5). For the numerator, we can write (2)(2)(2) and for the denominator, (5)(5)(5). Simplifying this gives us (2)³ / (5)³.
Let's see how Charlotte's getting along in the game. She did it! First place! A NEW #1 and bragging rights! But just as Charlotte goes to enter her name as the champion of the household...
Powers of Products and Quotients exercise
-
Determine the missing term.
HintsAn example using the power of products rule:
An example using the power of quotients rule:
If you raise a power to a power, you can write it as a product:
SolutionLet's start with the power of products rule:
$(a\times b)^m=a^m\times b^m$.
This means that you have to raise each factor to the same power.
To raise a power to a power, $(a^m)^p=a^{m\times p}$, keep the basis and multiply the exponents.
Let's look at the power of quotients rule:
$\left(\frac ab\right)^m=\frac{a^m}{b^m}$.
Here we raise the numerator as well as the denominator by the power.
-
Prove that $(2\times 5)^3 = 2^5\times 3^5$ using the power of products rule.
HintsA power is shorthand for a product:
Multiplication is commutative; i.e. $a\times b= b\times a$.
Remember the power of products rule:
SolutionLet's have a look at the power of products rule:
$(a\times b)^m=a^m\times b^m$.
Plugging in $a=2$, $b=5$, and $m=3$, we get that
$(2\times 5)^3=(2\times 5)\times(2\times 5)\times(2\times 5)$.
As multiplication is commutative, we can change the order of multiplication:
$(2\times 5)^3=2\times 2\times 2\times 5\times 5\times 5$.
We know that $2\times 2\times 2=2^3$ and that $5\times 5\times 5=5^3$, giving us $(2\times 5)^3=2^3\times 5^3$.
-
Complete the following examples.
HintsRemember the rules:
An example of the power of a power rule with $a=4$ and $m=p=2$:
SolutionUsing the rules,
$\begin{array}{lrcl} \text{product}&(a\times b)^m&=&a^m\times b^m\\ \text{power}&(a^m)^p&=&a^{m\times p}\\ \text{quotient}&\left(\frac ab\right)^m&=&\frac{a^{\large m}}{b^{\large m}} \end{array} $
we can compute the concrete examples Charlotte needs to solve in her game:
- $(4\times 3)^2=4^2\times 3^2$
- $(4^3)^2=4^{3\times 2}=4^6$
- $\left(\frac 43\right)^2=\frac{4^{\large 2}}{3^{\large 2}}$
- $(3\times 2)^4=3^4\times 2^4$
-
Determine which terms are equal.
HintsRemember the power of a product rule:
$(a\times b)^m=a^m\times b^m$.
An example of the power of a quotient rule:
To raise a power just multiply the exponents.
SolutionLet's have a look at some examples with constants as well as variables.
Using the power of a product rule, $(a\times b)^m=a^m\times b^m$, we have
- $50^x=(5\times 10)^x$
- $(2\times x)^2=2^2\times x^2=4x^2$
- $0.5^x=\left(\frac48\right)^x=\frac{4^{\large x}}{8^{\large x}}$
- $\frac{10^{\large 2}}{5^{\large 2}}=\left(\frac{10}5\right)^2=2^2=4$
- $(x^2)^3=x^{2\times 3}=x^6$
- $(2^3)^2=2^{3\times 2}=2^6$
-
Decide which example belongs to which rule.
HintsThink about the meaning of the variables in each rule.
Check if the rules are correct.
An example of the power of product rule with $a=3$, $b=10$, and $m=2$:
Solution$\begin{array}{lrcl} \text{product}&(a\times b)^m&=&a^m\times b^m\\ \text{power}&(a^m)^p&=&a^{m\times p}\\ \text{quotient}&\left(\frac ab\right)^m&=&\frac{a^{\large m}}{b^{\large m}} \end{array} $
Let's check each of the rules with an example.
The power of product rule with $a=2$, $b=5$ and $m=3$:
$\begin{array}{rclll} (2\times 5)^3&=&(2\times 5)\times(2\times 5)\times(2\times 5)&|&\text{commutative property}\\ &=&2\times 2\times 2\times 5\times 5\times 5&|&\text{definition of powers}\\ &=&2^3\times 5^3 \end{array}$
The power of power rule with $a=2$, $m=3$ and $p=4$:
$\begin{array}{rclll} (2^3)^4&=&(2^3)(2^3)(2^3)(2^3)&|&\text{add the exponents}\\ &=&2^{(3+3+3+3)}\\ &=&2^{12}=2^{3\times 4} \end{array}$
the power of quotient rule with $a=2$, $b=5$ and $m=3$:
$\begin{array}{rclll} \left(\frac25\right)^3&=&\left(\frac25\right)\left(\frac25\right)\left(\frac25\right)\\ &=&\frac{2\times 2 \times2}{5\times 5 \times 5}\\ &=&\frac{2^{\large 3}}{5^{\large 3}} \end{array}$
-
Rewrite each term using the power of a product, power, and quotient rules.
HintsTo raise a product just raise each factor to the same exponent.
To raise a quotient raise the numerator as well as the denominator to the same exponent.
To raise a power keep the basis and multiply the exponents.
SolutionWe have the following equalities:
- $\left(\frac23\right)^7=\frac{2^{\large 7}}{3^{\large 7}}$
- $(3^3)^3=3^{3\times 3}=3^9$
- $\left(\frac 56\right)^6=\frac{5^{\large 6}}{6^{\large 6}}$
- $(3x)^4=3^4\times x^4$
- $(xy)^5=x^5\times y^5$
- $(2^x)^2=2^{x\times 2}=2^{2x}$