Percent Error
Basics on the topic Percent Error
Percent Error
Percent error is a mathematical tool used to measure the accuracy of a value compared to its true or exact value. It's particularly useful in scientific experiments and everyday calculations where precision is important.
Understanding Percent Error – Explanation
To find the percent error, you need to understand three key terms: the actual value, estimated value, and percent error itself. The actual value is the exact number you're comparing to, while the estimated value is the prediction or measurement you've made. The formula to calculate percent error is:
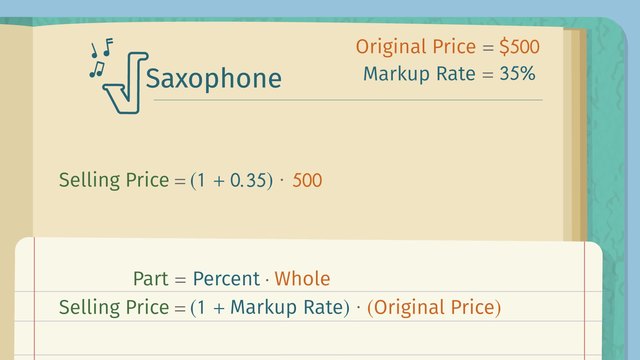
Percent Error $=|\dfrac{Actual-Estimated}{Actual}|\times 100$
This formula helps determine how far off an estimate is from the actual value in percentage terms.
| Part of Formula | Description |
|---|---|
| Actual | The true, exact, or correct value that serves as a reference to compare against. |
| Estimated | The measured or predicted value that is being compared to the actual value. |
| Actual - Estimated | The difference between the actual and estimated values, indicating the error in measurement. |
| I Actual - Estimated / Actual I | The absolute value of the difference divided by the actual value, representing the error relative to the actual value. |
| x 100 | Converts the relative error into a percentage, making it easier to interpret the magnitude of the error. |
Just like any formula, once the values are known they can be substituted for the corresponding variables to find the solution, in this case, the percent error.
Converting Decimals to Percents
To find the percent error you will need to remember how to convert a decimal to a percentage. The decimal can be multiplied by $100$ to find the percent. For example, to convert 0.65 to a percent, you would calculate $0.65 \times 100 = 65\%$. This method applies to any decimal and quickly transforms it into an easily understandable percentage. Additionally, you can explore more about these conversions in the section convert between percent, fractions and decimals.
Percent Error – Step-by-Step Process
Steps to Find the Percent Error:
- Identify the actual value.
- Identify the estimated value.
- Substitute these values into the percent error formula.
- Multiply the result by 100 to convert it into a percentage.
Example 1: Suppose a teacher estimates that their classroom is 15 meters long, but the actual length, as measured by a maintenance worker, is 14.8 meters.
- Identify the actual value: The actual length of the classroom is $14.8$ meters.
- Identify the estimated value: The estimated length by the teacher is $15$ meters.
- Substitute these values into the percent error formula: $\text{Percent Error} = \left| \frac{15 - 14.8}{14.8} \right|$
- Multiply the result by 100 to convert it into a percentage: $\left| \frac{15 - 14.8}{14.8} \right| \times 100 \approx 1.35\%$
The percent error in the teacher's estimate is approximately $1.35\%$.
Example 2: Sarah estimates that her grocery bill will be $\$$40 dollars, but the actual total comes to $\$$38.
- Identify the actual value: The actual cost of the groceries is $38$.
- Identify the estimated value: Sarah's estimated cost is $40$.
- Substitute these values into the percent error formula: $\text{Percent Error} = \left| \frac{40 - 38}{38} \right|$
- Multiply the result by 100 to convert it into a percentage: $\left| \frac{40 - 38}{38} \right| \times 100 \approx 5.26\%$
Sarah's estimation had a percent error of approximately $5.26\%$.
Use the percent error formula and try some on your own!
Percent Error – What Does This Number Mean?
Percent error isn't just about finding mistakes; it's a way to understand how precise or accurate your measurements are. This number tells you how much your estimate or measurement has deviated from the actual value in terms of percentage. A higher percent error means a less accurate measurement, while a lower percent error means your measurement is quite close to the true value. Let’s look at what these numbers really mean.
| Percent Error | Description |
|---|---|
| 0% | Perfect! Your measurement or estimate is exactly the same as the actual value. |
| Less than 5% | Excellent accuracy. This is often good enough for most applications, including science class. |
| 5% to 10% | Reasonable accuracy. There's some room for improvement, but it's acceptable for many situations. |
| Greater than 10% | Consider checking how you're measuring. This might be too inaccurate for reliable results, depending on what you're doing. |
Understanding this can help you judge the reliability of your results and see if you might need to change your method or tools.
Example 1: Measuring the height of a plant.
Person A's estimate: 22 cm
Person B's estimate: 24 cm
Actual value: 20 cm
Example 2: Predicting the score of a game.
Person A's estimate: 50 points
Person B's estimate: 55 points
Actual score: 48 points
Example 3: Estimating the number of pages in a book.
Person A's estimate: 300 pages
Person B's estimate: 285 pages
Actual number of pages: 290 pages
Percent Error – Real World Application
Understanding percent error is crucial because it shows us how accurate our measurements or guesses are compared to the real values. Here’s why that’s important:
- Checking Accuracy: It helps you see how close your science experiment measurements are to what they should be. This is like checking if you are hitting the target in archery.
- Improving Techniques: If you know your error is big, you can try different ways to measure more accurately next time. It’s like practicing a musical instrument to get better notes.
- Making Better Products: In making things like toys or gadgets, knowing the error helps make sure everything works properly and fits right.
- Better Decisions: In businesses, understanding errors in money estimates can help decide how much to spend or save.
- Enhancing Gaming: Consider playing a virtual reality (VR) game where you need to interact with objects in a virtual environment. If your VR headset doesn't track your movements accurately, it could ruin your experience. Calculating percent error helps improve these technologies, ensuring your actions in the virtual world feel real and responsive.
Percent Error – Summary
Key Learnings from this Text: * The percent error formula is a valuable tool for measuring the accuracy of measurements against actual values. * It involves three steps: finding the actual and estimated values, substituting them into the formula, and converting the result into a percentage. * A negative percent error indicates an underestimation. * This concept is useful in both academic settings and everyday life to evaluate and improve measurement techniques.
Percent Error – Frequently Asked Questions
Transcript Percent Error
At the tempered timber amusement park, Otter This World, Plunk 'N Dunk is the park's most popular attraction. Customers try to stop the needle within a 10% error of the exact value, 50 inches. If a customer is successful, dunk goes the weasel sittin' in the dunk tank. But, strange things have been happening at night. Lately, only a few customers have been able to stop the needle within the accepted percent error, so naturally, the weasels have been kicking back and relaxing in the dunk tank. Much to the chagrin of the weasels, Harriet, the park's meticulous manager, is investigating. In order to help Harriet figure out what went wrong, we'll need to understand percent error. To play Plunk 'N Dunk, customers try and stop a needle on the number 50, but if customers stop the needle within a 10 percent error of 50, or the "dunk zone", then dunk goes the weasel. Currently, the Dunk Zone has an exact value of 50 inches, and goes from the 47 inch mark on the left to the 52 inch mark on the right. To understand percent error, we first have to know about absolute error. Absolute error is the absolute value of the difference between the exact value and the measured value. In our case, the exact value is 50 inches and the measured value is 47 or 52 inches. Let's start with a measured value of 52 inches and find the absolute error. We first need to subtract the numbers, giving us the absolute value of 2. Since absolute value means to take the distance from zero on the number line, we're left with an absolute error of 2. Now we can find percent error with ease. Percent error is found by first dividing the absolute error by the absolute value of the exact value, then multiplying by 100 to get a percentage. We already know the absolute error, 2 inches, and we already know the exact value is 50 inches, so let's substitute that here. Dividing our absolute error by the absolute value of the exact value, 50, gives us 0.04. And when we multiply 0.04 by 100, we get a percent error of 4 percent! That's definitely not the 10% error that it should be. So let's look at the percent error to the 47 inch mark. We know that, to find percent error, we first need the absolute error divided by the absolute value of the exact value. Then, this is multiplied by 100, giving us our percent error. But how do we find absolute error, again? Remember, absolute error is how far our measured value is from the exact value we want. Therefore, we subtract the two numbers and take their absolute value because we want their distance apart and that's always positive. Now, we just need to substitute and solve! This time, our measured value is 47 inches and our exact value is still 50 inches. Subtracting 50 from 47 gives us negative 3. The absolute value of negative 3 is 3. That is our absolute error. Percent error takes it a bit further and shows us the error as a percentage of the exact value. Using substitution, we can take the absolute error, 3, and divide it by the absolute value of our exact value, 50, before multiplying by 100. This leaves us with 0.06, which, when multiplied by 100, gives us our percent error of 6%. That's also not the 10% that we want. Before contacting Harriet, let's review what we've learned. Absolute error is found by subtracting the exact value from the measured value and then taking the absolute value of the result. While percent error is found by dividing the absolute error by the absolute value of the exact value, then multiplying by 100 to get a percentage, percent error tells you how close a measured value is in relation to the exact value. In this example, the weasels decreased the allowed percentage error, which made it more difficult for the customers to stop the needle in the Dunk Zone. Now that we've helped Harriet understand percent error, she's come down to inspect the faulty attraction. She notices someone has changed the numbers on the box! Those weasels, they think they're so slick. Harriet's got just the surprise for them! There, that should fix it!
Percent Error exercise
-
Which formula shows the correct percent error equation?
HintsRemember, you are trying to find the percent error.
You must divide the absolute error by the exact value.
SolutionTo find the Percent Error, you must divide the Absolute Error by the Exact Value, so the correct formula is:
Percent Error = ${\frac{\text{Absolute Error}}{|\text{Exact Value}|}}$
-
What are the steps for finding the percent error of 247 from the exact value of 250?
HintsRemember, it is always a good idea to start by setting up the formula you are working with.
You will need to find the Absolute Error of 247 from 250 before you can solve the equation.
Converting the decimal to a percent is one of the last steps to finding Percent Error.
SolutionSet up the formula: Percent Error = ${\frac{\text{Absolute Error}}{|\text{Exact Value}|}}$.
Find the absolute error of 247 from 250. The absolute error is 3.
Enter the Absolute Error and Exact Values into the formula: Percent Error = ${\frac{\text{3}}{\text{250}}}$.
Now solve for Percent Error: $\bf{\frac{3}{250}} = 0.012$.
To convert a decimal to a percent, multiply it by one hundred: $0.012\cdot100 = 1.2$.
The Percent Error of 247 from 250 is 1.2%
-
Using 100 as the Exact Value, pair up the Estimated Values with the correct Percent Error.
HintsThe Exact Value in the formula will always be 100.
Remember, the Absolute Error is the estimates values distance from the Exact Value on a number line.
Once you have found the Absolute Error value, multiply it by 100 to get the Percent Error.
Let's look at the number 90. It is 10 from the exact value of 100, so rewrite the formula:
Percent Error = $\bf{\frac{\text{10}}{|\text{100}|}}$
Then solve by dividing 10 by 100, which is 0.1.
Percent Error = 0.1
Now multiply this by 100 to get the percent error of 10%.
The percent error of 90 from 100 is 10%.
Solution100 = 0%
Percent Error = $\frac{0}{100}$
Percent Error = 0
Percent Error = $0\cdot100 = 0$
Percent Error = 0%
90 = 10%
Percent Error = $\frac{10}{100}$
Percent Error = 0.1
Percent Error = $0.1\cdot100 = 10$
Percent Error = 10%
0 = 100%
Percent Error = $\frac{100}{100}$
Percent Error = 1
Percent Error = $1\cdot100 = 100$
Percent Error = 100%
120 = 20%
Percent Error = $\frac{20}{100}$
Percent Error = 0.2
Percent Error = $0.2\cdot100 = 20$
Percent Error = 20%
-
Can you find Divya's percent error?
HintsPercent Error is what we are trying to find.
To find absolute error, find the distance between $\$28$ and $\$35$ on a number line.
To begin, you would start by dividing 7 by 28, since 7 is the absolute error, and 28 is the total value.
Percent Error = $\frac{\text{7}}{\text{28}}$
SolutionPercent Error = $\frac{\text{Absolute Error}}{|\text{Exact Value}|}$
The absolute error of __USD__35 from __USD__28 is __USD__7
Percent Error = $\bf{\frac{7}{28}}$
$\frac{7}{28}$ = 0.25
$0.25\cdot100 =$ 25
Percent Error = 25%
-
Solve the problem using the formula: $\bf{Percent Error =}$ $\bf{\frac{\text{Absolute Error}}{|\text{Exact Value|}}}$
HintsYou must first find the Absolute Error, which is the distance the estimate is from the Exact Value. Here, it is 5.
Divide the Absolute Error by the Exact Value. Here, you would divide 5 by 50.
You will need to convert the decimal to a percent by multiplying it by 100 to find the Percent Error.
SolutionTo find the Absolute Error, find the distance of __USD__55 from the exact value of __USD__50.
Absolute Error = 5
Percent Error = $\frac{5}{50}$
Solve for Percent Error: $\frac{5}{50}$ = 0.1
Convert 0.1 to a percent
$0.1\cdot 100 = 10$
Percent Error = 10%
-
Find the percent error of the given values from the exact value of 75.
HintsUse the formula Percent Error = $\bf{\frac{\text{Absolute Error}}{|\text{Exact Value}|}}$ to solve the percent error of each value from 75.
The |Exact Value| in this problem will always be 75.
The absolute error in the formula is the distance of the value from 75 on a number line.
For example, the absolute error of 82 would be 7.
Percent Error = $\bf{\frac{\text{7}}{|\text{82}|}}$
Percent Error = 0.085
To find the Percent Error, multiply the percent error value by 100.
Percent Error = 0.085 $\cdot$ 100 = 8.5%.
82 has an absolute error of less than 10%.
SolutionPercent Error of 82 = 9.33%
Percent Error of 68 = 9.33%
Percent Error of 60 = 20%
Percent Error of 86 = 14.67%